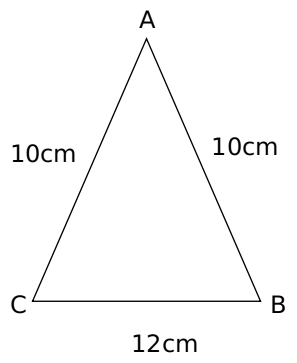
The sides of an isosceles triangles are 10 cm, 10 cm and 12 cm. What is the area of the triangle?

Solution

To find the area of the isosceles triangle ABC draw a perpendicular line from A to the base of the triangle BC and name that point as D such that it will become a right angled triangle.

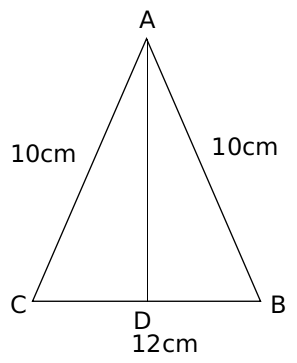
Now,BD = CD =6cm.The area of the right angled triangle is given as = $$\frac{1}{2}\times{b}\times{h}$$,where b and h are base and height.
 Height we can calculate from pythagoras theorem
 $$AD^{2}$$ = $$(AB^{2} - BD^{2})$$
$$\Rightarrow$$ $$AD^{2}$$ = $$(10^{2} - 6^{2})$$
$$\Rightarrow$$ AD = 8cm.
The area of right angled now will be = $$\frac{1}{2}\times{6}\times{8}$$ = 24$$cm^{2}$$
The area of triangle ABC will be two times the area of triangle ADB = 24+24=48$$cm^{2}$$.
Hence option B is correct.