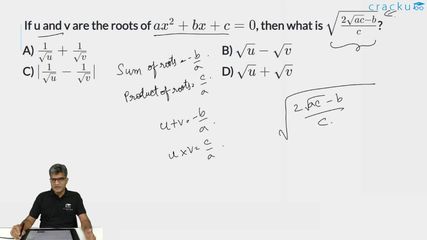
If u and v are the roots of $$ax^{2} + bx + c = 0$$, then what is $$\sqrt{\frac{2\sqrt{ac}-b}{c}}$$?

Using the sum and product of roots formula, we get $$\frac{c}{a}=uv$$, and hence, $$c=auv$$
And $$-\frac{b}{a}=u+v$$, and hence, $$-b=a\left(u+v\right)$$

Putting values of c and b in the equation we get, 
$$\sqrt{\ \frac{2\sqrt{\ ac}-b}{c}}=\sqrt{\ \frac{2\sqrt{\ a\left(auv\right)}+a\left(u+v\right)}{auv}}$$
=$$\sqrt{\ \frac{2\sqrt{\ a^2uv}+a\left(u+v\right)}{uav}}=\sqrt{\ \frac{2\sqrt{\ uv}+\left(u+v\right)}{uv}}$$
=$$\sqrt{\ \frac{\left(\sqrt{\ u}+\sqrt{\ v}\right)^2}{uv}}=\sqrt{\ \frac{\left(\sqrt{\ u}+\sqrt{\ v}\right)^2}{\sqrt{\ uv}^2}}=\sqrt{\ \left(\frac{\sqrt{\ u}+\sqrt{\ v}}{\sqrt{\ uv}}\right)^2}$$
=$$\frac{1}{\sqrt{\ u}}+\frac{1}{\sqrt{\ v}}$$

Therefore, Option A is the correct answer.