There are 150 residents in a society. Out of them, 50 residents own a motorcycle, 60 residents own a car and 20 residents own both a car and a motorcycle. How many residents neither own a motorcycle nor a car?

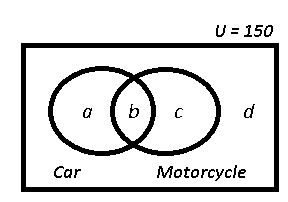
Total number of residents in the society = $$a+b+c+d=150$$ ---------------(i)

Residents who own motorcycle = $$b+c=50$$ ----------------(ii)

Residents who own car = $$a+b=60$$ --------------(iii)

Also, Residents who own both car and motorcycle = $$b=20$$

Substituting above values in equations (ii) and (iii), => $$c=50-20=30$$ and $$a=60-20=40$$

$$\therefore$$ From equation (i), we get : $$40+20+30+d=150$$

=> $$d=150-90=60$$

Thus, 60 residents neither own a motorcycle nor a car.

=> Ans - (C)