Question 25

In ΔUVW measure of angle V is 90 degrees. If sinU = 24/25, and UV = 0.7cm, then what is the length (in cm) of side VW?

Solution

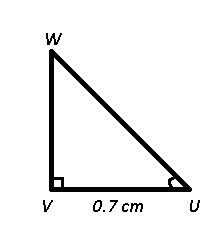
Given : UV = 0.7 cm and sin U = $$\frac{24}{25}$$ ------------(i)

To find : VW = ?

Solution : $$cosU=\sqrt{1-sin^2U}$$

=> $$cosU=\sqrt{1-\frac{576}{625}}=\sqrt{\frac{49}{625}}=\frac{7}{25}$$ ----------(ii)

Dividing equation (i) by (ii), => $$tanU=\frac{24}{7}$$

In right $$\triangle$$ UVW,

=> $$tan(U)=\frac{VW}{UV}$$

=> $$\frac{24}{7}=\frac{VW}{0.7}$$

=> $$VW=\frac{24}{7}\times0.7=2.4$$ cm

=> Ans - (C)

Create a FREE account and get:

CAT Quant Questions | CAT Quantitative Ability