The ratio of the incomes of P and Q is 16 : 9 and the ratio of their expenditures is 8 : 5.
The saving of P is 220% more than that of Q.R earns 25% less than P and spends 20% more than Q. What is the ratio of P's income to R's savings?

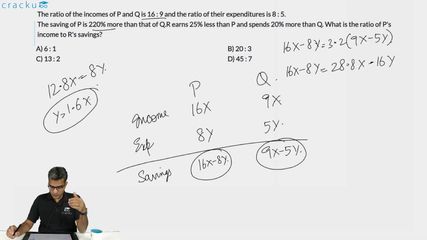
Let P and Q's income be 16x and 9x respectively, and P and Q's expenditure be 8y and 5y respectively, where x and y are constants. 

P's savings = 16x - 8y
Q's savings = 9x - 5y

We are given that P's savings are 220% more or 320% of Q's savings. Using this we get, 
$$16x-8y=\frac{32}{10}\left(9y-5x\right)$$
$$160x-80y=288x-160y$$
$$80y=128x$$
$$\frac{y}{x}=\frac{8}{5}$$

we are given that R's earning are 25% less than P's earnings, using this we get R's earnings to be $$\frac{3}{4}\times\ 16x=12x$$
We are also given that R's expenses are 20% more than Q, using this we get R's expenditure to be $$\frac{6}{5}\times\ 5y=6y$$

This gives R's savings to be 12x - 6y, using the relation between y and x, we can convert this in terms of x, 
$$12x-6\left(\frac{8}{5}x\right)=12x-\frac{48}{5}x=\frac{12}{5}x$$

The ratio of P's income to R's savings would be $$\frac{16x\times\ 5}{12x}$$
=$$\frac{20}{3}$$

Therefore, Option B is the correct answer.