Square and Rectangles Questions for NMAT – Download [PDF]
Download Square and Rectangles Questions for NMAT PDF. Top 10 very important Square and Rectangles Questions for NMAT based on asked questions in previous exam papers.
Download Square and Rectangles Questions for NMAT
Take NMAT mock test
Question 1: A square football field is of length 30 metres. It is surrounded by a footpath of uniform width and the total area of the footpath is 256 sq metres. What is the width of the footpath?
a) 16 metres
b) 8 metres
c) 4 metres
d) 2 metres
e) 1 meter
Question 2: Four horses are tethered at four corners of a square plot of side 14 m so that the adjacent horses can just reach one another. There is a small circular pond of area 20 $m^2$ at the centre. Find the ungrazed area.
[CAT 2002]
a) $22 m^2$
b) $42 m^2$
c) $84 m^2$
d) $168 m^2$
Instructions
A punching machine is used to punch a circular hole of diameter two Units from a square sheet of aluminium of width 2 units, as shown below. The hole is punched such that the circular hole touches one corner P of the square sheet and the diameter of the hole originating at P is in line with a diagonal of the square:
<img “=”” alt=”” class=”img-responsive” src=”https://cracku.in/media/questionGroup/DI_3_5.png”/>
Question 3: Find the area of the part of the circle (round punch) falling outside the square sheet.
[CAT 2006]
a) $\pi$ /4
b) ($\pi$ -1)/2
c) ($\pi$ -1)/4
d) ($\pi$ -2)/2
e) ($\pi$ -2)/4
Question 4: Find the area of the part of the circle (round punch) falling outside the square sheet.
[CAT 2006]
a) $\pi$ /4
b) ($\pi$ -1)/2
c) ($\pi$ -1)/4
d) ($\pi$ -2)/2
e) ($\pi$ -2)/4
Question 5: An equilateral triangle DOC is drawn inside a square ABCD. What is the value of the angle AOB in degrees?[CAT 2006]
a) 75
b) 90
c) 120
d) 135
e) 150
Question 6: The length of a rectangle is decreased by 20% and its breadth is increased by 20%, what is the change in its perimeter?
a) No change
b) 4% increase
c) 4% decrease
d) 10% increase
e) Can’t be determined
Question 7: Neeraj has agreed to mow a lawn, which is a 20 m × 40 m rectangle. He mows it with 1 m wide strip. If Neeraj starts at one corner and mows around the lawn toward the centre, about how many times would he go round before he has mowed half the lawn?
CAT [2002 ]
a) 2.5
b) 3.5
c) 3.8
d) 4
Question 8: In the figure given below, ABCD is a rectangle. The area of the isosceles right triangle ABE = 7 $cm^2$ ; EC = 3(BE). The area of ABCD (in $cm^2$) is
CAT [2002]
a) $21 cm^2$
b) $42 cm^2$
c) $28 cm^2$
d) $56 cm^2$
Question 9: In the given diagram, ABCD is a rectangle with AE = EF = FB. What is the ratio of the areas of CEF and that of the rectangle? [CAT 2001]
[CAT 2001]
a) $\frac{1}{6}$
b) $\frac{1}{8}$
c) $\frac{1}{9}$
d) None of these
Question 10: Consider a rectangle ABCD of area 90 units. The points P and Q trisect AB, and R bisects CD. The diagonal AC intersects the line segments PR and QR at M and N respectively, What is the area of the quadrilateral PQMN?
a) > 9.5 and < 10
b) > 10 and < 10.5
c) > 10.5 and < 11
d) > 11 and < 11.5
e) > 11.5
Join 7K MBA Aspirants Telegram Group
Download Highly Rated CAT preparation App
Answers & Solutions:
1) Answer (D)
The area of the football field and the footpath is $30^{2} + 256 = 1156$ sq metres $= 34^{2}$
So, width of the footpath is (34 – 30)/2 = 2 metres
2) Answer (A)
Length of the rope tied to each horse = 7 m.
Total area of the portion that the horses can graze = 4* $\pi 7^2$/4 = 49$\pi$
Area of the circular pond = 20 $m^2$
So, area left ungrazed = $14^2 – 20 – 49\pi m^2$ = 22 $m^2$ (approx)
3) Answer (D)
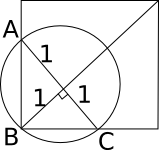
The area of triangle ABC is 1/2 * $\sqrt2$ * $\sqrt2$ = 1
Area of semi-circle ABC = $\pi/2$
So, area of circle outside the square = $\pi/2$ – 1 = ($\pi$ -2)/2
4) Answer (D)

The area of triangle ABC is 1/2 * $\sqrt2$ * $\sqrt2$ = 1
Area of semi-circle ABC = $\pi/2$
So, area of circle outside the square = $\pi/2$ – 1 = ($\pi$ -2)/2
5) Answer (E)

Triangle AOD is isosceles. So, angle DAO = angle DOA = 75. Similarly, angle BOC = 75. So, angle AOB = 150
6) Answer (E)
After change, the perimeter of the rectangle is 2*(80%*l + 120%*b) which can’t be compared to the earlier perimeter unless we know original length and breadth.
7) Answer (C)
For every trip around the lawn, the length of the lawn goes down by 2 metres and breadth goes down by 2 metres. So, if n is the number of trips before the area of the lawn is halved,
(20-2n)*(40-2n) = .5*20*40
800-120n+4$n^{2}$ = 400
The value of n has to be less than 20 to get a meaningful solution.
Solving, we get n = 3.82
8) Answer (D)
Let AB = BE = x . Area of triangle ABE = x^2/2 = 14 we get x= $\sqrt{14}$ So we have side BC = 4*$\sqrt{14}$ . Now area is AB*BC = 14 *4 = 56
9) Answer (A)
Area of triangle CEF = 1/2 * length of rectangle/3 * breadth of rectangle = Area of rectangle/6
So, required ratio = 1:6
10) Answer (D)
Consider the figure given below which shows the different areas

$\triangle$ APM and $\triangle$ CRM are similar triangles [ $\angle$ PAM = $\angle$ MCR as they are alternate interior angles, $\angle$ APM = $\angle$ CRM as they alternate interior angles and $\angle$ AMP = $\angle$ CMR as they are opposite angles. Hence, by A-A-A property, the two triangles are similar triangles]
$\triangle$ ANQ and $\triangle$ CNR are similar triangles [ $\angle$ QAN = $\angle$ NCR as they are alternate interior angles, $\angle$ AQN = $\angle$ CRN as they alternate interior angles and $\angle$ ANQ = $\angle$ CNR as they are opposite angles. Hence, by A-A-A property, the two triangles are similar triangles]
Let x and y be the sides of the rectangle. Hence, xy=90
Let h1 and h2 be the height of the triangles APM and CRM. Hence, h1+h2=y
Length AP = 1/3 x
Length CR = 1/2 x
As APM and CRM are similar triangles, h1 / h2 = AP/CR = (1/3)/ (1/2) = 2/3
h2 = 3/2 h1
h1 + 3/2 h1 = y
h1 = 2/5 y
Hence, area of $\triangle$ APM = 1/2 * AP * h1 = 1/2 * (1/3 x) * (2/5 y) = 1/15 * xy = 1/15 * 90 = 6
Let h3 and h4 be the height of the triangles ANQ and CNR. Hence, h3+h4=y
Length AQ = 2/3 x
Length CR = 1/2 x
As AQN and CRN are similar triangles, h3 / h4 = AQ/CR = (2/3)/ (1/2) = 4/3
h4 = 3/4 h3
h3 + 3/4 h3 = y
h3 = 4/7 y
Hence, area of $\triangle$ AQN = 1/2 * AQ * h3 = 1/2 * (2/3 x) * (4/7 y) =4/21 * 90 = 120 / 7
Hence, area of PQNM = Area of AQN – Area of APM = 120/7 – 6 = (120-42)/7 = 78/7
Hence, area of PQNM is >11 and <11.5
We hope this Square and Rectangles Questions for NMAT pdf for NMAT exam will be highly useful for your Preparation.






