Height and Distance Questions for SSC CGL PDF:
Download SSC CGL Height and Distance questions with answers PDF based on previous papers very useful for SSC CGL exams. 25 Very important Height and Distance objective questions for SSC exams.
Download SSC CGL Height and Distance Questions PDF
25 SSC CGL Mocks – Just Rs. 149
Question 1: A man rides at the rate of 18 km/hr, but stops for 6 mins. to change horses at the end of every 7th km. The time that he will take to cover a distance of 90 km is
a) 6 hrs.
b) 6 hrs 12 min.
c) 6 hrs 18 min.
d) 6 hrs 24 min.
Question 2: In a circle of radius 17 cm, two parallel chords of lengths 30 cm and 16 cm are drawn. If both the chords are on the same side of the centre, then the distance between the chord is
a) 9 cm
b) 7 cm
c) 23 cm
d) 11 cm
Question 3: N is the foot of the perpendicular from a point P of a circle with radius 7 cm, on a diameter AB of the circle. If the length of the chord PB is 12 cm, the distance of the point N from the point B is
a) $12\frac{2}{7}$
b) $3\frac{5}{7}$
c) $10\frac{2}{7}$
d) $6\frac{5}{7}$
Question 4: Two cars are moving with speeds $v1$ and $v2$ towards a crossing along two roads. If their distance from the crossing be 40 m and 50 m at an instant of time, then they do not collide, if their speeds are such that
a) $ v_1:v_2 \neq 4:5 $
b) $ v_1:v_2 \neq 5:4 $
c) $ v_1:v_2=16:25 $
d) $ v_1:v_2 = 25:16 $
Question 5: A rail road curve is to be laid out on a circle. What radius should be used if the track is to change direction by 25$^{\circ}$ in a distance of 40 metres?
a) 91.64 metres
b) 90.46 metres
c) 89.64 metres
d) 93.64 metres
SSC CGL Previous Papers Download PDF
Question 6: If the distance between two point (0, -5) and (x, 0) is 13 unit, then x =
a) 10
b) ± 10
c) 12
d) ± 12
Question 7: Walking at 5 km/hr a student reaches his school from his house 15 minutes early and walking at 3 km/hr he is late by 9 minutes. What is the distance between his school and his house?
a) 5 km
b) 8 km
c) 3 km
d) 2 km
Question 8: Walking at 6/7th of this usual speed a man is 25 minutes too late. His usual time to cover this distance is
a) 2 hours 30 minutes
b) 2 hours 15 minutes
c) 2 hours 25 minutes
d) 2 hours 10 minutes
Question 9: Walking at 3/4th of his usual speed , aman is $1\frac{1}{2}$ hours late. his usual time to cover the same distance, in hours, is?
a) $4\frac{1}{2}$
b) $4$
c) $5\frac{1}{2}$
d) $5$
Question 10: The diameter of a wheel is 98 cm. The number of revolutions in which it will have to cover a distance of 1540 m is
a) 500
b) 600
c) 700
d) 800
18000+ Questions – Free SSC Study Material
Question 11: Jogging at a speed of 6.5 km/hr a man can cover a distance in 18 hours. Running at a speed of 12 km/hr the man covers the same distance in
a) 7.85 hours
b) 10.5 hours
c) 8.36 hours
d) 9.75 hours
Question 12: Raju travels half of the distance to his school on foot at 10 km/hr and remaining half of the distance by bus at 30 km/hr. If the distance between school and his starting point is 60 km, find the time taken by Raju to reach his school?
a) 5 hours
b) 4.8 hours
c) 3.6 hours
d) 4 hours
Question 13: A boat in a still water moves with a speed of $9$ $\text{km/h}$ and the speed of the stream is $1.5$ $\text{km/h}$. A man rows for a distance of $52.5$ $\text{km}$ and returns back to the starting point. The total time taken( in hours ) is:
a) $7$
b) $5$
c) $12$
d) $6$
Question 14: A person walks with $\frac{3}{5}$ times the original speed and reaches the destination 1 hour late. The time taken to travel the distance at the original speed would be
a) 45 Minutes
b) 60 Minutes
c) 75 Minutes
d) 90 Minutes
Question 15: The floor of a room is of size $4m \times 3m$ and its height is 3m. The walls and ceiling of the room require painting. The area to be painted is
a) 66 m2
b) 54 m2
c) 43 m2
d) 33 m2
Question 16: The tops of two poles of height 24 m and 36 m are connected by a wire. If the wire makes an angle of 60 $^{\circ}$ with the horizontal, then the length of the wire is
a) $8\sqrt{3}$
b) $8$
c) $6\sqrt{3}$
d) $6$
Question 17: Two poles of height 7 metre and 12 metre stand on a plane ground. If the distance between their feet is 12 metre, the distance between their top will be
a) 15 metre
b) 13 metre
c) 19 metre
d) 17 metre
Question 18: A boat is sailing towards a lighthouse of height 20√3 m at a certain speed. The angle of elevation of the top of the lighthouse changes from 30° to 60° in 10 seconds. What is the time taken (in seconds) by the boat to reach the lighthouse from its initial position?
a) 10
b) 15
c) 20
d) 60
Question 19: The length of two parallel sides of a trapezium are 30 cm and 40 cm. If the area of the trapezium is 350 cm2, then what is the value (in cm) of its height?
a) 8
b) 10
c) 15
d) 12
Question 20: The length of two parallel sides of a trapezium are 15 cm and 20 cm If its area is 175 sq.cm, then its height is :
a) 10 cm
b) 15 cm
c) 25 cm
d) 20 cm
100+ Free GK Tests for SSC Exams
Question 21: The average height of a class comprising of 40 students in 155 cm. When two of the students leave the class, the average height of the class drops down to 153 cm. What is the average height of the two students who left the class?
a) 193 cm
b) 178 cm
c) 185 cm
d) 166 cm
Question 22: The average height of a class is 160 cm. If the average height of boys is 172cm and that of girls is 136cm, what is the ratio of number of boys to that of number of girls in the class?
a) 1:2
b) 3:2
c) 2:1
d) 2:3
Question 23: The average height of 30 boys out of a class of 50 is 160 cm. If the average height of the remaining boys is 165 cm, the average height of the whole class (in cm) is:
a) 161
b) 162
c) 163
d) 164
Question 24: The average height of 8 students is 152 cm. Two more students of heights 144 cm and 155 cm join the group. What is the new average height ?
a) 151.5 cm
b) 152.5 cm
c) 151 cm
d) 150.5 cm
Question 25: Out of 20 boys, 6 are each of 1 m 15 cm height, 8 are of 1 m 10 cm and rest of 1m 12cm. The average height of all of them is
a) 1m 12cm
b) 1m 121cm
c) 1m 211cm
d) 1m 21cm
1500+ Free SSC Questions & Answers
Answers & Solutions:
1) Answer (B)
Speed of man = 18 km/h
Total distance = 90 km
As he stops after 7th km, => $90=(12\times7)+6$
=> He stops 12 times in the journey.
Total stoppage time = $12\times6=72$ min
Real time = $\frac{90}{18}=5$ hours
$\therefore$ Required time taken = 5hr + 72 min
= 6 hrs 12 min
=> Ans – (B)
2) Answer (B)
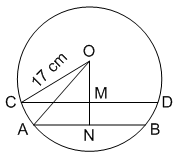
Given : CD = 30 cm and AB = 16 cm
To find : MN = ?
Solution : Perpendicular distance from the centre bisects the chord.
=> CM = $\frac{30}{2}=15$ cm and AN = $8$ cm
Now, in $\triangle$ OCM,
=> $(OM)^2=(OC)^2-(CM)^2$
=> $(OM)^2=(17)^2-(15)^2$
=> $(OM)^2=289-225=64$
=> $OM=\sqrt{64}=8$ cm
Similarly, in $\triangle$ OAN,
=> $(ON)^2=(OA)^2-(AN)^2$
=> $(ON)^2=(17)^2-(8)^2$
=> $(ON)^2=289-64=225$
=> $ON=\sqrt{225}=15$ cm
$\therefore$ MN = ON – OM
= $15-8=7$ cm
=> Ans – (B)
3) Answer (C)
As we know $cos\theta$ = $\frac{a^2 + b^2 – c^2}{2ab}$ (where $\theta$ is angle between sides a and b, side c is opposite to angle $\theta$ )
Hence for triangle POB (where O is centre of circle)
OP=OB=radius of circle=7
PB=12
Let’s say angle between PB and OB is $\theta$.
So putting up values and solving it, we will get $cos\theta$ = $\frac{6}{7}$
Hence value of BN will be BP $cos\theta = 12cos\theta = \frac{72}{7}$
4) Answer (A)
they will collide if time to reach the crossing is same.
it is the case when
time taken by 1st car = time taken by 2nd car.
40/v1 = 50/v2
v1/v2 = 4/5
but condition not to collide is v1/v2 $\neq$ 4/5.
so the answer is option A.
5) Answer (A)
With 25 degree angle length of arc will be ($r \times$ ($\theta$ in radian) = 40m (where $r$ is radius of arc and $\theta$ will be angle made by it)
Now solving above equation, we will get $r$ = 91.64 m.
6) Answer (D)
$Distance^{2} = diff.of x cordinates^{2}+diff.of y coordinates^{2}$
$13^{2}=x^{2}+5^{2}$
Hence $x=\pm12$
7) Answer (C)
Let the time and distance be t mins and d respectively,
In the first case:
Total time taken = (t – 15) mins = (t-15)/60 hrs.
Distance travelled = 5*(t-15)/60
In the second case:
Total time taken = (t + 9) mins = (t+9)/60 hrs.
Distance travelled = 3*(t+9)/60
So, 5*(t-15)/60 = 3*(t+9)/60,
Solving the above equation we get, t=51
So, d=3*(51+9)/60
=3 KMs
8) Answer (A)
Let the initial speed and time be s,t respectively,
then speed and time in the next case are 6s/7 and (t+25)
As distance = speed * time, and distance travelled in both cases is the same,
(6s/7)*(t+25) = s*t
Solving the above equation results in t=150min
9) Answer (A)
As distance is constant
Hence $v_1 \times t_1 = \frac{3v_1}{4} \times (t_1 + \frac{3}{2})$ (Where is $v_1$ is speed, t_1 is time taken to travel)
On solving above equation, we will get $t_1 = \frac{9}{2}$
10) Answer (A)
In 1 revolution wheel will complete a distance of $2\pi r = 2 \times \pi \times \frac{98}{2}$ = 308 cm.
Hence to cover 1540 m. , revolutions will be = $\frac{1540\times100}{308} = 500$
11) Answer (D)
Distance he covers = 6.5*18 = 117 km
Time taken by the man to cover the distance while running = 117/12 = 9.75 hours
12) Answer (D)
Total time taken to reach Raju’s school = $\frac{30}{10} + \frac{30}{30}$ = 3+1 = 4 hours
13) Answer (C)
Speed of the man in downstream = $( 9 + 1.5 )$ km/h = $10.5$ km/h
Speed of the man in upstream = $( 9 – 1.5 )$ km/h = $7.5$ km/h
Time taken while going upstream = $\frac{22.5}{7.5}$ hr = $7$ hr
Time taken while going upstream = $\frac{22.5}{10.5}$ hr = $5$ hr
Total Time taken = $(7+5)$ hr = $12$ hr
Hence , the correct option is C
14) Answer (D)
Since Time and Speed are inversely proportional
Therefore , $\frac{5}{3}$( Usual Time ) – ( Usual Time ) = 1
$\implies$ $\frac{2}{3}$( Usual Time ) = 1
Hence , Usual Time = $\frac{3}{2}$ Hours = 90 Minutes
Correct Option is D
15) Answer (B)
Area of walls and ceiling of room = area of 4 walls + area of 1 ceiling
= $(2 \times (3 \times 3) + 2\times (4 \times 3)) + 4 \times 3$
= 18 + 24 + 12 = 54
16) Answer (A)
Let’s say height of bigger pole is 12+24.
Where length of wire will be hypotenuse, pole length of 12 in bigger pole will be perpendicular and distance between poles will be base.
And angle between base and hypotenuse will be $\theta$
Hence length of wire will be = $12cosec\theta$ (where $\theta=60^o$)
So length of wire will be = $12 \frac{2}{\sqrt3} = 8\sqrt3$
17) Answer (B)
Height of pole AB = 7 metre = DE and CD = 12 metre
BD = 12 metre = AE
Now, CE = CD-DE = 12-7 = 5 metre
In $\triangle$ ACE
AC = $\sqrt{(AE)^2 + (CE)^2}$
= $\sqrt{12^2 + 5^2} = \sqrt{169}$
= 13 metre
18) Answer (B)

Given : CD = $20\sqrt3$ m and time taken to reach B from A = 10 seconds
To find : Time taken to reach D from A
Solution : In $\triangle$ BCD,
=> $tan(60^\circ)=\frac{CD}{BD}$
=> $\sqrt3=\frac{20\sqrt3}{BD}$
=> $BD=20$ m
Similarly, in $\triangle$ ACD,
=> $tan(30^\circ)=\frac{CD}{AD}$
=> $\frac{1}{\sqrt3}=\frac{20\sqrt3}{20+AB}$
=> $AB+20=60$
=> $AB=60-20=40$ m
=> Speed of boat (while travelling from A to B) = distance/time
= $\frac{40}{10}=4$ m/s
=> AD = BD + AB = 20 + 40 = 60 m
$\therefore$ Time taken to reach D from A = $\frac{60}{4}=15$ seconds
=> Ans – (B)
19) Answer (B)
Sum of two parallel sides of a trapezium = $30+40=70$ cm
Let height = $h$ cm
=> Area of trapezium = $\frac{1}{2}\times h\times$ (sum of parallel sides)
=> $\frac{1}{2}\times(h)\times(70)=350$
=> $\frac{h}{2}=\frac{350}{70}=5$
=> $h=5\times2=10$ cm
=> Ans – (B)
20) Answer (A)
Sum of the two parallel sides of the trapezium = $15+20=35$ cm
Let its height = $h$ cm
=> Area of trapezium = $\frac{1}{2}\times$ (sum of parallel sides) $\times$ height
=> $\frac{1}{2}\times35\times h=175$
=> $h=\frac{175}{35}\times2$
=> $h=5\times2=10$ cm
=> Ans – (A)
21) Answer (A)
Let the average height of the two students be x.
So, sum of their ages will be 2x
The sum of the heights of all the students in the class will be 155 X 40
This should be equal to-
155 X 40 = 153 X 38 + 2x
38(155-153) + 2 X 155 = 2x
=> x = 38 + 155 = 193cm
22) Answer (C)
Let the number of boys in the class be ‘b’ and number of girls in the class be ‘g’.
$b\times 172 +g \times 136 = (b+g) \times 160$
$12b = 24g$
$b:g = 2:1$
Hence, option c is the correct answer.
23) Answer (B)
Total boys in class = 50
Average height of first 30 boys = 160 cm
=> Total height of 30 boys = $30 \times 160=4800$ cm
Similarly, total height of remaining 20 boys = $20 \times 165=3300$ cm
$\therefore$ Average height of the whole class = $\frac{(4800+3300)}{30+20}$
= $\frac{8100}{50}=162$ cm
=> Ans – (B)
24) Answer (A)
Average height of 8 students = 152 cm
=> Total height of 8 students = $152\times8=1216$ cm
After addition of 2 students, total students of 10 students = $1216+144+155=1515$
$\therefore$ New average height = $\frac{1515}{10}=151.5$ cm
=> Ans – (A)
25) Answer (B)
Total number of boys = 20
Total height of first 6 boys = $6\times1.15=6.9$ m
Total height of next 8 boys = $8\times1.10=8.8$ m
Total height of last 6 boys = $6\times1.12=6.72$ m
=> Average height = $\frac{(6.9+8.8+6.72)}{20}$
= $\frac{22.42}{20}=1.121=$ 1m 121 cm
=> Ans – (B)







