Geometry Questions for RRB NTPC Set-3 PDF
Very important Geometry questions based on asked questions in previous papers. Download PDF of Top-15 RRB NTPC Geometry questions.
Download Geometry Questions for RRB NTPC Set-3 PDF
Take a free mock test for RRB NTPC
Download RRB NTPC Previous Papers PDF
Question 1: The three sides of a triangle are in the ratio 3:4:5. If the perimeter of the triangle is 48 cm, what is the area of the triangle?
a) 24 $cm^2$
b) 72 $cm^2$
c) 48 $cm^2$
d) 96 $cm^2$
Question 2: The perimeters of a square and a rectangle are equal. If the ratio of the length to breadth of the rectangle is 2:1, what is the ratio of the area of the rectangle to the area of the square?
a) 7 : 8
b) 8 : 9
c) 6 : 7
d) 4 : 5
Question 3: The length and breadth of a rectangle are in the ratio 4:3. A parallelogram is now superimposed on this rectangle such that the two parallel sides of the parallelogram coincide with the two longer sides of the rectangle and one side of the parallelogram is exactly coincident with one of the longer sides of the rectangle. One of the angles of the parallelogram is 120 degrees. Approximately, what percentage of the area of the rectangle is overlapped by the parallelogram? ($\sqrt3$ = 1.732)
a) 55%
b) 78%
c) 96%
d) 35%
Question 4: The length of the altitude AD in triangle ABC is 14 cm. If the length of the side AB is 15 cm and AB and BC are in the ratio 3 : 5, what is the length of the altitude drawn from C?
a) 15 cm
b) 21 cm
c) 23.33 cm
d) 27.67 cm
Question 5: The percentage of increase in the perimeter of a square is 20%. What is the percentage increase in the area of the square?
a) 44%
b) 22%
c) 33%
d) 50%
RRB NTPC Previous Papers [Download PDF]
Question 6: tan90C is undefined. As $ \theta $ is increased from 89C towards 90C value of tan $ \theta $ tends to
a) 0
b) $ +\alpha $
c) 1
d) underfined
Question 7: The value of $sin^{2} 30 + sin ^{2} 60 $ is
a) 1
b) $\frac{3}{2}$
c) 2
d) $\frac{3}{4}$
Question 8: If two sides of a triangle, the included angle and another angle are given, how many triangles can be constructed?
a) 0
b) 1
c) 2
d) 3
Question 9: A third angle orthographic projection of an object is given below. What is the object ?
a) Triangle
b) Trapezium
c) Cone
d) Frustrum of a cone
Question 10: How many lines can be said to exist or be drawn in a three dimensional space, which are mutually perpendicular to each other ?
a) 2
b) 3
c) 4
d) 8
Question 11: In coordinate geometry, distance of the point (-4, 3) from the origin
a) 3
b) 4
c) 5
d) 25
Question 12: Two tangents are drawn to a circle of radius 10 cm. The tangents are parallel to each other. What is the distance between the two tangents ?
a) 10 cm
b) 20 cm
c) $10\sqrt{2}$
d) $10\sqrt{3}$
Question 13: How many cubes are there in the figure ?
a) 6
b) 8
c) 9
d) 10
Question 14: Length and breadth of a rectangle are in the ratio 3:4 while the perimeter of it is 56 cm.What is the area of the rectangle ?
a) 192 sq.cm
b) 144 sq.cm
c) 196 sq.cm
d) 158 sq.cm
Question 15: A carpenter bought a rectangular white board of dimension 4 feet x 3 feet, which cost him Rs 12 per square feet.The cost of fixing aluminum lining to all the edges was Rs 4 per feet.At what price should he sell the finished white board so as to gain 25% on the cost price ?
a) Rs 225
b) Rs 250
c) Rs 275
d) Rs 300
Download General Science Notes PDF
Answers & Solutions:
1) Answer (D)
The ratio of the sides of the triangle is 3:4:5 and the perimeter is 48 cm, so the sides are 12 cm, 16 cm and 20 cm. There are two ways to find the area of the triangle.
The triangle is a right-angled triangle because $12^2 + 16^2 = 20^2$.
So, the area is given by ½ * 12 * 16 = 96 $cm^2$.
Since the sides of the triangle are known, the area can also be found out by applying the formula A = $\sqrt{s*(s-a)*(s-b)*(s-c)}$, where ‘s’ is the semi-perimeter of the triangle given by s = (a+b+c)/2 and ‘a’, ‘b’, ‘c’ are the sides of the triangle.
Here, s = (12 + 16 + 20)/2 = 24 cm
Area = $\sqrt{24 * (24 – 12) * (24 – 16) * (24 – 20)}$ = $\sqrt{24 * 12 * 8 * 4}$ = 12 * 2 * 2 * 2 = 12 * 8 = 96 $cm^2$
2) Answer (B)
Let the sides of the rectangle be 2k and k. So, area = $2k^2$
Perimeter of the rectangle = 6k
So, side of the square = 6k/4 = 1.5k
Area of the square = $(1.5k)^2$ = $2.25k^2$
So, required ratio = 2 : 2.25 = 8 : 9
3) Answer (B)
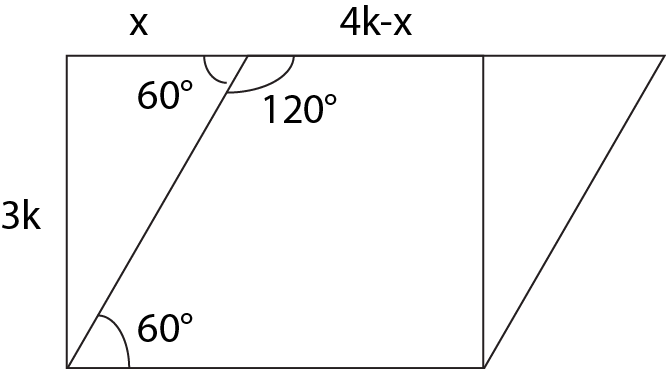
Since one angle of the parallelogram is 120 degrees, the other angle is 60 degrees.
From the figure, the required percentage = (1 – Area of the triangle/Area of the rectangle) * 100%
Tan 60 = $\sqrt3$ = 3k/x => x = $\sqrt3$k
So, required percentage = (1 – ½ * $\sqrt3$k * 3k/[3k * 4k]) * 100% = $(8-\sqrt3)/8$ * 100% = 78.3% = 78% approximately
4) Answer (C)

The area of the triangle is given by ½ * base * height.
If the base is BC, then the height is AD. If the base is AB, the height is the altitude drawn from vertex C on AB.
So, area = ½ * BC * AD = ½ * AB * CE
=> CE = BC * AD/AB = 5 * 14/3 = 70/3 = 23.33 cm
5) Answer (A)
Let the side of the square be ‘s’. Perimeter = 4s, Area = $s^2$
New perimeter = 120/100 * 4s = 24/5 s = 4.8s
So, new side = 4.8s/4 = 1.2s
So, new area = $(1.2s)^2$ = $1.44s^2$
So, percentage increase in area = (1.44 – 1)/1 * 100% = 44%
6) Answer (B)
Tan 90 is undefined and Tan 89 is positive.
So, as angle is increased from 89 to 90, tan $ \theta $ tends to positive infinity.
7) Answer (A)
sin 30 = 1/2
sin 60 = $ \sqrt{3}/2$
So $ (\frac {1}{2})^ {2} + (\frac {\sqrt{3}}{2})^ {2} $ = 1
8) Answer (C)
If the given two sides are a and b, then the second angle can be between the a and the third side or b and the third side.
Hence, 2 triangles can be drawn using the given measurements.
9) Answer (D)
By cutting a portion of the cone parallel to its base at the top, a frustum is formed.
The first and second figures are top view and side view of a frustum.
10) Answer (B)
In a three dimensional space, three axes exist – X, Y and Z.
11) Answer (C)
Distance between (-4,3) and (0,0) = $\sqrt{(0+4)^2+(0-3)^2}$ = $\sqrt{25}$ = 5
12) Answer (B)
If the tangents are parallel to each other, the distance between them is equal to its diameter.
13) Answer (D)
There are 3 hidden cubes in the first row and 1 hidden cube in the second row. So, in total there are 3 + 3 + 3 + 1 = 10 cubes.
14) Answer (A)
The length and breadth are in the ratio 3:4
Let them be 3x and 4x respectively.
Perimeter = 56
So, 2(7x) = 56
So, x = 4
Area = 3x * 4x = 3*4*4*4 = 192 sq cms
15) Answer (B)
The cost of the board = 4x3x12= 144
Cost of the aluminium lining = (4+4+3+3)x4 = 56
So total cost = 200 Rs
Selling price = 200 x 1.25 = 250 Rs
DOWNLOAD APP FOR RRB FREE MOCKS
We hope this Geometry Questions Set-3 pdf for RRB NTPC Exam will be highly useful for your Preparation.





