CAT Questions on Boats and Streams:

CAT Questions on Boats and Streams

CAT Mocks for just Rs. 1199

Download CAT Quant Questions PDF

Take Free Mock Test for CAT 2018

Question 1: 

Elite rowers can row upstream from Haridwar to Rishikesh in 5 hours. The amount of time they take to row in still water for the same distance is 50 mins more than the time they take to row downstream. What is the time taken by them to row downstream from Rishikesh to Haridwar (given that it is greater than 2 hours)?

a) 3.5 hours
b) 2.25 hours
c) 3 hours
d) 2.5 hours

Question 2:

Vijay and Sujay start from two points P and Q on a river and travel towards each other. If they had been travelling in still water they would have met at a point R which is thrice as distant from Q as it is from P. If Sujay had been travelling along the stream and Vijay against it they would have met in 1 hour. What is the time (in minutes) they would take to meet if Vijay had been travelling along the stream and Sujay against it?

Answer: 60

Solution:
In both the cases the distance between them would be the same and also their relative speed moving towards each other would also be the same, because while calculating relative speed the speed of the stream would get cancelled.
Hence both ways the time taken will be 60 minutes.

Download CAT 2018 Syllabus PDF

Question 3:

A group of people was rowing a boat upstream in a river. They spotted a dog drowning and flowing downstream with the river. To save the dog from drowning, they immediately started rowing at their full capacity i.e. 20 m/s in still water. As soon as they reached the dog, they stopped rowing. It took them 18 seconds to pull the dog into the boat, while both the dog and the boat were flowing with the water current. As soon as they pulled the dog into the boat, they started rowing downstream at their full capacity and reached the point where they first spotted the dog in 45 seconds. It is given that the water current is 2 m/s. What was the distance (in metres) between the dog and the boat when they first spotted him?

Answer: 1140

Solution:

Consider the following figure-

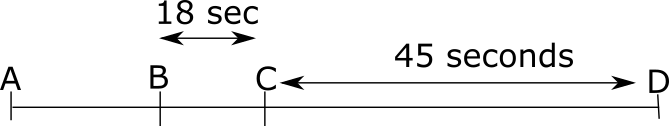
Imagine that the dog was at A and the boat was at D when they first spotted the dog.

B is the point where they met the dog. The boat travelled from D to B upstream and in the same time the dog travelled downstream with the speed of stream from A to B.

During BC, both the dog and the boat were flowing with the stream at 2 m/s.

During CD, the boat was moving at full capacity downstream.

Speed of the boat downstream = 20+2 = 22 m/s and speed of the boat upstream = 20-2 = 18 m/s.

Length of BC = 2m/s X 18sec= 36 m

Length of CD = 22 m/s X 45 sec = 990 m.

In the time the boat travelled DB upstream, the dog travelled the distance AB downstream with the water current.

We know that DB = DC + CB = 990 + 36 = 1026

Time taken by the boat to travel 1026m upstream = 1026/18 = 57 sec.

The same time is taken by the dog to travel from A to B along with the stream.

=> AB = 57 X 2m/s = 114m

Thus, the distance AD = AB+BC+CD = 114+990+36 = 1140m

Question 4:

A boat goes from A to B upstream in 6 hours and comes back from B to A downstream in 4 hours. If the speed of the stream is one fifth of the speed of the boat, then find the distance(in km) between A and B?

a) 48 km
b) 96 km
c) 60 km
d) cannot be determined

Preparation tips for CAT – Beginners

How to prepare for CAT by Self-Study

Solutions: (1 and 4 )

1) Answer (d)

Let the speed of the water be W and the speed of rowing in still water be R. Let the distance between Haridwar and Rishikesh be S.
So, $$\frac{S}{R-W} = 5$$
And, $$\frac{S}{R} – \frac{S}{R+W} = \frac{5}{6}$$
Therefore, $$\frac{SW}{R(R+W)} = \frac{5}{6}$$
Dividing first equation by this, we get, $$\frac{R(R+W)}{W(R-W)} = 6$$
Or, $$R^2 + WR = 6WR – 6W^2$$ or $$R^2 – 5WR + 6W^2 = 0$$
Therefore, $$R = 2W$$ or $$R=3W$$
If R=2W, S = 5W and time taken to row downstream is 5/3 = 100 minutes.
If R=3W S = 10W and time taken to row downstream is 10/4 = 2.5 hours.

As time taken to row downstream is given to be greater than 2 hours, the correct answer is 2.5 hours.

4) Answer (d)

Let the distance between A and B be ‘d’. Let the speed of the boat in still water be 5x km/hr. So the speed of the stream would be x km/hr
Now according to the question,
d/4x – d/6x = 2 => d/12x = 2
=> d = 24x
Thus we only get the relationship between the speed and the distance. Since we do not know any other parameter, we can not find the value of ‘d’. Thus, option d is the correct choice

Take a free CAT online mock test

Download Free CAT Preparation App