Mensuration Problems For SSC CGL
Download SSC CGL Mensuration Problems questions with answers PDF based on previous papers very useful for SSC CGL exams. 20 Very important Mensuration Problems on objective questions (MCQ’s) for SSC exams.
Download Mensuration Problems For SSC CGL
25 SSC CGL Mocks – Just Rs. 149
Question 1: If a metallic cone of radius 30 cm and height 40 cm is melted and recast into metallic spheres of radius 10 cm, find the number of spheres formed.
a) 5
b) 9
c) 6
d) 12
Question 2: find the volume of a cube if the perimeter of one face of the cube is 30 cm.
a) 400.175
b) 472.175
c) 362.725
d) 421.875
Question 3: A solid spherical copper ball, whose diameter is 42 cm, is melted and converted into a wire having diameter equal to 42 cm. The length of the wire is
a) 28
b) 14
c) $\large\frac{56}{3}$
d) $\large\frac{28}{3}$
Question 4: The radii of two cylinders are in the ratio 2:3. Their curved surface areas are in the ratio 4:7. Then find the ratio between their volumes.
a) 8:23
b) 7:24
c) 8:21
d) 23:8
Question 5: The radii of two cylinders are in the ratio 3:5 and their Curved Surface Areas are in the ratio 5:8. Find the ratio between their volumes.
a) 9:24
b) 8:23
c) 25:24
d) 24:8
SSC CGL Previous Papers Download PDF
SSC CHSL PREVIOUS PAPERS DOWNLOAD
Question 6: The base of a right prism, whose height is 2 cm, is a square. If the total surface area of the prism is 10 cm2, then its volume is:
a) $3\ cm^{3}$
b) $1\ cm^{3}$
c) $2\ cm^{3}$
d) $4\ cm^{3}$
Question 7: The radius of a wire is decreased to one third. If volume remains the same, length will increase by:
a) 6 times
b) 1 time
c) 3 times
d) 9 times
Question 8: What is the ratio of volume of cylinder to the volume of cone if their heights are in the ratio of 3:2 (i.e height of cylinder to height of cone) and the radius of the cylinder is 1.428 times the radius of the cone.
a) 25:49
b) 49:50
c) 50:49
d) 49:25
Question 9: A solid spherical copper ball, whose diameter is 14 cm, is melted and converted into a wire having diameter equal to 14 cm. The length of the wire is
a) 27 cm
b) $\frac{16}{3}$
c) 15 cm
d) $\frac{28}{3}$
Question 10: A cylindrical well of height 20 metres and radius 14 metres is dug in a field 72 metres long and 44 metres wide. The earth taken out is spread evenly on the field. What is the increase (in metre) in the level of the field?
a) 6.67
b) 3.56
c) 5.61
d) 4.83
18000+ Questions – Free SSC Study Material
Question 11: If the diameter of a circle is the side of a square, then the ratio of the areas of the circle and the square is
a) $\pi : 1$
b) $\pi : 4$
c) $\pi : 2$
d) $\pi : 8$
Question 12: The area of the iron sheet required to prepare a cone 40 cm high with base radius 9 cm is (Take $\pi = \frac{22}{7}$)
a) ${\large\frac{9900}{7}}$
b) ${\large\frac{9800}{7}}$
c) ${\large\frac{18000}{7}}$
d) ${\large\frac{11000}{7}}$
Question 13: find the area of an equilateral triangle if the height of the triangle is 24 cm.
a) $192\sqrt{3} cm^2$
b) $175\sqrt{3} cm^2$
c) $178\sqrt{3} cm^2$
d) $164\sqrt{3} cm^2$
Question 14: Three sides of a triangular meadow are of length 28 m, 45 m and 53 m long respectively. Find the cost of sowing seeds(in rupees per sq.m) in the meadow at the rate of 12 rupees per sq.m.
a) 7560
b) 6860
c) 7960
d) 7860
Question 15: In an equilateral triangle,if h-R=15 cm where h=height of the triangle and R=circumradius then what is the area of the triangle ?
a) $275\sqrt{3}$
b) $225\sqrt{3}$
c) $500\sqrt{3}$
d) $675\sqrt{3}$
Question 16: In an equilateral triangle,if h-R=5 cm where h=height of the triangle and R=circumradius then what is the area of the triangle ?
a) $50\sqrt{3}$
b) $100\sqrt{3}$
c) $75\sqrt{3}$
d) $25\sqrt{3}$
Question 17: Find the area(in sq.cm) of the sector subtending 72° at the centre of a circle with a radius of 35cm ?
a) 690
b) 734
c) 770
d) 792
Question 18: Find the percentage increase in its area, if the length and breadth of a rectangle increases by 30% and 25% each.
a) 54.25
b) 48.75
c) 58.25
d) 62.5
Question 19: A wire of length 704 cm is used to form a circle. What will be the area$(in m^2)$ of the circle formed?
a) 39424
b) 3.9424
c) 39246
d) 36424
Question 20: Find the maximum possible area$(in cm^2)$ of a triangle which can be formed using a thread of length 36 cm.?
a) $45$
b) $39\sqrt{3}$
c) $36\sqrt{3}$
d) $36$
1500+ Free SSC Questions & Answers
Answers & Solutions:
1) Answer (B)
Given, a metallic cone of radius 30 cm and height 40 cm
Volume of metallic cone = $\dfrac{1}{3} \pi r^2 h$
= $\dfrac{1}{3} \pi * 30^2 * 40 = 12000 \pi cm^3$
metallic spheres of radius 10 cm
Volume of sphere = $\dfrac{4}{3} \pi R^3$
= $\dfrac{4}{3} \pi * 10^3 = \dfrac{4000 \pi}{3} cm^3$
=> Required no. of spheres = $\dfrac{12000 \pi}{\dfrac{4000 \pi}{3}}$
= 9
2) Answer (D)
Given that one face of a cube is a square with perimeter 30 cm
Let each side of the cube be $a$
=> 4 $a$ = 30
=> $a$ = 7.5
Volume of cube = $7.5^3$
= 421.875 $cm^3$
3) Answer (A)
Given, spherical copper ball, whose diameter is 42 cm
Radius of spherical copper ball(r) = 21 cm
Volume of sphere = $\frac{4}{3}\pi r^3$
Radius of Cylindrical wire(R) = 21cm
Let length of wire be ‘L’
Volume of cylinder = $\pi R^2L$
Here Volume of sphere = Volume of sphere
$\large\frac{4}{3}$ $\pi r^3$ = $\pi R^2L$
$\large\frac{4}{3}$ $\times21\times21\times21$ = 21$\times$21$\times$L
$\large\therefore$ Length of the wire ‘L’ = $28$ cm
4) Answer (C)
Given $\dfrac{r_1}{r_2} = \dfrac{2}{3}$
Curved Surface Area $= 2\pi r h$
⇒ $\dfrac{2 \pi r_1 h_1}{2 \pi r_2 h_2} = \dfrac{4}{7}$
⇒ $\dfrac{2}{3} \times \dfrac{h_1}{h_2} = \dfrac{4}{7}$
⇒ $\dfrac{h_1}{h_2} = \dfrac{6}{7}$
Volume $= \pi r^2 h$
⇒ $\dfrac{\pi r_1^2 h_1}{\pi r_2^2 h_2} = ((\dfrac{r_1}{r_2})^2) \times (\dfrac{h_1}{h_2})$
= $\dfrac{4}{9} \times \dfrac{6}{7} = \dfrac{8}{21}$
5) Answer (A)
Given $\dfrac{r_1}{r_2} = \dfrac{3}{5}$
Curved Surface Area $= 2\pi r h$
⇒ $\dfrac{2 \pi r_1 h_1}{2 \pi r_2 h_2} = \dfrac{5}{8}$
⇒ $\dfrac{3}{5} \times \dfrac{h_1}{h_2} = \dfrac{5}{8}$
⇒ $\dfrac{h_1}{h_2} = \dfrac{25}{24}$
Volume $= \pi r^2 h$
⇒ $\dfrac{\pi r_1^2 h_1}{\pi r_2^2 h_2} = ((\dfrac{r_1}{r_2})^2) \times (\dfrac{h_1}{h_2})$
= $\dfrac{9}{25} \times \dfrac{25}{24} = \dfrac{9}{24}$
100+ Free GK Tests for SSC Exams
6) Answer (C)
Let side of base = $a$ cm and height = $h=2$ cm
Total surface area of prism = Curved surface area + (base+top) area
=> $10$ = Perimeter of base $\times$ height + $2\times$ area of base
=> $(4\times a\times2)+(2\times a^2)=10$
=> $a^2+4a-5=0$
=> $a^2+5a-a-5=0$
=> $a(a+5)-1(a+5)=0$
=> $(a+5)(a-1)=0$
=> $a=1$ $[\because a$ cannot be negative.$]$
$\therefore$ Volume = Base area $\times$ height
= $(1)^2\times2=2$ $cm^3$
=> Ans – (C)
7) Answer (D)
Let radius of wire is $r=3$ cm and length = $h=1$ cm
=> Volume of cylinderical wire = $\pi r^2h$
= $\pi\times(3)^2\times1=9\pi$ $cm^2$
New radius = $r’=\frac{1}{3}\times3=1$ cm
Let new length = $h’$ cm
If volume remains the same, => $\pi (r’)^2\times(h’)=9\pi$
=> $(1)^2\times(h’)=9$
=> $h’=9$
$\therefore$ Length was increased by = $\frac{h’}{h}=9$
=> Ans – (D)
8) Answer (C)
Radius of cylinder=$\frac{10}{7}$(radius of cone)
$\frac{r_{cyli}}{r_{cone}}$=$\frac{10}{7}$
Ratio of heights $\frac{h_{cyli}}{h_{cone}}$=$\frac{1}{2}$
Volume of cone= $\frac{1}{3}$ $\times $ $\pi $ $\times $ $r_{cone} $ $\times $ $r_{cone} $ $\times h $
Volume of cylinder=$\pi $ $\times $ $ r_{cyli} $ $\times $ $r_{cyli} $ $\times h $
Required ratio=50:49
9) Answer (D)
Radius of spherical copper ball(r) = 7cm
Volume of sphere = $\frac{4}{3}\prod r^3$
Radius of Cylindrical wire(R) = 7cm
Let length of wire be ‘L’
Volume of cylinder = $\prod R^2L$
Here Volume of sphere = Volume of sphere
$\frac{4}{3}\prod r^3$ = $\prod R^2L$
$\frac{4}{3}\times7\times7\times7$ = 7$\times$7$\times$L
$\therefore$ Length of the wire ‘L’ = $\frac{28}{3}$ cm
10) Answer (D)
Increase in the level of the field is the height of field (cuboidal shape) when volume of well (cylinderical) is equal to the volume of field (cuboidal).
Radius of well = $R=14$ m and height = $H=20$ m
Length of field = $l=72$ m and width = $b=44$ m
Let height = $h$ m
=> Volume of cuboid = Volume of cylinder
Now, volume of cuboid = (Area of rectangle – Area of circle) $\times$ height
=> $(lb-\pi R^2)\times h=\pi R^2H$
=> $[(72\times44)-(\frac{22}{7}\times14^2)]\times(h)=\frac{22}{7}\times(14)^2\times20$
=> $(3168-616)h=44\times280$
=> $h=\frac{44\times280}{2552}\approx4.83$ m
=> Ans – (D)
11) Answer (B)
Let the radius be $x$. Since the diameter is equal to the side of square
=> Side of square = $2x$
Ratio of area of circle to that of square
= $\large\frac{\pi (x)^2}{(2x)^2}$
= $\large\frac{\pi}{4}$
=> Required ratio = $\pi : 4$
12) Answer (A)
We know that the
Surface area of cone= $\large\frac{22}{7}$ $\times r\times(r+L)$
where L=slant height L= $\sqrt{(r)^{2} + (h)^{2}}$
Here when $r=9$ and $h=40$,
L= $\sqrt{(9^{2} + (40)^{2})}$ = $\sqrt{1681}$ = 41 cm
Area, A= ${\large\frac{22}{7}}$ $\times9\times(9+41)$
A= ${\large\frac{9900}{7}}(cm)^{2}$
15000 Questions – Free SSC Study Material
13) Answer (A)
Given,
AD = 24 cm and ABC is an equilateral triangle
In an equilateral triangle all the angles are equal to 60°
In $\triangle$ADC
=> $tan \angle ACD = \frac{AD}{DC}$
=> $tan 60° = \frac{24}{DC}$
=> DC = $\frac{24}{\sqrt{3}} = 8\sqrt{3}$
=> BC = 2*DC = $16\sqrt{3}$
Area of $\triangle$ ABC = $\frac{\sqrt{3}}{4} * side^2$
= $\frac{\sqrt{3}}{4} * (16\sqrt{3})^2$
= $192\sqrt{3} cm^2$
14) Answer (A)
Given the sides of triangle are 28 m, 45 m and 53 m
Since, $28^2 + 45^2 = 784+2025 = 2809 = 53^2$
=> The given sides are of right angled triangle as,
$c^2 + b^2 = a^2$where a, b, c are the sides of the triangle.
=> Area of triangular field = $\large\frac{1}{2}$ $ \times 28 \times 45= 630 m^2$
=> Cost of sowing seeds = $12 \times 630 = Rs. 7560 rupees/m^2$
15) Answer (D)
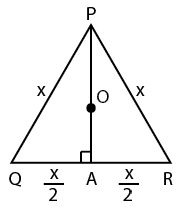
In an equilateral triangle,all the points such as orthocentre,centroid,circumcenter coincide.
Let the triangle be PQR and the circumcentre be O. let median intersect QR at A
Centroid divides median in the ratio 2:1.PA=h,OA=R
OA=15 cm
Therefore (PO:OA)=2:1
PO:15=2:1
PO=30
PA=PO+OP
PA=30+15
PA=45
PA is also the altitude using it side of the triangle can be calculated.
$\sqrt{3}*s/2$=45
s=$30\sqrt{3}$
Area of an equilateral triangle=$\sqrt{3}(s^{2})/4$
=$675\sqrt{3}$
16) Answer (C)
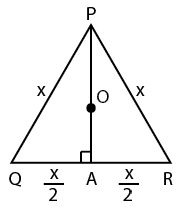
In an equilateral triangle,all the points such as orthocentre,centroid,circumcenter coincide.
Let the triangle be PQR and the circumcentre be O. let median intersect QR at A
Centroid divides median in the ratio 2:1
OA=5 cm
Therefore (PO:OA)=2:1
PO:5=2:1
PO=10
PA=PO+OP
PA=10+5
PA=15
PA is also the altitude using it side of the triangle can be calculated.
$\sqrt{3}*s/2$=15
s=$10\sqrt{3}$
Area of an equilateral triangle=$\sqrt{3}(s^{2})/4$
=$75\sqrt{3}$
17) Answer (C)
We know that,
Area of sector of a circle = $\frac{x}{360}*\pi *r^{2}$
Where $x$ is the angle subtended at the centre and $r$ is the radius of the circle
Area =$\frac{72}{360}\times\pi \times35^{2}$ = 770 sq.cm
18) Answer (D)
Let $l$ and $b$ be the initial values of length and breadth respectively.
Therefore, the final values of length and breadth are $1.3l and 1.25b$ respectively.
Initial area of the rectangle = $lb$
Final area of the rectangle = $1.3\times1.25 lb = 1.625 lb$
Therefore, required percentage = $\large\frac{(1.625-1)lb}{lb}\times$100 = 62.5%.
So, the correct option to choose is D.
19) Answer (B)
Let the radius of the circle formed be ‘r’
The length of the wire will be the circumference of the circle.
So we get,
$2\pi r=704$
$=>2\times\frac{22}{7}\times r=704$
$r=112$ cm
Thus the area will be = $\pi r^2=256\times154$.
=$39424 cm^2$
=$3.9424 m^2$. [$1m^2 = 10000cm^2$]
20) Answer (C)
The perimeter of the triangle is given as 36 cm.
The triangle of a fixed perimeter will have the maximum possible area when all the sides are of equal length.
So each side should be of length 12 cm for the triangle to have maximum possible area(i.e. Equilateral triangle).
In this case the area will be,
$\frac{\sqrt{3}}{4}\times a^2$
$\frac{\sqrt{3}}{4}\times12\times12$
$=36\sqrt{3}$ $cm^2$
DOWNLOAD APP FOR SSC FREE MOCKS
We hope this Problems on Mensuration questions for SSC Exam will be highly useful for your preparation.





