Selection Criteria Questions for MAH-CET PDF
Here you can download the important MAH – CET Selection Criteria Questions PDF by Cracku. Very Important MAH – CET 2022 and These questions will help your MAH – CET preparation. So kindly download the PDF for reference and do more practice.
Download Selection Criteria Questions for MAH-CET PDF
Enroll to MAH-CET Crash Course
Instructions
DIRECTIONS for the following three questions: Answer the questions on the basis of the information given below.

Rang Barsey Paint Company (RBPC) is in the business of manufacturing paints. RBPC buys RED, YELLOW, WHITE, ORANGE, and PINK paints. ORANGE paint can be also produced by mixing RED and YELLOW paints in equal proportions. Similarly, PINK paint can also be produced by mixing equal amounts of RED and WHITE paints. Among other paints, RBPC sells CREAM paint, (formed by mixing WHITE and YELLOW in the ratio 70:30) AVOCADO paint (formed by mixing equal amounts of ORANGE and PINK paint) and WASHEDORANGE paint (formed by mixing equal amounts of ORANGE and WHITE paint). The following table provides the price at which RBPC buys paints.
Question 1: The cheapest way to manufacture AVOCADO paint would cost
a) Rs. 19.50 per litre.
b) Rs. 19.75 per litre
c) Rs. 20.00 per litre.
d) Rs. 20.25 per litre.
1) Answer (B)
Solution:
AVOCADO paint can be manufactured by adding orange and pink in equal quantity. 0.5 ltr of orange would cost 11 and the cheapest way to make pink would be by mixing white and red , so the cost for 0.5 ltr of pink comes out to be 8.75 . SO total cost becomes 11+8.75 = 19.75 Rs. which is the cheapest.
Question 2: WASHEDORANGE can be manufactured by mixing
a) CREAM and RED in the ratio 14:10.
b) CREAM and RED in the ratio 3:1.
c) YELLOW and PINK in the ratio 1:1.
d) RED, YELLOW, and WHITE in the ratio 1:1:2.
2) Answer (D)
Solution:
WASHEDORANGE can be made by mixing orange and white , orange can be made by mixing equal quantities red and yellow which would be 1/2 of white quantity. Thus RED, YELLOW, and WHITE in the ratio 1:1:2 are needed.
Question 3: Assume that AVOCADO, CREAM and WASHEDORANGE each sells for the same price. Which of the three is the most profitable to manufacture?
a) AVOCADO
b) CREAM
c) WASHEDORANGE
d) Sufficient data is not available.
3) Answer (B)
Solution:
Cream would be undoubtedly the most profitable as maximum amount of white paint is used in it and white is the cheapest out of all other paints. Hence option B.
Instructions
Directions for the following four questions: Answer the questions on the basis of the information given below.
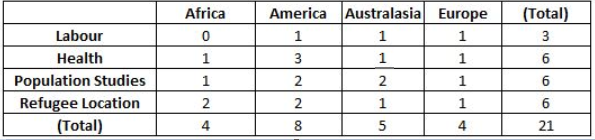
Twenty one participants from four continents (Africa, Americas, Australasia, and Europe) attended a United Nations conference. Each participant was an expert in one of four fields, labour, health, population studies, and refugee relocation. The following five facts about the participants are given.
(a) The number of labour experts in the camp was exactly half the number of experts in each of the three other categories.
(b) Africa did not send any labour expert. Otherwise, every continent, including Africa, sent at least one expert for each category.
(c) None of the continents sent more than three experts in any category.
(d) If there had been one less Australasian expert, then the Americas would have had twice as many experts as each of the other continents.
(e) Mike and Alfanso are leading experts of population studies who attended the conference. They are from Australasia.
Question 4: Which of the following combinations is NOT possible?
a) 2 experts in population studies from the Americas and 2 health experts from Africa attended the conference.
b) 2 experts in population studies from the Americas and 1 health expert from Africa attended the conference.
c) 3 experts in refugee relocation from the Americas and 1 health expert from Africa attended the conference.
d) Africa and America each had 1 expert in population studies attending the conference.
4) Answer (D)
Solution:
According to given conditions, the possible solutions are as given in the tables below:
From the above tables we can see that there was no possible case in which Africa and America had 1 expert each in population studies. Thus, statement D is false.
Question 5: If Ramos is the lone American expert in population studies, which of the following is NOT true about the numbers of experts in the conference from the four continents?
a) There is one expert in health from Africa.
b) There is one expert in refugee relocation from Africa.
c) There are two experts in health from the Americas.
d) There are three experts in refugee relocation from the Americas.
5) Answer (C)
Solution:
From the first condition a, assume the number of experts in labour = x, then the number of experts in each of the other three categories =2x
Now, x+2x+2x+2x+2x = 21 => x = 3 and 2x = 6
So the number of experts in labour = 3 and the number of experts in each of the three categories = 6
From condition d, if the number of experts of Astralaisa = y, then the number of experts of Americas = 2(y-1), the number of experts of Europe = y-1, the number of experts of Africa= y-1
Now, y+y-1+y-1+2(y-1)=21 => 5y-4=21 => y=5
Hence the table can be filled as follows:

Using condition b, the number of labour experts of Africa = 0 and the number of labour experts of the rest of the continents will be 1 each.
Consider the column of Europe, since Europe sent at least 1 in each category, each category will have 1 expert. (Since the total sum is 4)
From the condition e, the population studies of Australasia will contain 2 experts. So all the other categories will have to take at 1 expert because the total sum is 5 and no value can be 0. The table can be filled as follows:

Now, according to the condition c, the possible solutions are as given in the tables below:



From the tables we can see that when there is only 1 population expert from America, there are 3 American Health Experts. Hence option C is not true.
Take Free MAH-CET mock tests here
Question 6: Alex, an American expert in refugee relocation, was the first keynote speaker in the conference. What can be inferred about the number of American experts in refugee relocation in the conference, excluding Alex?
i. At least one
ii. At most two
a) Only i and not ii
b) Only ii and not I
c) Both i and ii
d) Neither i nor ii
6) Answer (C)
Solution:
According to given conditions, the possible solutions are as given in the tables below:



If an American expert in refugee relocation, was the first keynote speaker in the conference. Then apart from him there is atleast 1 and atmost 2 american expert in refugee relocation. Hence option C.
Question 7: Which of the following numbers cannot be determined from the information given?
a) Number of labour experts from the Americas.
b) Number of health experts from Europe.
c) Number of health experts from Australasia.
d) Number of experts in refugee relocation from Africa.
7) Answer (D)
Solution:
According to given conditions, the possible solutions are as given in the tables below:



We can see that there are 2 possibilities for number of experts in refugee relocation from Africa.Hence it cannot be determined.
Instructions
Two traders, Chetan and Michael, were involved in the buying and selling Of MCS shares over five trading days. At the beginning of the first day, the MCS share was priced at Rs 100, while at the end of the fifth day it was priced at Rs 110. At the end of each day, the MCS share price either went up by Rs 10, or else, it came down by Rs 10. Both Chetan and Michael took buying and selling decisions at the end of each trading day. The beginning price of MCS share on a given day was the same as the ending price of the previous day. Chetan and Michael started with the same number of shares and amount of cash, and had enough of both. Below are some additional facts about how Chetan and Michael traded over the five trading days.
• Each day if the price went up, Chetan sold 10 shares of MCS at the closing price. On the other hand, each day if the price went down, he bought 10 shares at the closing price.
• If on any day, the closing price was above Rs 110, then Michael sold 10 shares of MCS, while if it was below Rs 90, he bought 10 shares, all at the closing price.
Question 8: If Chetan sold 10 shares of MCS on three consecutive days, while Michael sold 10 shares only once during the five days, what was the price of MCS at the end of day 3?
a) Rs 90
b) Rs 100
c) Rs 110
d) Rs 120
e) Rs 130
8) Answer (C)
Solution:

The above table includes the values of the share price at the end of each day. Chetan and Michael column shows the number of shares at the end of 5th day with Chetan and Michael respectively. (-10 means he has sold 10 shares, +10 means he has bought 10 shares)
there are 10 different possible cases according to the initial and final share price.
The question asks about the case where Chetan has sold 3 times and Michael sells only once.
Starting with Michael, for exactly one sell, the price should touch 120 only once as Michael sells the share only at price greater than 110.
if the price touches 120 twice or more, Michael will sell the share more than once which is not a desirable case.
Also, chetan has to sell thrice consecutively which is only possible if the share price is 90 at one instance and rises to 120 in straight 3 days.
This is only possible in case 2. Hence the price on 3rd day’s end in case 2 is 110.
Question 9: If Michael ended up with Rs 100 less cash than Chetan at the end of day 5, what was the difference in the number of shares possessed by Michael and Chetan (at the end of day 5)?
a) Michael had 10 less shares than Chetan.
b) Michael had 10 more shares than Chetan.
c) Chetan had 10 more shares than Michael.
d) Chetan had 20 more shares than Michael.
e) Both had the same number of shares.
9) Answer (E)
Solution:

The above table includes the values of the share price at the end of each day. Chetan and Michael column shows the number of shares at the end of 5th day with Chetan and Michael respectively. (-10 means he has sold 10 shares, +10 means he has bought 10 shares)
there are 10 different possible cases according to the initial and final share price.
Please note that Chetan will always be having -10 shares(10 shares sold) and 1300 as cash.
This is because Chetan buys for every fall in price and sells for every rise in price. But the fluctuation in share price is constant as it starts from 100 and closes at 110 on day 5. So, in total chetan will always sell 10 shares in all 5 days combined.
As chetan has sold 10 shares , he’ll get 110*10 =1100 cash because of it in every case. Also chetan earns Rs. 200 in every case because of buying at low and selling at high. So total cash chetan will always have after 5 days = 1100+200 =1300.
The question asks about the case where Michael ended up with Rs 100 less cash than Chetan at the end of day 5.
So we have to look for the case where Michael has 1200 cash which is only possible when Michael has -10 number of shares in the end of day 5 and also has made profit of Rs. 100.
This is possible in case 7 as Michael has sold the shares at rs 120 but did not sell those shares as price never went below 90.
In the end Michael will have -10 shares at a price 110 which is Rs. 10 less than the price he sold at.
So he makes profit of 100 rs through it and will have the cash of 1100 through the sold shares.
Their difference in 7th case is 1300-1200=100
Also, in this case they have same number of shares sold at the end of the 5th day. So E is the answer.
Question 10: If Chetan ended up with Rs 1300 more cash than Michael at the end of day 5, what was the price of MCS share at the end of day 4?
a) Rs 90
b) Rs 100
c) Rs 110
d) Rs 120
e) Not uniquely determinable
10) Answer (B)
Solution:

The above table includes the values of the share price at the end of each day. Chetan and Michael column shows the number of shares at the end of 5th day with Chetan and Michael respectively. (-10 means he has sold 10 shares, +10 means he has bought 10 shares)
there are 10 different possible cases according to the initial and final share price.
Please note that Chetan will always be having -10 shares(10 shares sold) and 1300 as cash.
This is because Chetan buys for every fall in price and sells for every rise in price. But the fluctuation in share price is constant as it starts from 100 and closes at 110 on day 5. So, in total Chetan will always sell 10 shares in all 5 days combined.
As Chetan has sold 10 shares, he’ll get 110*10 =1100 cash because of it in every case. Also, Chetan earns Rs. 200 in every case because of buying at low and selling at high. So total cash Chetan will always have after 5 days = 1100+200 =1300.
The question asks about the case where Michael ended up with Rs 1300 cash less than Chetan.
As Chetan will have exactly 1300 cash in every case; we have to look for the cases where Michael does not make any profit and also does not have any sold shares at the end of 5 days.
This is possible in 4 cases ie. 3,4,5 and 9.
In each of these cases, the price fluctuates in the range 90-110 which does not allow Michael to buy or sell any shares.
Also, in all these cases the price of MCS is 100 at the end of day 4.
So the answer is 100.
Question 11: What could have been the maximum possible increase in combined cash balance of Chetan and Michael at the end of the fifth day?
a) Rs 3700
b) Rs 4000
c) Rs 4700
d) Rs 5000
e) Rs 6000
11) Answer (D)
Solution:

The above table includes the values of the share price at the end of each day. Chetan and Michael column shows the number of shares at the end of 5th day with Chetan and Michael respectively. (-10 means he has sold 10 shares, +10 means he has bought 10 shares)
there are 10 different possible cases according to the initial and final share price.
Please note that Chetan will always be having -10 shares(10 shares sold) and 1300 as cash.
This is because Chetan buys for every fall in price and sells for every rise in price. But the fluctuation in share price is constant as it starts from 100 and closes at 110 on day 5. So, in total Chetan will always sell 10 shares in all 5 days combined.
As Chetan has sold 10 shares, he’ll get 110*10 =1100 cash because of it in every case. Also, Chetan earns Rs. 200 in every case because of buying at low and selling at high. So total cash Chetan will always have after 5 days = 1100+200 =1300.
The question asks about the case where there has been the maximum possible increase in the combined cash balance of Chetan and Michael at the end of the fifth day.
As we know chetan has 1300 cash in all the cases , so we have to maximize the case where Michael has the most cash.
Also, if we see clearly, the profit made by selling and buying is in hundreds while the cash received by selling the shares is much far in terms of cash.
As 10 shares sold give = 10*110 = 1100 cash. So we have to look at the case where Michael has sold most shares which is case 8.
In case 8 the price rises from 100 to 110 ->120 ->130 ->120 ->110
in this case 110(0 new shares)->120(Michael sold 10 shares)->130(Michael sold 10 shares)120(Michael sold 10 shares)->110(Michael does nothing) = 30 shares sold in total
Cash = 20 shares at 120 and 10 shares at 130 = 120*20+130*10 = 2400+1300 =3700
As Chetan has 1300 cash and Michael has 3700 cash;
total cash they have is : 3700+1300 =5000
Question 12: If Michael ended up with 20 more shares than Chetan at the end of day 5, what was the price of the share at the end of day 3?
a) Rs 90
b) Rs 100
c) Rs 110
d) Rs 120
e) Rs 130
12) Answer (A)
Solution:

The above table includes the values of the share price at the end of each day. Chetan and Michael column shows the number of shares at the end of 5th day with Chetan and Michael respectively. (-10 means he has sold 10 shares, +10 means he has bought 10 shares)
there are 10 different possible cases according to the initial and final share price.
Please note that Chetan will always be having -10 shares(10 shares sold) and 1300 as cash.
To have 20 more shares than Chetan, Michael has to buy 10 shares which is case 1.
In case 1, the share price at the end of day 3 is 90.
Instructions
K, L, M, N, P, Q, R, S, U and W are the only ten members in a department. There is a proposal to form a team from within the members of the department, subject to the following conditions:
- A team must include exactly one among P,R and S.
- A team must include either M or Q, but not both.
- If a team includes K, then it must also include L, and vice versa.
- If a team includes one among S, U and W, then it should also include the other two.
- L and N cannot be members of the same team.
- L and U cannot be members of the same team.
The size of a team is defined as the number of members in the team.
Question 13: What could be the size of a team that includes K?
a) 2 or 3
b) 2 or 4
c) 3 or 4
d) Only 2
e) Only 4
13) Answer (E)
Solution:
A team which has K should have L also.
Since L is there in the team, N and U should not be there in the team. Since U is not there in the team, S and W should not be there in the team.
So, the team will have K, L, one of P and R and one of M or Q.
So, the team size will be 4.
Question 14: In how many ways a team can be constituted so that the team includes N?
a) 2
b) 3
c) 4
d) 5
e) 6
14) Answer (E)
Solution:
Since N is in the team, L and K cannot be in the team.
The team can have one of M and Q. So, 2 ways of selection.
If the team has S, then it should have U and W as well.
If the team has no S, then it should have one of P or R.
So, the number of ways of forming the team is 2*(1+2) = 6 ways
Question 15: What would be the size of the largest possible team?
a) 8
b) 7
c) 6
d) 5
e) cannot be determined
15) Answer (D)
Solution:
Out of P, R and S only 1 can be in the team. If S is there, U and W will also be there. So, P and R should not be in the team for its size to be maximum.
Out of M and Q, only 1 can be there.
If L is there in the team, N and U cannot be in the team.
If L is not there in the team, then K is also not there in the team but N and U can be in the team.
So, the maximum team size is 5 consisting of S, U, W, (M or Q), N.
Question 16: Who can be a member of a team of size 5?
a) K
b) L
c) M
d) P
e) R
16) Answer (C)
Solution:
Out of P, R and S only 1 can be in the team. If S is there, U and W will also be there. So, P and R should not be in the team for its size to be maximum.
Out of M and Q, only 1 can be there.
If L is there in the team, N and U cannot be in the team.
If L is not there in the team, then K is also not there in the team but N and U can be in the team.
So, the maximum team size is 5 consisting of S, U, W, (M or Q), N.
So, M can be a member of team size 5.
Question 17: Who cannot be a member of a team of size 3?
a) L
b) M
c) N
d) P
e) Q
17) Answer (A)
Solution:
If L is in the team, the team should include K also. The team should have one among P, R and S and one among M and Q.
So, the team size cannot be 3 if L is in the team.
Instructions
Venkat, a stockbroker, invested a part of his money in the stock of four companies — A, B, C and D. Each of these companies belonged to different industries, viz., Cement, Information Technology (IT), Auto, and Steel, in no particular order. At the time of investment, the price of each stock was Rs.100. Venkat purchased only one stock of each of these companies. He was expecting returns of 20%, 10%, 30%, and 40% from the stock of companies A, B, C and D, respectively. Returns are defined as the change in the value of the stock after one year, expressed as a percentage of the initial value. During the year, two of these companies announced extraordinarily good results. One of these two companies belonged to the Cement or the IT industry, while the other one belonged to either the Steel or the Auto industry. As a result, the returns on the stocks of these two companies were higher than the initially expected returns. For the company belonging to the Cement or the IT industry with extraordinarily good results, the returns were twice that of the initially expected returns. For the company belonging to the Steel or the Auto industry, the returns on announcement of extraordinarily good results were only one and a half times that of the initially expected returns. For the remaining two companies, which did not announce extraordinarily good results, the returns realized during the year were the same as initially expected.
Question 18: What is the minimum average return Venkat would have earned during the year?
a) 30%
b) 31.25%
c) 32.5%
d) Cannot be determined
18) Answer (A)
Solution:
Hence , minimum average return will be 30 %
Question 19: If Venkat earned a 35% return on average during the year, then which of these statements would necessarily be true?I. Company A belonged either to Auto or to Steel Industry.II. Company B did not announce extraordinarily good results.III. Company A announced extraordinarily good results.IV. Company D did not announce extraordinarily good results.
a) I and II only
b) II and III only
c) III and IV only
d) II and IV only
19) Answer (B)
Solution:
So to get an average return of 35%, A announced extraordinary results and B did not.
Hence, option B is the answer.
Question 20: If Venkat earned a 38.75% return on average during the year, then which of these statement(s) would necessarily be true?I. Company C belonged either to Auto or to Steel Industry.II. Company D belonged either to Auto or to Steel Industry.III. Company A announced extraordinarily good results.IV. Company B did not announce extraordinarily good results.
a) I and II only
b) II and III only
c) I and IV only
d) II and IV only
20) Answer (C)
Solution:
To give 38.75 % average return we can see that B didnt give extraordinary returns and C can be any of the auto/steel industry.