Mensuration Questions for MAH-CET | Download PDF

Here you can download important MAH – CET Mensuration Questions PDF by Cracku. Very Important MAH – CET 2022 and These questions will help your MAH – CET preparation. So kindly download the PDF for reference and do more practice.

Download Mensuration Questions for MAH-CET PDF

Enroll to MAH-CET Crash Course

Question 1: The edge of an ice cube is 14 cm. The volume of the largest cylindrical ice cube that can be fit into it?

a) 2200 cu. cm

b) 2000 cu. cm

c) 2156 cu. cm

d) 2400 cu. cm

e) None of these

Question 2: The edge of an ice cube is 14 cm. The volume of the largest cylindrical ice cube that can be formed out of it is

a) 2200 cu. cm

b) 2000 cu. cm

c) 2156 cu. cm

d) 2400 cu. cm

e) None of these

Question 3: A rope makes 125 rounds of a cylinder with base radius 15 cm. How many times can it go round a cylinder with base radius 25 cm?

a) 100

b) 75

c) 80

d) 65

e) None of these

Question 4: A right circular cylindrical tank has the storage capacity of 98808 ml. If the radius of the base of the cylinder is three-fourth of the height, what is the diameter of the base ?

a) 28 cms

b) 56 cms

c) 21 cms

d) 58 cms

e) None of these

Question 5: The respective ratio of curved surface area and total surface area of a cylinder is 4:5. If the curved surface area of the cylinder is 1232 cm2, what is the height ? (in cm)

a) 28 cm

b) 34 cm

c) 36 cm

d) 30 cm

e) None of these

Take Free MAH-CET mock tests here

Question 6: The sum of the radius and height of a cylinder is 18 metre. The total surface area of the cylinder is 792 sq. metre, what is the volume of the cylinder ? (in cubic metre)

a) 1848

b) 1440

c) 1716

d) 1724

e) 1694

Question 7: If the volume and curved surface area of a cylinder are 616 $m^3$ and 352 $m^2$ respectively what is the total surface area of the cylinder (in $m^2$)

a) 429

b) 419

c) 435

d) 421

e) 417

Question 8: The sum of the radius and height of a cylinder is 42 cm. Its total surface area is 3696 cm 2. What is the volume of cylinder ?

a) 17428 cubic cm

b) 17248 cubic cm

c) 17244 cubic cm

d) 17444 cubic cm

e) None of these

Instructions

Read the following information and answer the questions based on it.
The length ,breadth and height of a rectangular piece of wood in the 4cm,3cm, 5cm respectively
Opposite side of 5cm x 4 cm pieces are coloured in red colour
Oppsite sides 4cm x 3 cm ,are cloured in blue
Rest 5 cm x 3 cm are coloured in green in both sides
Now the piece is cut in such way that a cuboid of 1cm x 1cm x 1cm will be made

Question 9: How many cuboids shall have only one colour ?

a) 12

b) 16

c) 22

d) 28

e) None of these

Question 10: How many cuboids shall have only two colours red and green in their two sides?

a) 8

b) 12

c) 16

d) 20

e) None of these

Question 11: How many cuboids shall not any colour?

a) No any

b) 2

c) 4

d) 6

e) None of these

Question 12: How many cuboids shall have all the three colours?

a) 8

b) 10

c) 12

d) 14

e) None of these

Question 13: The six faces of a wooden cube of side 6 cm are labelled A, B, C, D, E and F respectively. Three of these faces A, B, and C are each adjacent to the other two, and are painted red. The other three faces are not painted. Then, the wooden cube is neatly cut into 216 little cubes of equal size. How many of the little cubes have no sides painted?

a) 125

b) 135

c) 91

d) 108

e) 100

Question 14: A colourless cube is painted blue and then cut parallel to sides to form two rectangular solids of equal volume. What percentage of surface area of each of new solids is not painted blue?

a) 25

b) 16

c) 20

d) 18

Question 15: The intersection of two cubes cannot be

a) cube

b) triangle

c) rectangle

d) none of these

Question 16: The cost of the paint is 36.50 per kg. If 1 kg of paint covers 16 square feet, how much will it cost to paint outside of a cube having 8 feet each side?

a) 692

b) 768

c) 876

d) 972

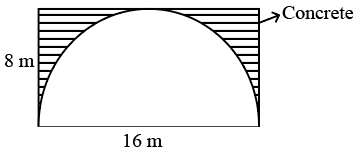
Question 17: Consider a cuboidal underground tunnel of length 500 m whose cross-section is given in the figure. If $1 m^3$ of concrete costs 1000 rupees, find the amount of money needed to build the Tunnel.

a) $8(4-\pi)10^6 $ rupees

b) $64(4-\pi)10^6 $ rupees

c) $16(4-\pi)10^6 $ rupees

d) $32(4-\pi)10^6 $ rupees

Question 18: The length, breadth and height of a rectangular cuboid are in the ratio 1: 2 : 3. If the length, breadth and height are increased by 100% each then what would be the increase in the volume of the cuboid ?

a) 3 times

b) 7 times

c) 10 times

d) 9 times

Question 19: Ram, a farmer, managed to grow shaped- watermelons inside glass cases of different shapes. The shapes he used were: a perfect cube, hemi-spherical, cuboid, cylindrical along with the normal spherical shaped watermelons. Thickness of the skin was same for all the shapes. Each of the glass cases was so designed that the total volume and the weight of the all the water- melons would be equal irrespective of the shape.
A customer wants to but water-melons for making juice, for which the skin of the water- melon has to be peeled off, and therefore is a waste. Which shape should the customer buy?

a) Cube

b) Hemi-sphere

c) Cuboid

d) Cylinder

e) Normal spherical

Question 20: A cube of side 12 cm is painted red on all the faces and then cut into smaller cubes, each of side 3 cm. What is the total number of smaller cubes having none of their faces painted?

a) 16

b) 8

c) 12

d) 24

Get 5 MAH-CET mocks at just Rs.299

Answers & Solutions:

1) Answer (C)

Radius of the cylinder = r = $\frac{14}{2}$ = 7
Height of the cylinder = h =14
Volume = Pi x r² x h
= $\frac{22}{7}$ x 7 x 7 x 14 = 2156

2) Answer (C)

Radius of the cylinder = r = $\frac{14}{2}$ = 7

Height of the cylinder = h =14

Volume = Pi x r² x h

= $\frac{22}{7}$ x 7 x 7 x 14 = 2156

3) Answer (B)

Let the required number of rounds be x.

More radius, less rounds (Inverse Proportion)

Radius in cm ==  Round

15 == 125

25 == x

⟹ x=(15 * 125)/25 = 75 rounds

4) Answer (D)

Volume if cylinder = π$(R)^2$h

R is the radius and h is the height of cylinder

Volume = 98808 ml

It is given that R = $\frac{3}{4}$h

$\frac{4}{3}$ $(R)^3$ π = 98808

R = 28.68

Diameter = 28.68 × 2 ~58

5) Answer (A)

Let R and H be the radius and height of cylinder

Curved Surface area of cylinder = 2$\barwedge$RH = 1232

($\barwedge$RH= 616 sqr cm)

Total surface area of cylinder = 2$\barwedge$RH + 2$\barwedge(R)^2$ = 2$\barwedge$R(H+R)

it is given that CurvedSurfaceArea/TotalSurfaceArea = 4/5

1232/(1232 + 2$\barwedge(R)^2$) = 4/5

R = 7 cm

2$\barwedge$RH = 1232

2$\barwedge$7H = 1232

44H =1232
H = 28 cm

 

 

6) Answer (E)

let the height and radius of cylinder be H mtr and R mtr

R + H = 18

total surface area of cylinder = 2$\barwedge$RH + 2$\barwedge(R)^2$ = 792

R(H + R) = $\frac{792×7}{22×2}$

R = 7 mtr

H = 18-7 = 11 mtr

volume = $\frac{22}{7}(R)^2(H)$

Volume = 1694 cubic mtr

7) Answer (A)

Volume of a cylinder=$\pi \times r^{2} \times h$
where $r$ and $h$ are the radius and height of the cylinder.
$\pi \times r^{2} \times h$ = $616 m^{3}$
Curved Surface Area of Cylinder=$2\times \pi \times r \times h$=$352 m^{2}$
$\pi \times r \times h$=$176$
Replacing $\pi \times r \times h$ in Volume formula we get,
$ r \times 176$=$616$
$r=3.5 m$
Total Surface Area = Curved Surface Area + 2$\times$ Area of base
=$352 + 2\times pi \times r^{2}$
=$352 + 2\times pi \times 3.5^{2}$
=$352+77$
=$429 m^{2}.$
Hence Option A is the correct answer.

 

 

 

 

8) Answer (B)

Total surface area of cylinder

=> $2 \pi r h + 2 \pi r^2 = 3696$

=> $2 \pi r (r + h) = 3696$

$\because (r + h) = 42$   [Given]

=> $2 \times \frac{22}{7} \times r \times 42 = 3696$

=> $44 \times 6 \times r = 3696$

=> $r = \frac{3696}{44 \times 6} = 14$ cm

=> $h = 42 – 14 = 28$ cm

$\therefore$ Volume of cylinder = $\pi r^2 h$

= $\frac{22}{7} \times 14 \times 14 \times 28$

= $17248 cm^3$

9) Answer (E)

Number of cuboids which have only 1 colour are the middle cuboids in all the faces. Also, there are 2 types of each faces.

2*(B-2)*(H-2)+2*(H-2)*(L-2).

=2*(4-2)*(3-2)+2*(3-2)*(5-2)+2*(5-2)*(4-2).

= 2*2*1 + 2*1*3 + 2*3*2.= 4 + 6 + 12.

=22.

10) Answer (B)

Number of cuboids which have only two colours red and green in their two sides are the middle cuboids at the corner edges. There are 4 such edges which have combination of red and green colour.

Number of required cuboids = $(5-2) \times 4$

= $3 \times 4=12$

=> Ans – (B)

11) Answer (D)

Number of cuboids which do not have any colour = $(5-2) \times (4-2) \times (3-2)$

= $3 \times 2 \times 1=6$

=> Ans – (D)

12) Answer (A)

The number of cuboid which will have all the three colours are the corner pieces.

Thus, 8 cuboids will have all the three colours.

=> Ans – (A)

13) Answer (A)

Since A, B and C are adjacent faces. If we remove them, the resultant solid will also be a cube with side 5.

Hence total number of cubes unpainted = $5^3$ = 125

14) Answer (A)

Assuming the sides of the cube = a, then surface painted blue = 6a$^2$

Now after cutting into half the blue surface = 3a$^2$ for each solid and a new colourless surface with area a$^2$ is generated.

Hence the total surface area  = 3a$^2$ + a$^2$ = 4a$^2$

% surface area not painted blue = $\ \dfrac{\ a^2}{4a^2}\times\ 100$ = 25

15) Answer (A)

Cubes are a 3-D figure, and so, the intersection of two 3-D figure must be a 2-D figure.

So, Cube cannot be the intersection of two cubes.

16) Answer (C)

Total surface area of cube = $6\times\ 8^2\ =384$

Amount of paint required = $\ \frac{\ 384}{16}=24\ kgs$

Cost of 24kgs paint = $24\times\ 36.5\ =876$

17) Answer (C)

Area of the tunnel to be filled with concrete = 16*8-$\pi\ \left(32\right)$

Length of the length along which the tunnel has to be filled with concrete = 500m

Volume = 16*8-$\pi\ \left(32\right)$*500

= $32(4-\pi)$*500

Cost of concrete per $1 m^3$ = 1000

Cost of $32(4-\pi)$*500 = $32(4-\pi)$*500*1000

=$16(4-\pi)*10^{6}$

C is the correct answer.

18) Answer (B)

Given the length, breadth and height of a rectangular cuboid are in the ratio 1: 2 : 3.

Let the length, breadth and height be k, 2k, and 3k units where ‘k’ is a constant.

Volume of the cuboid(V’) = $l\times b\times h$ units = $k\times 2k\times 3k$  = $6k^3$ units

Given the length, breadth and height are increased by 100% each .

Then the new length, breadth and height will be doubled.

So new length, breadth and height of the rectangular cuboid are 2k, 4k, and 6k units.

New Volume of the cuboid (V’) = $2k\times 4k\times 6k = 48k^3$ units.

Increase in the volume of the cuboid = V’ – V = $48k^3 – 6k^3 = 42k^3 = 7 [6k^3] = 7V$

Therefore the volume has increased 7 times the initial volume.

19) Answer (E)

Let the volume of watermelon = $V$

Total surface area = $S$

Thickness of the skin = $t$

=> Volume usable for juice = $V – S t$

Hence, if total surface area is minimum then usable volume of the watermelon will be max.

For equal volume, sphere has the least surface area.

Ans – (E)

20) Answer (B)

Total number of cubes will be = $\frac{12 \times 12 \times 12}{3 \times 3 \times 3}$ = 64
Cubes with 3 sides painted = cubes at corner sides = 8
Cubes with 2 sides painted = cubes on lines = 24
Cubes with 1 sides painted = cubes on middle of surface = 24
So cubes with no side painted = 64 – (8+24+24) = 8

Take MAH-CET Mock Tests

Enroll to CAT 2022 course