Average and Interest Questions for SSC CHSL and MTS

Here you can download the Average and Interest Questions for SSC CHSL and MTS PDF with solutions by Cracku. These are the most important Average and Interest questions PDF prepared by various sources also based on previous year’s papers. Utilize this PDF for Average and Interest for SSC CHSL and MTS. You can find a list of Average and Interests in this PDF which help you to test yourself and practice. So you can click on the below link to download the PDF for reference and do more practice.

Download Average and Interest Questions for SSC CHSL and MTS

Enroll to 15 SSC CHSL 2022 Mocks At Just Rs. 149

Question 1: What is the difference (in ₹) between the compound interest, when interest is compounded 6-monthly, and the simple interest on a sum of ₹10,000 for $1\frac{1}{2}$ years at 10% p.a.?

a) 102.25

b) 87

c) 76.25

d) 91.5

1) Answer (C)

Solution:

If interest is half-yearly, Then

Time = $1.5\times\ 2=3$

Rate = $\frac{10}{2}=\ 5\%$

$A=P\left(1+\frac{r}{100}\right)^n$

= $A=10,000\left(1+\frac{5}{100}\right)^3$

$A=10,000\ \times\ \frac{21}{20}\times\ \frac{21}{20}\times\ \frac{21}{20}=11576.25$

C.I = A – P = 11576.25 – 10000 = ₹1576.35

Simple Interest = $S.I\ =\frac{\left(P\times\ R\times\ T\right)}{100}$

= $\frac{\left(10000\times\ 10\times\ 1.5\right)}{100}$

= ₹1500

Required Difference = ₹1576.25 – ₹1500 = ₹76.25

Hence, Option C is correct.

Question 2: A sum of ₹25600 is invested on simple interest partly at 7% per annum and the remaining at 9% per annum. The total interest at the end of 3 years is ₹5832. How much money(in ₹) was invested at 9% per annum?

a) 18000

b) 7600

c) 9600

d) 16000

2) Answer (B)

Solution:

Let the amount invested in 9% per annum = P

Amount invested in 7% per annum = 25600 – P

The total interest at the end of 3 years is ₹5832.

P x 3 x $\frac{9}{100}$ + (25600 – P) x 3 x $\frac{7}{100}$ = 5832

$\frac{9}{100}$P + 1792 – $\frac{7}{100}$P = 1944

$\frac{2}{100}$P = 152

P = ₹7600

$\therefore$  Amount invested at 9% per annum = P = ₹7600

Hence, the correct answer is Option B

Question 3: Two equal sums were lent on simple interest at 6% and 10% per annum respectively. The first sum was recovered two years later than the second sum and the amount in each case was ₹1105. What was the sum (in ₹) lent in each scheme?

a) 900

b) 850

c) 936

d) 891

3) Answer (B)

Solution:

Let the sum lent in each scheme = P

Let the sum at 6% and 10% simple interest was recovered after ‘t+2’ years and ‘t’ years respectively.

Amount obtained from simple interest at 6% = P + $\frac{P\times(t+2)\times6}{100}$

1105 = P + $\frac{P\times(t+2)\times6}{100}$

1105 – P = $\frac{P\times(t+2)\times6}{100}$…….(1)

Amount obtained from simple interest at 10% = P + $\frac{P\times t\times10}{100}$

1105 = P + $\frac{P\times t\times10}{100}$

1105 – P = $\frac{P\times t\times10}{100}$……..(2)

From (1) and (2),

$\frac{P\times(t+2)\times6}{100}$ = $\frac{P\times t\times10}{100}$

6t + 12 = 10t

t = 3

Substituting t = 3 in equation (2), we get P = 850

The sum lent in each scheme = ₹850

Hence, the correct answer is Option B

Question 4: A sum of ₹9500 amounts to ₹11495 in 2 years at a certain rate percent per annum, interest compounded yearly. What is the simple interest (in ₹) on the same sum for the same time and double the rate?

a) 3990

b) 3420

c) 4560

d) 3800

4) Answer (D)

Solution:

Let the rate of interest = R

11495 = 9500(1+$\frac{R}{100}$)$^2$

(1+$\frac{R}{100}$)$^2$ = $\frac{11495}{9500}$

(1+$\frac{R}{100}$)$^2$ = $\frac{121}{100}$

1+$\frac{R}{100}$ = $\frac{11}{10}$

$\frac{R}{100}$ = $\frac{1}{10}$

R = 10%

Simple interest = $\frac{9500\times2\times20}{100}$

= ₹3800

Hence, the correct answer is Option D

Question 5: A person borrowed a sum of ₹30800 at 10% p.a. for 3 years, interest compounded annually. At the end of two years, he paid a sum of ₹13268. At the end of 3rd year, he paid ₹ x to clear of the debt. What is the value of x ?

a) 26400

b) 26510

c) 26200

d) 26620

5) Answer (A)

Solution:

Amount to be paid after 2 years = 30800(1+$\frac{10}{100}$)$^2$

= 30800($\frac{11}{10}$)$^2$

= 30800($\frac{121}{100}$)

= ₹37268

Amount paid by the person = ₹13268

Remaining amount = ₹37268 – ₹13268 = ₹24000

Amount to be paid at the end of 3rd year to clear debt(i.e, compound interest on ₹24000 for next 1 year) = 24000(1+$\frac{10}{100}$)$^1$

$\Rightarrow$  x = 24000($\frac{11}{10}$)

$\Rightarrow$  x = ₹26400

Hence, the correct answer is Option A

Take a free SSC CHSL Tier-1 mock test

Download SSC CGL Tier-1 Previous Papers PDF

Question 6: At what rate percent per annum will ₹7200 amountto ₹7938 in one year, if interest is compounded half yearly?

a) 5

b) 8

c) 12

d) 10

6) Answer (D)

Solution:

Let the rate of interest per annum = R

According to the problem,

7938 = 7200(1+$\frac{\frac{R}{2}}{100}$)$^2$

3969 = 3600(1+$\frac{\frac{R}{2}}{100}$)$^2$

441 = 400(1+$\frac{\frac{R}{2}}{100}$)$^2$

(1+$\frac{\frac{R}{2}}{100}$)$^2$ = $\frac{441}{400}$

1+$\frac{\frac{R}{2}}{100}$ = $\frac{21}{20}$

$\frac{R}{200}$ = $\frac{1}{20}$

R = 10%

Hence, the correct answer is Option D

Question 7: A sum at simple interest becomes two times in 8 years at a certain rate of interest p.a. The time in which the same sum will be 4 times at the same rate of interest at simple interest is:

a) 25 years

b) 20 years

c) 30 years

d) 24 years

7) Answer (D)

Solution:

Let the principal amount = P

Rate of interest = R

Time = 8 years

Amount = 2P

$\Rightarrow$  2P = Principal amount + Simple Interest

$\Rightarrow$  2P = P + $\frac{\text{P}\times8\times \text{R}}{100}$

$\Rightarrow$  P =  $\frac{\text{P}\times8\times \text{R}}{100}$

$\Rightarrow$  R = 12.5%

Let the time in which sum will be 4 times at the same rate of interest = T

i.e, Amount = 4P

$\Rightarrow$  4P = P + $\frac{\text{P}\times \text{T}\times12.5}{100}$

$\Rightarrow$  3P = $\frac{\text{P}\times \text{T}\times12.5}{100}$

$\Rightarrow$  3 = $\frac{\text{T}}{8}$

$\Rightarrow$  T = 24 years

$\therefore\ $The time in which the same sum will be 4 times at the same rate of interest = 24 years

Hence, the correct answer is Option D

Question 8: Suresh lent out a sum of money to Rakesh for 5 years at simple interest. At the end of 5 years, Rakesh paid 9/8 of the sum to Suresh to clear out the amount. Find the rate of simple interest per annum.

a) 3.5% p.a.

b) 2.5% p.a.

c) 3% p.a.

d) 2% p.a.

8) Answer (B)

Solution:

Let the sum of money lent out by Suresh to Rakesh = P

Amount paid by Rakesh to Suresh after 5 years = $\frac{9}{8}$P

Let the rate of simple interest = R%

$\Rightarrow$  P + $\frac{\text{P}\times5\times \text{R}}{100}$ = $\frac{9}{8}$P

$\Rightarrow$  1 + $\frac{R}{20}$ = $\frac{9}{8}$

$\Rightarrow$  $\frac{R}{20}$ = $\frac{9}{8}-1$

$\Rightarrow$  $\frac{R}{20}$ = $\frac{1}{8}$

$\Rightarrow$  R = 2.5%

$\therefore\ $Rate of simple interest per annum = 2.5%

Hence, the correct answer is Option B

Question 9: If the difference between the compound interest and simple interest on a certain sum of money for three years at 10% p.a. is ₹ 558, then the sum is:

a) ₹ 18,500

b) ₹ 15,000

c) ₹ 16,000

d) ₹ 18,000

9) Answer (D)

Solution:

Let the principal sum = P

Rate = 10%

Time = 3 years

Compound interest on the sum = P$\left(1+\frac{10}{100}\right)^3-$ P = P$\left(\frac{110}{100}\right)^3-$ P = P$\frac{1331}{1000}-$ P = $\frac{331}{1000}$P

Simple interest on the sum = $\frac{P\times3\times10}{100}$ = $\frac{3}{10}$P

According to the problem,

$\frac{331}{1000}$P $-$ $\frac{3}{10}$P = 558

$\Rightarrow$  $\frac{331P-300P}{1000}$ = 558

$\Rightarrow$  $\frac{31P}{1000}$ = 558

$\Rightarrow$   P = 18000

$\therefore\ $The principal sum = ₹ 18,000

Hence, the correct answer is Option D

Question 10: The sum of simple interest on a sum at 8% p.a. for 4 years and 8 years is ₹960. The sum is:

a) ₹800

b) ₹1100

c) ₹1000

d) ₹900

10) Answer (C)

Solution:

Let the Principal amount = P

Rate = 8%

Given, the sum of simple interest on P for 4 years and 8 years is ₹960

$\Rightarrow$  $\frac{P\times4\times8}{100}+\frac{P\times8\times8}{100}=960$

$\Rightarrow$  $\frac{32P}{100}+\frac{64P}{100}=960$

$\Rightarrow$  $\frac{96P}{100}=960$

$\Rightarrow$   P = 1000

$\therefore\ $The required sum = ₹1000

Hence, the correct answer is Option C

Question 11: A sum of ₹50,000 is lent partly at 4% and remaining at 5% per annum. If the yearly simple interest on the average is 4.6%, the two parts are:

a) ₹22500, ₹27500

b) ₹15000, ₹35000

c) ₹20000, ₹30000

d) ₹25000, ₹25000

11) Answer (C)

Solution:

Let’s assume the amount lent partly at 4% is ₹’y’.

50,000 of 4.6% = y of 4% + (50,000-y) of 5%

$50,000\times4.6=y\times4+(50,000-y)\times5$

$230000=4y+250000-5y$

5y-4y = 250000-230000

y = 20000

So the two parts are ‘y’ and (50,000-y) = 20,000 and (50,000-y)

= 20,000 and (50,000-20,000)

= ₹20000 and ₹30000

Question 12: Table shows the annual Expenditure of a Company (in Lakh Rupees) over the years.

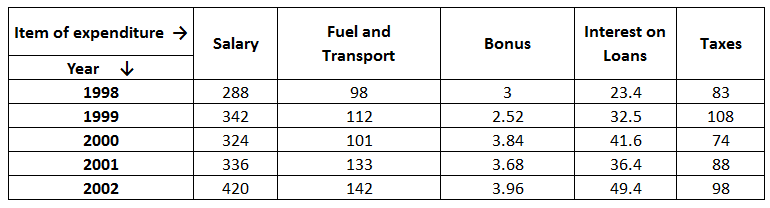
What is the average amount of Interest on loans (in Lakh rupees) which the company paid during the period 1998 to 2002?

a) 34.18

b) 33.72

c) 32.43

d) 36.66

12) Answer (D)

Solution:

As per the given data,

Total amount of interest on loans $=23.4+32.5+41.6+36.4+49.4=183.3$

Hence, average amount of interest on loans $\dfrac{183.3}{5}=36.7 =36.66$lakhs

Question 13: Asum of ₹ 10000 is lent partly at 8% and remaining at 10% per annum.If the yearly interest on the average is 9.2%, then both parts are respectively.

a) ₹5500 and ₹ 4500

b) ₹4500 and ₹ 5500

c) ₹5000 and ₹ 5000

d) ₹4000 and ₹ 6000

13) Answer (D)

Solution:

Let the amount lent at 8% be Rs.x
Then, Amount lent at 10% will be Rs.(10000-x)
Time period = 1 year

$\dfrac{x\times8}{100} + \dfrac{(10000-x)\times10}{100} = \dfrac{10000\times9.2}{100}$

=> $\dfrac{8x}{100}+\dfrac{100000-10x}{100} = 920$

=> $100000-2x = 92000$
=> $2x = 8000$
=> $x = 4000$
Therefore, The amounts are Rs.4000 and Rs.6000.

Question 14: Sachin lent out Rs 60000 in two parts, first at 4% and the second at 10% interest. The yearly average interest comes out to be 6.4%. What are the amounts (in Rs) that were lent at 4% and 10% respectively?

a) 40000,20000

b) 48000,12000

c) 36000,24000

d) 32000,28000

14) Answer (C)

Solution:

Let sum lent for 4% = Rs. $100x$ and sum lent for 10% = Rs. $(60,000-100x)$

Time period = 1 year and rate of interest = 6.4%

=> Simple interest = $\frac{P\times R\times T}{100}$

= $\frac{60,000\times6.4\times1}{100}=Rs.$ $3840$

According to ques,

=> $\frac{100x\times4\times1}{100}+\frac{(60000-100x)\times10\times1}{100}=3840$

=> $4x+(600-x)\times10=3840$

=> $4x+6000-10x=3840$

=> $6x=6000-3840=2160$

=> $x=\frac{2160}{6}=360$

$\therefore$ Amount lent at 4% = $100\times360=Rs.$ $36,000$

Amount lent at 10% = $(60,000-36,000)=Rs.$ $24,000$

=> Ans – (C)

Question 15: Puneet lent out Rs 30000 in two parts, one at 5% and the other at 9% interest. The yearly average interest comes out to be 6%. What are the amounts (in Rs) that are lent at 5% and 9% respectively?

a) 20000, 10000

b) 22500, 7500

c) 18000, 12000

d) 21000, 9000

15) Answer (B)

Solution:

Let sum lent for 5% = Rs. $100x$ and sum lent for 9% = Rs. $(30,000-100x)$

Time period = 1 year and rate of interest = 6%

=> Simple interest = $\frac{P\times R\times T}{100}$

= $\frac{30,000\times6\times1}{100}=Rs.$ $1800$

According to ques,

=> $\frac{100x\times5\times1}{100}+\frac{(30000-100x)\times9\times1}{100}=1800$

=> $5x+(300-x)\times9=1800$

=> $5x+(9\times300)-9x=(6\times300)$

=> $-4x=300(6-9)$

=> $x=\frac{300\times3}{4}=225$

$\therefore$ Amount lent at 5% = $100\times225=Rs.$ $22,500$

Amount lent at 9% = $(30,000-22,500)=Rs.$ $7,500$

=> Ans – (B)

Question 16: A fruit seller has a sale of ₹10,435, ₹9,927,₹10,855 ₹10,230 and ₹9,562 for five consecutive months.How much sale (in ₹) must he have in the sixth month so that he gets an average sale of ₹10,500?

a) 9,231

b) 8,231

c) 8,991

d) 11,991

16) Answer (D)

Solution:

As we know,

Average = $\frac{Sumofobservation}{total\ number\ of\ observation}$

Let the sale of 6th month be x

Average = $\frac{\left(10435+9927+10855+10230+9562+x\right)}{6}=10500$

= $51009\ +\ x=63000$

x = 11991

Hence, option D is correct.

Question 17: The average weight of 6 people increased by 2.5 kg when a new person came in place of other person weighing 55 kg. what can be the weight of new person (in kg)?

a) 70

b) 62.5

c) 60

d) 75.5

17) Answer (A)

Solution:

Total weight increased = number of persons $\times\ $ increased weight

i.e;$6\times\ 2.5=15$

Weight of new person = weight of replaced person + Total increased weight

i.e; 55 + 15 = 70 kg

Hence, Option A is correct.

Question 18: The average of eleven numbers is 56. The average of first three numbers is 52 and that of next five numbers is 60. The 9th and 10th number are 3 and 1 more than the 11th number respectively. What is the average of 9th and 11th numbers?

a) 53.5

b) 52

c) 52.5

d) 54

18) Answer (A)

Solution:

The average of first three numbers is 52.

Sum of the first three numbers = 52 x 3 = 156

The average of next five numbers is 60.

Sum of the next five numbers = 60 x 5 = 300

Let the 11th number be ‘n’.

The average of eleven numbers is 56.

Sum of the eleven numbers = 56 x 11 = 616

156 + 300 + (n + 3) + (n + 1) + n = 616

3n + 460 = 616

3n = 156

n = 52

Average of 9th and 11th numbers = $\frac{(n+3)+n}{2}$

= $\frac{107}{2}$

= 53.5

Hence, the correct answer is Option A

Question 19: The average of 23 numbers is 51. The average of first 12 numbers is 49 and the average of last 12 numbers is 54. If the twelfth number is removed, then the average of the remaining numbers (correct to two decimal places) is:

a) 53:25

b) 50.45

c) 51.75

d) 52.65

19) Answer (B)

Solution:

The average of 23 numbers is 51.

Sum of the 23 numbers = 51 x 23 = 1173…….(1)

The average of first 12 numbers is 49.

Sum of the first 12 numbers = 49 x 12 = 588

Sum of the first 11 numbers + 12th number = 588…..(2)

The average of last 12 numbers is 54.

Sum of the last 12 numbers = 54 x 12 = 648

12th number + Sum of the last 11 numbers = 648…..(3)

Adding (2) and (3),

Sum of the first 11 numbers + 12th number + 12th number + Sum of the last 11 numbers = 588 + 648

(Sum of the first 11 numbers + 12th number + Sum of the last 11 numbers) + 12th number = 1236

Sum of the 23 numbers + 12th number = 1236

1173 + 12th number = 1236

12th number = 63

Required average = $\frac{1173-63}{22}$

= 50.4545

Hence, the correct answer is Option B

Question 20: The average weight of a certain number of students in a class is 55.5 kg. If 4 students with average weight 60 kg join the class, then the average weight of all students in the class increases by 360 g. The number of students in the class, initially, is:

a) 46

b) 31

c) 41

d) 36

20) Answer (A)

Solution:

Let the initial number of students = n

Average of the weight of ‘n’ students = 55.55

Sum of the weight of ‘n’ students = 55.55n

Sum of the weight of 4 students = 60 x 4 = 240 kg

According to the problem,

$\frac{55.55n+240}{n+4}=55.55+\frac{360}{1000}$

$\frac{55.55n+240}{n+4}=55.55+0.36$

$\frac{55.55n+240}{n+4}=55.86$

55.55n + 240 = 55.86n + 223.44

0.36n = 16.56

n = 46

The initial number of students = n = 46

Hence, the correct answer is Option A

Download SSC Preparation App

Enroll to 15 SSC CHSL 2022 Mocks At Just Rs. 149