CAT Logical Reasoning Questions (LRDI) PDF [Most Important]
Logical Reasoning & Data Interpretation (LRDI) is one of the key three sections in the CAT exam. This section is a deciding factor for many MBA aspirants. It is essential that you solve more and more sets from the CAT Logical Reasoning section. Also, do check out all the Logical Reasoning sets from the CAT Previous Papers with detailed video solutions. This article will look into some important Logical Reasoning sets for the CAT Exam. If you want to practice these important CAT Logical Reasoning questions (LRDI) PDF, you can download the PDF given below, which is completely Free.
Download Logical Reasoning Questions for CAT
Enroll for CAT 2022 Crash Course
Instructions
A shopping mall has a large basement parking lot with parking slots painted in it along a single row. These slots are quite narrow; a compact car can fit in a single slot but an SUV requires two slots. When a car arrives, the parking attendant guides the car to the first available slot from the beginning of the row into which the car can fit.
For our purpose, cars are numbered according to the order in which they arrive at the lot. For example, the first car to arrive is given a number 1, the second a number 2, and so on. This numbering does not indicate whether a car is a compact or an SUV. The configuration of a parking lot is a sequence of the car numbers in each slot. Each single vacant slot is represented by letter V.
For instance, suppose cars numbered 1 through 5 arrive and park, where cars 1, 3 and 5 are compact cars and 2 and 4 are SUVs. At this point, the parking lot would be described by the sequence 1, 2, 3, 4, 5. If cars 2 and 5 now vacate their slots, the parking lot would now be described as 1, V, V, 3, 4. If a compact car (numbered 6) arrives subsequently followed by an SUV (numbered 7), the parking lot would be described by the sequence 1, 6, V, 3, 4, 7.
Answer the following questions INDEPENDENTLY of each other.
Question 1: Suppose that car 4 is not the first car to leave and that the sequence at a time between the arrival of the car 7 and car 8 is V, 7, 3, 6, 5. Then which of the following statements MUST be false?
a) Car 2 is a compact.
b) Car 7 is a compact.
c) Car 4 is an SUV.
d) Car 6 is a compact.
1) Answer (D)
Solution:
Here we can see that cars 3 and 5 are still in their position. Thus car 4 was not the first car to leave, either 1 or 2 left before 4. Let’s say only car 2 left before car 4. Now supposingly if car 2 is an SUV, car 6 was parked in that lot. Thus car 2 and car 7 are compact cars. Option 4 is correct.
Question 2: Suppose the sequence at some point of time is 4, 5, 6, V, 3. Which of the following is NOT necessarily true?
a) Car 4 is a compact.
b) Car 1 is an SUV.
c) Car 3 is an SUV
d) Car 5 is a compact.
2) Answer (C)
Solution:
The original sequence as given in the question is 4,5,6,V,3
This is possible when cars 1,2,3 arrived and then cars 1 and 2 leave. After that cars 4,5 and 6 arrive.
Now there are 4 slots to the left of car 3. This is only possible when cars 1 and 2 were SUVs. Now out of these 4 slots,
3 slots are occupied by cars 4,5 and 6. As a result these are compact cars . Car 3 can be a SUV or a Compact car and it won’t impact the final solution.
Question 3: Suppose eight cars have arrived, of which two have left. Also suppose that car 4 is a compact and car 7 is an SUV. Which of the following is a POSSIBLE current configuration of the parking lot?
a) 8, 2, 3, V, 6, 5, 7
b) V, 2, 3, 7, 5, 6, 8
c) 8, 2, 3, V, 5, 7, 6
d) 8, 2, 3, V, 5, 6, 7
3) Answer (D)
Solution:
Let’s look at option 4.
Order of cars is 8,2,3,V,5,6,7. This sequence is easily possible.
Let’s say cars 1,2,3,4,5,6,7 arrive one after the another.
Now Car 1 leaves and Car 8 takes that place.
Finally Car 4 leaves. Hence we can see that this combination of cars is possible
Question 4: Initially cars numbered 1, 2, 3, and 4 arrive among which 1 and 4 are SUVs while 2 and 3 are compact cars. Car 1 then leaves, followed by the arrivals of car 5 (a compact car) and car 6 (an SUV). Car 4 then leaves. Then car 7 (an SUV) and car 8 (a compact car) arrive. At this moment, which among the following numbered car is parked next to car 3?
a) 8
b) 5
c) 6
d) 7
4) Answer (D)
Solution:
following is the order of arrival and departure of cars
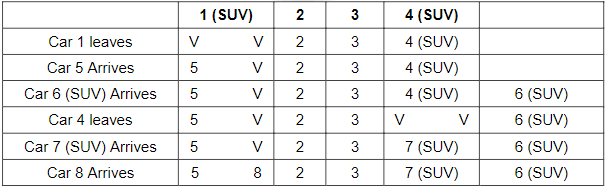
As we can see that car 2 and car 7 are parked next to car 3
Instructions
Twenty five coloured beads are to be arranged in a grid comprising of five rows and five columns. Each cell in the grid must contain exactly one bead. Each bead is coloured either Red, Blue or Green. While arranging the beads along any of the five rows or along any of the five columns, the rules given below are to be followed:
1. Two adjacent beads along the same row or column are always of different colours.
2. There is at least one Green bead between any two Blue beads along the same row or column.
3. There is at least one Blue and at least one Green bead between any two Red beads along the same row or column.
Every unique, complete arrangement of twenty five beads is called a configuration.
Question 5: Two Red beads have been placed in ‘second row, third column’ and ‘third row, second column’. How many more Red beads can be placed so as to maximise the number of Red beads used in the configuration?
5) Answer: 6
Solution:
6 more beads can be placed as shown

Checkout: CAT Free Practice Questions and Videos
Question 6: What is the minimum number of Blue beads in any configuration?
6) Answer: 6
Solution:
To solve this question we can use the answer of the previous question, since maximum 9 red beads are possible, filling the remaining space with green and blue beads, in such a way that number of blue beads is minimised

Hence number of blue beads is 6
Question 7: What is the maximum possible number of Red beads that can appear in any configuration?
7) Answer: 9
Solution:
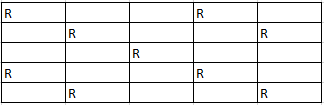
Maximum 9 red beads are possible as shown here
Question 8: The total number of possible configurations using beads of only two colours is:
8) Answer: 2
Solution:

There are only 2 configurations possible

Instructions
A high security research lab requires the researchers to set a pass key sequence based on the scan of the five fingers of their left hands. When an employee first joins the lab, her fingers are scanned in an order of her choice, and then when she wants to re-enter the facility, she has to scan the five fingers in the same sequence.
The lab authorities are considering some relaxations of the scan order requirements, since it is observed that some employees often get locked-out because they forget the sequence.
Question 9: The lab has decided to allow a variation in the sequence of scans of the five fingers so that at most two scans (out of five) are out of place. For example, if the original sequence is Thumb (T), index finger (I), middle finger (M), ring finger (R) and little finger (L) then TLMRI is also allowed, but TMRLI is not.
How many different sequences of scans are allowed for any given person’s original scan?
9) Answer: 11
Solution:
Let the original sequence be TIMRL
Two fingers can be out of place. This can be done if and only if two fingers interchange their position. These two can be selected in $^5C_2 = 10$ ways. In addition to these, the original sequence will also be accepted. Hence the total number of acceptable sequences = 10 + 1 = 11
Question 10: The lab has decided to allow variations of the original sequence so that input of the scanned sequence of five fingers is allowed to vary from the original sequence by one place for any of the fingers. Thus, for example, if TIMRL is the original sequence, then ITRML is also allowed, but LIMRT is not.
How many different sequences are allowed for any given person’s original scan?
a) 7
b) 5
c) 8
d) 13
10) Answer (C)
Solution:
Input of the scanned sequence of five fingers is allowed to vary from the original sequence by one place for any of the fingers. This can be achieved only when two consecutive fingers are interchanged. Let the original sequence be TIMRL
Case 1: Only a set of two consecutive numbers are interchanged.
They can be selected in 5-1 = 4 ways
Case 2: Two sets of two consecutive numbers are interchanged.
(i) TI are interchanged, => (MR, RL) => 2 ways
(ii) IM are interchanged => (RL) => 1 way
Total no of ways possible = 4 + 2 + 1 = 7
Including the original sequence, we get the total number of allowed combinations as 8
Question 11: The lab has now decided to require six scans in the pass key sequence, where exactly one finger is scanned twice, and the other fingers are scanned exactly once, which can be done in any order. For example, a possible sequence is TIMTRL.
Suppose the lab allows a variation of the original sequence (of six inputs) where at most two scans (out of six) are out of place, as long as the finger originally scanned twice is scanned twice and other fingers are scanned once.
How many different sequences of scans are allowed for any given person’s original scan?
11) Answer: 15
Solution:
There can be two scans out of place.
TIMTRL is the original sequence.
If T is interchanged: There will be four ways: ITMTRL, MITTRL, RIMTTL, LIMTRT
If I is interchanged: There will be four ways
If M is interchanged: There will be three ways
If T is interchanged: There will be two ways
If R is interchanged: There will be one way
Total 14.
Another sequence allowed is original, So total 15 ways.
Question 12: The lab has now decided to require six scans in the pass key sequence, where exactly one finger is scanned twice, and the other fingers are scanned exactly once, which can be done in any order. For example, a possible sequence is TIMTRL.
Suppose the lab allows a variation of the original sequence (of six inputs) so that input in the form of scanned sequence of six fingers is allowed to vary from the original sequence by one place for any of the fingers, as long as the finger originally scanned twice is scanned twice and other fingers are scanned once.
How many different sequences of scans are allowed if the original scan sequence is LRLTIM?
a) 8
b) 11
c) 13
d) 14
12) Answer (C)
Solution:
1. If original sequence is given.
2. If either of LR, RL, LT, TI, IM is interchanged => 5 ways.
3. If LR and LT and IM interchanged. The sequence will look like: RLTLMI
4. If LR and LT are interchanged.
5. If LR and TI are interchanged.
6. If LR and IM are interchanged.
7. If RL and TI are interchanged.
8. If RL and IM are interchanged.
9. If LT and IM are interchanged.
Total 13 ways possible.
Instructions
Adriana, Bandita, Chitra, and Daisy are four female students, and Amit, Barun, Chetan, and Deb are four male students. Each of them studies in one of three institutes – X, Y, and Z. Each student majors in one subject among Marketing, Operations, and Finance, and minors in a different one among these three subjects. The following facts are known about the eight students:
1. Three students are from X, three are from Y, and the remaining two students, both female, are from Z.
2. Both the male students from Y minor in Finance, while the female student from Y majors in Operations.
3. Only one male student majors in Operations, while three female students minor in Marketing.
4. One female and two male students major in Finance.
5. Adriana and Deb are from the same institute. Daisy and Amit are from the same institute.
6. Barun is from Y and majors in Operations. Chetan is from X and majors in Finance.
7. Daisy minors in Operations.
Question 13: Who are the students from the institute Z?
a) Chitra and Daisy
b) Adriana and Bandita
c) Bandita and Chitra
d) Adriana and Daisy
13) Answer (C)
Solution:
There are 8 students in total – 4 male and 4 female. There are 3 institutes X, Y, and Z.
3 students are from institute X, 3 students are from institute Y, and 2 students are from institute Z. No student majors and minors in the same subject.
It has been given that both the students from institute Z are female. Also, it has been given that both the male students from institute Y minor in Finance. Therefore, the third student from institute Y should be female. Institute X should also have 2 male and 1 female student.

Both the male students from Y minor in Finance, while the female student from Y majors in Operations. Barun is from Y and majors in Operations. Chetan is from X and majors in Finance.

It has been given that one female student and 2 male students major in finance. We know that the male student from Y minors in finance. Therefore, he cannot major in finance. Therefore, both the male students from X should major in finance.
Daisy and Amit are from the same institute. Therefore, Daisy cannot be from institute Z (since Amit is a male student and both the students from Z are female). Daisy minors in operations. The girl from institute Y majors in Operations. Therefore, Daisy cannot be from institute Y as well. Daisy and Amit should be from institute X. 3 female students minor in marketing. Therefore, all girls except Daisy should minor in marketing.

Adriana and Deb are from the same institute. Therefore, both of them should be from institute Y. Bandita and Chitra should be from institute Z.
Only one male student majors in Operations. We know that Barun is the student. Two male students major in Finance. We know that Amit and Chetan major in finance. Therefore, Deb should major in Marketing.

Bandita and Chitra are from institute Z. Therefore, option C is the right answer.
Question 14: Which subject does Deb minor in?
a) Operations
b) Finance
c) Marketing
d) Cannot be determined uniquely from the given information
14) Answer (B)
Solution:
There are 8 students in total – 4 male and 4 female. There are 3 institutes X, Y, and Z.
3 students are from institute X, 3 students are from institute Y, and 2 students are from institute Z. No student majors and minors in the same subject.
It has been given that both the students from institute Z are female. Also, it has been given that both the male students from institute Y minor in Finance. Therefore, the third student from institute Y should be female. Institute X should also have 2 male and 1 female student.

Both the male students from Y minor in Finance, while the female student from Y majors in Operations. Barun is from Y and majors in Operations. Chetan is from X and majors in Finance.

It has been given that one female student and 2 male students major in finance. We know that the male student from Y minors in finance. Therefore, he cannot major in finance. Therefore, both the male students from X should major in finance.
Daisy and Amit are from the same institute. Therefore, Daisy cannot be from institute Z (since Amit is a male student and both the students from Z are female). Daisy minors in operations. The girl from institute Y majors in Operations. Therefore, Daisy cannot be from institute Y as well. Daisy and Amit should be from institute X. 3 female students minor in marketing. Therefore, all girls except Daisy should minor in marketing.

Adriana and Deb are from the same institute. Therefore, both of them should be from institute Y. Bandita and Chitra should be from institute Z.
Only one male student majors in Operations. We know that Barun is the student. Two male students major in Finance. We know that Amit and Chetan major in finance. Therefore, Deb should major in Marketing.

Deb minors in Finance. Therefore, option B is the right answer.
Question 15: Which subject does Amit major in?
a) Marketing
b) Operations
c) Cannot be determined uniquely from the given information
d) Finance
15) Answer (D)
Solution:
There are 8 students in total – 4 male and 4 female. There are 3 institutes X, Y, and Z.
3 students are from institute X, 3 students are from institute Y, and 2 students are from institute Z. No student majors and minors in the same subject.
It has been given that both the students from institute Z are female. Also, it has been given that both the male students from institute Y minor in Finance. Therefore, the third student from institute Y should be female. Institute X should also have 2 male and 1 female student.

Both the male students from Y minor in Finance, while the female student from Y majors in Operations. Barun is from Y and majors in Operations. Chetan is from X and majors in Finance.

It has been given that one female student and 2 male students major in finance. We know that the male student from Y minors in finance. Therefore, he cannot major in finance. Therefore, both the male students from X should major in finance.
Daisy and Amit are from the same institute. Therefore, Daisy cannot be from institute Z (since Amit is a male student and both the students from Z are female). Daisy minors in operations. The girl from institute Y majors in Operations. Therefore, Daisy cannot be from institute Y as well. Daisy and Amit should be from institute X. 3 female students minor in marketing. Therefore, all girls except Daisy should minor in marketing.

Adriana and Deb are from the same institute. Therefore, both of them should be from institute Y. Bandita and Chitra should be from institute Z.
Only one male student majors in Operations. We know that Barun is the student. Two male students major in Finance. We know that Amit and Chetan major in finance. Therefore, Deb should major in Marketing.

Amit majors in finance. Therefore, option D is the right answer.
Question 16: If Chitra majors in Finance, which subject does Bandita major in?
a) Finance
b) Cannot be determined uniquely from the given information
c) Operations
d) Marketing
16) Answer (C)
Solution:
There are 8 students in total – 4 male and 4 female. There are 3 institutes X, Y, and Z.
3 students are from institute X, 3 students are from institute Y, and 2 students are from institute Z. No student majors and minors in the same subject.
It has been given that both the students from institute Z are female. Also, it has been given that both the male students from institute Y minor in Finance. Therefore, the third student from institute Y should be female. Institute X should also have 2 male and 1 female student.

Both the male students from Y minor in Finance, while the female student from Y majors in Operations. Barun is from Y and majors in Operations. Chetan is from X and majors in Finance.

It has been given that one female student and 2 male students major in finance. We know that the male student from Y minors in finance. Therefore, he cannot major in finance. Therefore, both the male students from X should major in finance.
Daisy and Amit are from the same institute. Therefore, Daisy cannot be from institute Z (since Amit is a male student and both the students from Z are female). Daisy minors in operations. The girl from institute Y majors in Operations. Therefore, Daisy cannot be from institute Y as well. Daisy and Amit should be from institute X. 3 female students minor in marketing. Therefore, all girls except Daisy should minor in marketing.

Adriana and Deb are from the same institute. Therefore, both of them should be from institute Y. Bandita and Chitra should be from institute Z.
Only one male student majors in Operations. We know that Barun is the student. Two male students major in Finance. We know that Amit and Chetan major in finance. Therefore, Deb should major in Marketing.

If Chitra majors in finance, Bandita cannot major in finance (only one female student majors in finance). She cannot major in marketing as well (since she has a minor degree in marketing). Therefore, Bandita should major in operations and hence, option C is the right answer.
Instructions
According to a coding scheme the sentence:
“Peacock is designated as the national bird of India” is coded as 5688999 35 1135556678 56 458 13666689 1334 79 13366
This coding scheme has the following rules:
a: The scheme is case-insensitive (does not distinguish between upper case and lower case letters).
b: Each letter has a unique code which is a single digit from among 1,2,3, …, 9.
c: The digit 9 codes two letters, and every other digit codes three letters.
d: The code for a word is constructed by arranging the digits corresponding to its letters in a non-decreasing sequence.
Answer these questions on the basis of this information.
Question 17: What best can be concluded about the code for the letter L?
a) 1
b) 8
c) 1 or 8
d) 6
17) Answer (A)
Solution:

We can see that India’s code is 13366 therefore we can say that I’s code is either 3 or 6.
Also, we can see that code for word “is” is 35 therefore we can say that I’s code is 3. Consequently, we can say that S’s code is 5.
Also, we can see that code of word ‘as’ is 56 therefore we can say that A’s code is 6. Consequently, we can say that S’s code is 5.
There is only one letter ‘O’ common in words ‘of’ and ‘national’. In code word as well only digit ‘9’ is common in both. Hence, we can say that letter ‘O’ is assigned numerical ‘9’. Consequently, we can say that F is assigned number 7.

It is given that ‘9’ is assigned to only two alphabets one of them is ‘O’. We can see that there are three 9’s in Peacock’s code. One of the digit ‘9’ is used for ‘O’.Remaining two 9’s must represent same letter. We can see that only letter ‘C’ has appeared twice in Peacock. Therefore, we can say that ‘C’ is assigned number ‘9’.
In word national ‘N’ has appeared twice. In code only digit ‘6’ has appeared more than once. Hence, we can say that code of letter N is ‘6’. Consequently, we can say that code for letter ‘D’ is ‘1’ because in India rest of the numerals are already taken.

In words, ‘the’ and ‘national’ only letter ‘t’ is common. In code as well only digit ‘8’ is common in two codes. Hence, we can say that letter code for letter ‘t’ is 8.
In words, ‘the’ and ‘peacock’ only letter ‘e’ is common. In code as well only digit ‘5’ is common in two codes. Hence, we can say that letter code for letter ‘e’ is 5. Consequently, we can say that leftover letter, in word “the”, ‘H’s code is 4.

We can see that code for word “NATIONAL” is 13666689. Hence, we can say that code for the letter L is ‘1’. Hence, option A is the correct answer.
Question 18: What best can be concluded about the code for the letter B?
a) 3 or 4
b) 1 or 3 or 4
c) 1
d) 3
18) Answer (A)
Solution:

We can see that India’s code is 13366 therefore we can say that I’s code is either 3 or 6.
Also, we can see that code for word “is” is 35 therefore we can say that I’s code is 3. Consequently, we can say that S’s code is 5.
Also, we can see that code of word ‘as’ is 56 therefore we can say that A’s code is 6. Consequently, we can say that S’s code is 5.
There is only one letter ‘O’ common in words ‘of’ and ‘national’. In code word as well only digit ‘9’ is common in both. Hence, we can say that letter ‘O’ is assigned numerical ‘9’. Consequently, we can say that F is assigned number 7.

It is given that ‘9’ is assigned to only two alphabets one of them is ‘O’. We can see that there are three 9’s in Peacock’s code. One of the digit ‘9’ is used for ‘O’.Remaining two 9’s must represent same letter. We can see that only letter ‘C’ has appeared twice in Peacock. Therefore, we can say that ‘C’ is assigned number ‘9’.
In word national ‘N’ has appeared twice. In code only digit ‘6’ has appeared more than once. Hence, we can say that code of letter N is ‘6’. Consequently, we can say that code for letter ‘D’ is ‘1’ because in India rest of the numerals are already taken.

In words, ‘the’ and ‘national’ only letter ‘t’ is common. In code as well only digit ‘8’ is common in two codes. Hence, we can say that letter code for letter ‘t’ is 8.
In words, ‘the’ and ‘peacock’ only letter ‘e’ is common. In code as well only digit ‘5’ is common in two codes. Hence, we can say that letter code for letter ‘e’ is 5. Consequently, we can say that leftover letter, in word “the”, ‘H’s code is 4.

We can see that code for word “NATIONAL” is 13666689. Hence, we can say that code for the letter L is ‘1’.

We can see that code for word “BIRD” is 1334. 1 corresponds to D and one 3 corresponds to I. Hence, we can say that code for letters ‘R’ and ‘B’ are ‘3’ and ‘4’ in any order.
Therefore, we can say that for letter ‘B’ there are two possible numbers: 3 or 4
Hence, option A is the correct answer.
Question 19: For how many digits can the complete list of letters associated with that digit be identified?
a) 1
b) 2
c) 0
d) 3
19) Answer (B)
Solution:

We can see that India’s code is 13366 therefore we can say that I’s code is either 3 or 6.
Also, we can see that code for word “is” is 35 therefore we can say that I’s code is 3. Consequently, we can say that S’s code is 5.
Also, we can see that code of word ‘as’ is 56 therefore we can say that A’s code is 6. Consequently, we can say that S’s code is 5.
There is only one letter ‘O’ common in words ‘of’ and ‘national’. In code word as well only digit ‘9’ is common in both. Hence, we can say that letter ‘O’ is assigned numerical ‘9’. Consequently, we can say that F is assigned number 7.

It is given that ‘9’ is assigned to only two alphabets one of them is ‘O’. We can see that there are three 9’s in Peacock’s code. One of the digit ‘9’ is used for ‘O’.Remaining two 9’s must represent same letter. We can see that only letter ‘C’ has appeared twice in Peacock. Therefore, we can say that ‘C’ is assigned number ‘9’.
In word national ‘N’ has appeared twice. In code only digit ‘6’ has appeared more than once. Hence, we can say that code of letter N is ‘6’. Consequently, we can say that code for letter ‘D’ is ‘1’ because in India rest of the numerals are already taken.

In words, ‘the’ and ‘national’ only letter ‘t’ is common. In code as well only digit ‘8’ is common in two codes. Hence, we can say that letter code for letter ‘t’ is 8.
In words, ‘the’ and ‘peacock’ only letter ‘e’ is common. In code as well only digit ‘5’ is common in two codes. Hence, we can say that letter code for letter ‘e’ is 5. Consequently, we can say that leftover letter, in word “the”, ‘H’s code is 4.

We can see that code for word “NATIONAL” is 13666689. Hence, we can say that code for the letter L is ‘1’.

We can see that code for word “DESIGNATED” is 1135556678. Hence, we can say that code for the letter ‘G’ is ‘7’.

We can see that code for word “PEACOCK” is 5688999. Hence, we can say that code for the letters ‘P’ and ‘K’ is ‘8’.

Digit ‘1’ is used for L and D only. We can not figure out the third letter for which digit 1 is used.
Digit ‘2’ is not used for any letter. Hence, we can not figure out all the letters for which digit 2 is correct code.
Digit ‘3’ is used for letter ‘I’ only. Hence, we can not figure out all the letters for which digit 3 is correct code.
Digit ‘4’ is used for letters ‘H’ and one of ‘B’ and ‘R’. Hence, we can not figure out all the letters for which digit 4 is correct code.
Digit ‘5’ is used for letters ‘S’ and ‘E’. We can not figure out the third letter for which digit 5 is used.
Digit ‘6’ is used for letters ‘A’ and ‘N’. We can not figure out the third letter for which digit 6 is used.
Digit ‘7’ is used for letters ‘G’ and ‘F’. We can not figure out the third letter for which digit 7 is used.
Digit ‘8’ is used for letters ‘T’, ‘P’ and K. Hence, we can say that this is one of the digit for which the complete list of letters associated is known.
Digit ‘9’ is used for letters ‘C’ and ‘O’. Hence, we can say that this is one of the digit for which the complete list of letters associated is known.
Therefore, we can say that for only two digits (8 and 9), the complete list of letters associated is known. Hence, option B is the correct answer.
Question 20: Which set of letters CANNOT be coded with the same digit?
a) S,E,Z
b) I,B,M
c) S,U,V
d) X,Y,Z
20) Answer (C)
Solution:

We can see that India’s code is 13366 therefore we can say that I’s code is either 3 or 6.
Also, we can see that code for word “is” is 35 therefore we can say that I’s code is 3. Consequently, we can say that S’s code is 5.
Also, we can see that code of word ‘as’ is 56 therefore we can say that A’s code is 6. Consequently, we can say that S’s code is 5.
There is only one letter ‘O’ common in words ‘of’ and ‘national’. In code word as well only digit ‘9’ is common in both. Hence, we can say that letter ‘O’ is assigned numerical ‘9’. Consequently, we can say that F is assigned number 7.

It is given that ‘9’ is assigned to only two alphabets one of them is ‘O’. We can see that there are three 9’s in Peacock’s code. One of the digit ‘9’ is used for ‘O’.Remaining two 9’s must represent same letter. We can see that only letter ‘C’ has appeared twice in Peacock. Therefore, we can say that ‘C’ is assigned number ‘9’.
In word national ‘N’ has appeared twice. In code only digit ‘6’ has appeared more than once. Hence, we can say that code of letter N is ‘6’. Consequently, we can say that code for letter ‘D’ is ‘1’ because in India rest of the numerals are already taken.

In words, ‘the’ and ‘national’ only letter ‘t’ is common. In code as well only digit ‘8’ is common in two codes. Hence, we can say that letter code for letter ‘t’ is 8.
In words, ‘the’ and ‘peacock’ only letter ‘e’ is common. In code as well only digit ‘5’ is common in two codes. Hence, we can say that letter code for letter ‘e’ is 5. Consequently, we can say that leftover letter, in word “the”, ‘H’s code is 4.

We can see that code for word “NATIONAL” is 13666689. Hence, we can say that code for the letter L is ‘1’.

We can see that code for word “DESIGNATED” is 1135556678. Hence, we can say that code for the letter ‘G’ is ‘7’.

We can see that code for word “PEACOCK” is 5688999. Hence, we can say that code for the letters ‘P’ and ‘K’ is ‘8’.

Let us check this by options:
(A) S,E,Z: If letter ‘Z’ is assigned code ‘5’ then this case is possible.
(B) I,B,M: If letters ‘B’ and ‘M’ are assigned code ‘3’ then this case is possible.
(C) S,U,V: If letters ‘U’ and ‘V’ are assigned code ‘5’ then this case is possible. But in that case digit 5 will have 4 letters associated with it which is not possible. Hence, this is the answer.
(D) X,Y,Z: If letters ‘X’, ‘Y’ and ‘Z’ are assigned code ‘2’ then this case is possible.



![CAT Averages Questions PDF [Important Questions] CAT AVERAGES Questions PDF](https://cracku.in/blog/wp-content/uploads/2022/07/CAT-AVERAGES-Questions-PDF-218x150.png)
