Logical Reasoning and Data Interpretation (LRDI) is one of the most important sections of the CAT. For many aspirants, LRDI has become the deciding section in the CAT. Hence, it is extremely important that you take this section seriously. You can check out these LRDI CAT Previous year questions. Practice a good number of Sets in the CAT LRDI questions. In this article, we will look into some important LRDI Questions for CAT. These are a good source for practice; If you want to practice these questions, you can download this CAT LRDI Questions PDF below, which is completely Free.
Download LRDI Questions for CAT
Enroll for CAT 2022 Online Course
Instructions
A significant amount of traffic flows from point S to point T in the one-way street network shown below. Points A, B, C, and D are junctions in the network, and the arrows mark the direction of traffic flow. The fuel cost in rupees for travelling along a street is indicated by the number adjacent to the arrow representing the street. –
<img “=”” alt=”” class=”img-responsive” src=”https://cracku.in/media/questionGroup/DI-2.png”/>
Motorists traveling from point S to point T would obviously take the route for which the total cost of traveling is the minimum. If two or more routes have the same least travel cost, then motorists are indifferent between them. Hence, the traffic gets evenly distributed among all the least cost routes.
The government can control the flow of traffic only by levying appropriate toll at each junction. For example, if a motorist takes the route S-A-T (using junction A alone), then the total cost of travel would be Rs 14 (i.e., Rs 9 + Rs 5) plus the toll charged at junction A.
Question 1: If the government wants to ensure that all motorists travelling from S to T pay the same amount (fuel costs and toll combined) regardless of the route they choose and the street from B to C is under repairs (and hence unusable), then a feasible set of toll charged (in rupees) at junctions A, B, C, and D respectively to achieve this goal is:
a) 2,5,3,2
b) 0,5,3,2
c) 1,5,3,2
d) 2,3,5,1
e) 1,3,5,1
1) Answer (C)
Solution:
Let the toll charged at junctions A, B, C, and D be a,b,c and d respectively. Then the so that equal amount is collected through all route we have, 9+a+5=2+b+2+a+5=10+d+c=13+d. Then from the options only option C satisfies the above equality. hence option C.
Question 2: If the government wants to ensure that no traffic flows on the street from D to T, while equal amount of traffic flows through junctions A and C, then a feasible set of toll charged (in rupees) at junctions A, B, C,and D respectively to achieve this goal is:
a) 1,5,3,3
b) 1,4,4,3
c) 1,5,4,2
d) 0,5,2,3
e) 0,5,2,2
2) Answer (E)
Solution:
Let the toll charged at junctions A, B, C, and D be a,b,c and d respectively. Now since we want equal traffic through A and C , total cost through routes passing from A and C should be equal. So we have (9+a+5) + (2+b+2+a+5) = (2+3+b+c+2) + (7+d+1+c+2) . Only option E satisfy the above equality.
Question 3: If the government wants to ensure that all routes from S to T get the same amount of traffic, then a feasible set of toll charged (in rupees) at junctions A, B, C, and D respectively to achieve this goal is:
a) 0, 5, 2, 2
b) 0,5,4,1
c) 1,5,3,3
d) 1, 5, 3,2
e) 1,5,4,2
3) Answer (D)
Solution:
Now the fuel cost along different routes are :
SAT = 14
SBAT = 9
SBCT = 7
SDCT = 10
SDT = 13
Now , if we consider option D . Total cost for all routes comes out to be same which is 15. Hence option D.
Question 4: If the government wants to ensure that the traffic at S gets evenly distributed along streets from S to A, from S to B, and from S to D, then a feasible set of toll charged (in rupees) at junctions A, B, C, and D respectively to achieve this goal is:
a) 0,5,4,1
b) 0,5,2,2
c) 1,5,3,3
d) 1,5,3,2
e) 0,4,3,2
4) Answer (A)
Solution:
Total cost = fuel cost + toll
Total cost along SAT : 14+tollA
Total cost along SBAT : 9+tollA+tollB
Total cost along SDT : 13+tollD
Now when option A is considered , total costs come out to be same.
Hence option A is correct.
Question 5: The government wants to devise a toll policy such that the total cost to the commuters per trip is minimized. The policy should also ensure that not more than 70 per cent of the total traffic passes through junction B. The cost incurred by the commuter travelling from point S to point T under this policy will be:
a) Rs 7
b) Rs 9
c) Rs 10
d) Rs 13
e) Rs 14
5) Answer (C)
Solution:
The costs of the routes are as given below:
S – B – C – T = 7
S – B – A – T = 9
S – D – C – T = 10
S – D – T = 13
S – A -T = 14
Hence now 100% of the traffic flows through S – B – C – T
Now if we make the cost of traveling through S – B – C – T same as some other route not going through B, then the traffic will be equally distributed between these two routes. The lowest such route is S-D-C-T. The difference in cost = 3. Hence if we levy a toll of Rs.3 at B, the costs of SBCT and SBAT become 10,12 respectively and other routes are not affected. So 50% traffic flows through SBCT and 50% flows through SDCT . Hence cost in this policy = 10.
Checkout: CAT Free Practice Questions and Videos
Instructions
A study to look at the early learning of rural kids was carried out in a number of villages spanning three states, chosen from the North East (NE), the West (W) and the South (S). 50 four-year old kids each were sampled from each of the 150 villages from NE, 250 villages from W and 200 villages from S. It was found that of the 30000 surveyed kids 55% studied in primary schools run by government (G), 37% in private schools (P) while the remaining 8% did not go to school (O).
The kids surveyed were further divided into two groups based on whether their mothers dropped out of school before completing primary education or not.. The table below gives the number of kids in different types of schools for mothers who dropped out of school before completing primary education:
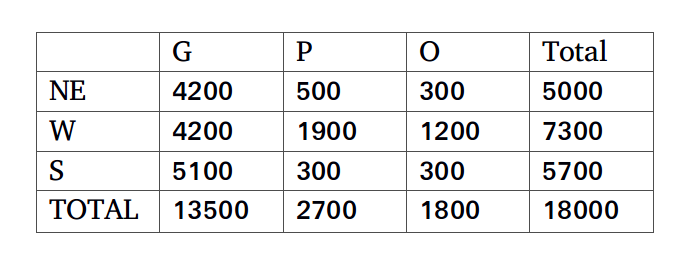
It is also known that:
1. In S, 60% of the surveyed kids were in G. Moreover, In S, all surveyed kids whose mothers had completed primary education were in school.
2. In NE, among the O kids, 50% had mothers who had dropped out before completing primary education.
3. The number of kids in G in NE was the same as the number of kids in G in W.
Question 6: What percentage of kids from S were studying in P?
a) 37%
b) 6%
c) 79%
d) 56%
6) Answer (A)
Solution:
Let us make note of the information given in the set.
The set states that rural kids were surveyed from 3 regions NE, West and South.
50 students each were surveyed from 150 villages in the NE, 250 villages from the West and 200 villages from the South.
Total number of kids from the NE = 150*50 = 7500
Total number of kids from the West = 250*50 = 12500
Total number of kids from the South = 200*50 = 10,000.
The table, given in the question, gives the number of students whose mothers dropped out before completing primary education. Therefore, the mothers of the remaining students should have completed primary education.
There are 7500 students from the NE in total. The mothers of 5000 of those students dropped out before completing primary education. Therefore, the remaining 2500 kids should have mothers who have completed primary education.
Let us make 2 tables – one representing the number of students and the other representing the number of students whose mother has completed primary education.
55% of the surveyed kids studied in schools run by the Government.
Number of kids studying in Govt. School should be 0.55*30000 = 16,500
From the given table, we know that the mothers of 13,500 kids who went to Govt. Schools dropped out of primary school.
Therefore, the remaining 16,500 – 13,500 = 3000 kids should have mother who have completed primary school.
37% of the surveyed kids study in private schools.
Number of kids studying in private schools should be 0.37*30000 = 11,100.
Number of kids in private schools whose mothers have completed primary school = 11,100 – 2700 = 8400.
8% of the surveyed kids did not go to school.
Number of kids who did not go to school = 0.08* 30000 = 2400
Number of kids not going to schools whose mothers have completed primary school = 2400 – 1800 = 600.
In S, 60% of the surveyed kids were in G.
Therefore, 6000 kids in S must be from G.
In NE, among the O kids, 50% had mothers who had dropped out before completing primary education.
There are 300 O kids whose mothers dropped out before completing primary education. These kids represent 50% of the total number of O-kids. Therefore, there must be 600 O-kids from NE – 300 kids should have mothers who dropped out before completing primary education and 300 kids should have mothers who have completed primary education.
The number of kids in G in NE was the same as the number of kids in G in W. Therefore, the number of kids in G in NE and the number of kids in G in W should be equal to 5250.
Total number of kids:

Number of kids whose mothers have completed primary education:

We have been given that in S, all surveyed kids whose mothers had completed primary education were in school. Therefore, the number of kids not going to school whose mothers have completed primary education in S should be 0. Filling the tables, we get,
Total number of kids:

Number of kids whose mothers have completed primary education:

As we can see from the table, 3700 kids out of the 10,000 kids from S are studying in P. 37% of the total number of students from S were studying in P. Therefore, option A is the right answer.
Question 7: Among the kids in W whose mothers had completed primary education, how many were not in school?
a) 300
b) 1200
c) 1050
d) 1500
7) Answer (A)
Solution:
Let us make note of the information given in the set.
The set states that rural kids were surveyed from 3 regions NE, West and South.
50 students each were surveyed from 150 villages in the NE, 250 villages from the West and 200 villages from the South.
Total number of kids from the NE = 150*50 = 7500
Total number of kids from the West = 250*50 = 12500
Total number of kids from the South = 200*50 = 10,000.
The table, given in the question, gives the number of students whose mothers dropped out before completing primary education. Therefore, the mothers of the remaining students should have completed primary education.
There are 7500 students from the NE in total. The mothers of 5000 of those students dropped out before completing primary education. Therefore, the remaining 2500 kids should have mothers who have completed primary education.
Let us make 2 tables – one representing the number of students and the other representing the number of students whose mother has completed primary education.
55% of the surveyed kids studied in schools run by the Government.
Number of kids studying in Govt. School should be 0.55*30000 = 16,500
From the given table, we know that the mothers of 13,500 kids who went to Govt. Schools dropped out of primary school.
Therefore, the remaining 16,500 – 13,500 = 3000 kids should have mother who have completed primary school.
37% of the surveyed kids study in private schools.
Number of kids studying in private schools should be 0.37*30000 = 11,100.
Number of kids in private schools whose mothers have completed primary school = 11,100 – 2700 = 8400.
8% of the surveyed kids did not go to school.
Number of kids who did not go to school = 0.08* 30000 = 2400
Number of kids not going to schools whose mothers have completed primary school = 2400 – 1800 = 600.
In S, 60% of the surveyed kids were in G.
Therefore, 6000 kids in S must be from G.
In NE, among the O kids, 50% had mothers who had dropped out before completing primary education.
There are 300 O kids whose mothers dropped out before completing primary education. These kids represent 50% of the total number of O-kids. Therefore, there must be 600 O-kids from NE – 300 kids should have mothers who dropped out before completing primary education and 300 kids should have mothers who have completed primary education.
The number of kids in G in NE was the same as the number of kids in G in W. Therefore, the number of kids in G in NE and the number of kids in G in W should be equal to 5250.
Total number of kids:

Number of kids whose mothers have completed primary education:

We have been given that in S, all surveyed kids whose mothers had completed primary education were in school. Therefore, the number of kids not going to school whose mothers have completed primary education in S should be 0. Filling the tables, we get,
Total number of kids:

Number of kids whose mothers have completed primary education:

There are 300 students who are not in school in W whose mothers have completed primary education. Therefore, option A is the right answer.
Question 8: In a follow up survey of the same kids two years later, it was found that all the kids were now in school. Of the kids who were not in school earlier, in one region, 25% were in G now, whereas the rest were enrolled in P; in the second region, all such kids were in G now; while in the third region, 50% of such kids had now joined G while the rest had joined P. As a result, in all three regions put together, 50% of the kids who were earlier out of school had joined G. It was also seen that no surveyed kid had changed schools.
What number of the surveyed kids now were in G in W?
a) 6000
b) 5250
c) 6750
d) 6300
8) Answer (A)
Solution:
Let us make note of the information given in the set.
The set states that rural kids were surveyed from 3 regions NE, West and South.
50 students each were surveyed from 150 villages in the NE, 250 villages from the West and 200 villages from the South.
Total number of kids from the NE = 150*50 = 7500
Total number of kids from the West = 250*50 = 12500
Total number of kids from the South = 200*50 = 10,000.
The table, given in the question, gives the number of students whose mothers dropped out before completing primary education. Therefore, the mothers of the remaining students should have completed primary education.
There are 7500 students from the NE in total. The mothers of 5000 of those students dropped out before completing primary education. Therefore, the remaining 2500 kids should have mothers who have completed primary education.
Let us make 2 tables – one representing the number of students and the other representing the number of students whose mother has completed primary education.
55% of the surveyed kids studied in schools run by the Government.
Number of kids studying in Govt. School should be 0.55*30000 = 16,500
From the given table, we know that the mothers of 13,500 kids who went to Govt. Schools dropped out of primary school.
Therefore, the remaining 16,500 – 13,500 = 3000 kids should have mother who have completed primary school.
37% of the surveyed kids study in private schools.
Number of kids studying in private schools should be 0.37*30000 = 11,100.
Number of kids in private schools whose mothers have completed primary school = 11,100 – 2700 = 8400.
8% of the surveyed kids did not go to school.
Number of kids who did not go to school = 0.08* 30000 = 2400
Number of kids not going to schools whose mothers have completed primary school = 2400 – 1800 = 600.
In S, 60% of the surveyed kids were in G.
Therefore, 6000 kids in S must be from G.
In NE, among the O kids, 50% had mothers who had dropped out before completing primary education.
There are 300 O kids whose mothers dropped out before completing primary education. These kids represent 50% of the total number of O-kids. Therefore, there must be 600 O-kids from NE – 300 kids should have mothers who dropped out before completing primary education and 300 kids should have mothers who have completed primary education.
The number of kids in G in NE was the same as the number of kids in G in W. Therefore, the number of kids in G in NE and the number of kids in G in W should be equal to 5250.
Total number of kids:

Number of kids whose mothers have completed primary education:

We have been given that in S, all surveyed kids whose mothers had completed primary education were in school. Therefore, the number of kids not going to school whose mothers have completed primary education in S should be 0. Filling the tables, we get,
Total number of kids:

Number of kids whose mothers have completed primary education:

Number of kids who were out of school in the initial survey = 2400.
The number of kids who were out of school in NE is 600, W is 1500 and S is 300.
25% of the kids from one area, 100% of the kids from one area and 50% of the kids from another area are transferred to G. As a result, the number of kids who were not in school earlier but studying in G now became 50% of 2400 = 1200.
100% of the kids in W could not have been transferred to G (Since the total number of kids transferred to G is 1200 and the number of kids in W alone is 1500).
Let us assume that 100% of the kids from NE transferred (600). In this case, if 25% of the kids from W are transferred (375), 50% of the kids from S (300) will be transferred. The total in this case would be 600 + 375+ 300 = 1275. Therefore, we can eliminate this case.
If 100% of the kids from NE (600), 25% of the kids from S (75) and 50% of the kids from W (750) are transferred, then the total would have been 1425. Therefore, we can eliminate this case as well.
If 50% of the kids from W (750), 25% of the kids from NE (150) and 100% of the kids from S (300) are transferred, then the total number of kids transferred to G would have been 750 + 150 + 300 = 1200. Therefore, this must have been the case.
Number of students transferred to G in W = 750.
Number of students present in G in W earlier = 5250
Total = 6000
Therefore, option A is the right answer.
Question 9: In a follow up survey of the same kids two years later, it was found that all the kids were now in school. Of the kids who were not in school earlier, in on.e region, 25% were in G now, whereas the rest were enrolled in P; in the second region, all such kids were in G now; while in the third region, 50% of such kids had now joined G while the rest had joined P. As a result, in all three regions put together, 50% of the kids who were earlier out of school had joined G. It was also seen that no surveyed kid had changed schools.
What percentage of the surveyed kids in S, whose mothers had dropped out before completing primary education, were in G now?
a) 94.7%
b) 89.5%
c) 93.4%
d) Cannot be determined from the given information
9) Answer (A)
Solution:
Let us make note of the information given in the set.
The set states that rural kids were surveyed from 3 regions NE, West and South.
50 students each were surveyed from 150 villages in the NE, 250 villages from the West and 200 villages from the South.
Total number of kids from the NE = 150*50 = 7500
Total number of kids from the West = 250*50 = 12500
Total number of kids from the South = 200*50 = 10,000.
The table, given in the question, gives the number of students whose mothers dropped out before completing primary education. Therefore, the mothers of the remaining students should have completed primary education.
There are 7500 students from the NE in total. The mothers of 5000 of those students dropped out before completing primary education. Therefore, the remaining 2500 kids should have mothers who have completed primary education.
Let us make 2 tables – one representing the number of students and the other representing the number of students whose mother has completed primary education.
55% of the surveyed kids studied in schools run by the Government.
Number of kids studying in Govt. School should be 0.55*30000 = 16,500
From the given table, we know that the mothers of 13,500 kids who went to Govt. Schools dropped out of primary school.
Therefore, the remaining 16,500 – 13,500 = 3000 kids should have mother who have completed primary school.
37% of the surveyed kids study in private schools.
Number of kids studying in private schools should be 0.37*30000 = 11,100.
Number of kids in private schools whose mothers have completed primary school = 11,100 – 2700 = 8400.
8% of the surveyed kids did not go to school.
Number of kids who did not go to school = 0.08* 30000 = 2400
Number of kids not going to schools whose mothers have completed primary school = 2400 – 1800 = 600.
In S, 60% of the surveyed kids were in G.
Therefore, 6000 kids in S must be from G.
In NE, among the O kids, 50% had mothers who had dropped out before completing primary education.
There are 300 O kids whose mothers dropped out before completing primary education. These kids represent 50% of the total number of O-kids. Therefore, there must be 600 O-kids from NE – 300 kids should have mothers who dropped out before completing primary education and 300 kids should have mothers who have completed primary education.
The number of kids in G in NE was the same as the number of kids in G in W. Therefore, the number of kids in G in NE and the number of kids in G in W should be equal to 5250.
Total number of kids:

Number of kids whose mothers have completed primary education:

We have been given that in S, all surveyed kids whose mothers had completed primary education were in school. Therefore, the number of kids not going to school whose mothers have completed primary education in S should be 0. Filling the tables, we get,
Total number of kids:

Number of kids whose mothers have completed primary education:

Number of kids who were out of school in the initial survey = 2400.
The number of kids who were out of school in NE is 600, W is 1500 and S is 300.
25% of the kids from one area, 100% of the kids from one area and 50% of the kids from another area are transferred to G. As a result, the number of kids who were not in school earlier but studying in G now became 50% of 2400 = 1200.
100% of the kids in W could not have been transferred to G (Since the total number of kids transferred to G is 1200 and the number of kids in W alone is 1500).
Let us assume that 100% of the kids from NE transferred (600). In this case, if 25% of the kids from W are transferred (375), 50% of the kids from S (300) will be transferred. The total in this case would be 600 + 375+ 300 = 1275. Therefore, we can eliminate this case.
If 100% of the kids from NE (600), 25% of the kids from S (75) and 50% of the kids from W (750) are transferred, then the total would have been 1425. Therefore, we can eliminate this case as well.
If 50% of the kids from W (750), 25% of the kids from NE (150) and 100% of the kids from S (300) are transferred, then the total number of kids transferred to G would have been 750 + 150 + 300 = 1200. Therefore, this must have been the case.
Already, 5100 kids whose mothers had dropped out were in G in S.
After the transfer, 300 more students will be added to the count.
Therefore, 5400 students whose mothers had dropped out will be in G in S.
Total number of kids whose mothers had dropped out in S = 5700
Percentage = 5400/5700 = 94.73%.
Therefore, option A is the right answer.
Instructions
Question Numbers: (55 to 58)
In a square layout of site 5m ~ 5m 25 equal-sized square platforms of different heights are built. The heights (in metre) of individual platforms are as shown below:
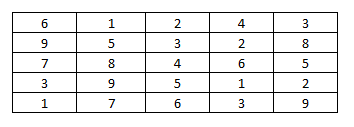
Individuals (all of same height) are seated on these platforms. We say an individual A can reach individual B, if all the three following conditions are met;
(i) A and B are In the same row or column
(ii) A is at a lower height than B
(iii) If there is/are any individuals (s) between A and B, such individual(s) must be at a height lower than that of A.
Thus in the table given above, consider the Individual seated at height 8 on 3rd row and 2nd column. He can be reached by four individuals. He can be reached by the individual on his left at height 7, by the two individuals on his right at heights of 4 and 6 and by the individual above at height 5.
Rows in the layout are numbered from top to bottom and columns are numbered from left to right.
Question 10: How many individuals in this layout can be reached by just one individual?
a) 3
b) 5
c) 7
d) 8
10) Answer (C)
Solution:
The table represent the platforms and by how many platforms can it be reached. For example, in row 1 and column 1, platform with height 6 can be reached by 3 people.
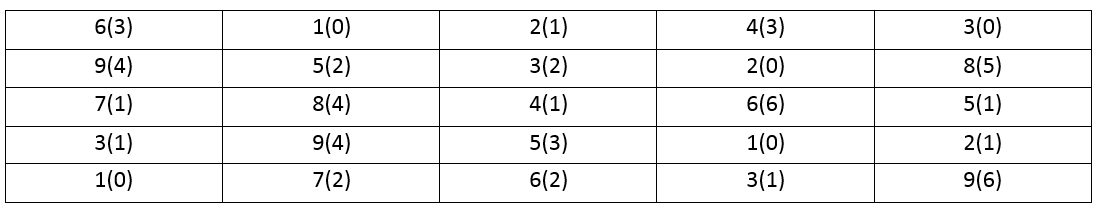
Hence, there are a total of 7 people.
Question 11: Which of the following is true for any individual at a platform of height 1 m in this layout?
a) They can be reached by all the individuals in their own row and column.
b) They can be reached by at least 4 individuals.
c) They can be reached by at least one individual.
d) They cannot be reached by anyone.
11) Answer (D)
Solution:
Since, we have been given that a person can be reached only by those who are smaller than him. Hence, 1 cannot be reached by anyone. Thus, option D is the correct answer.
Question 12: We can find two individuals who cannot be reached by anyone in
a) the last row.
b) the fourth row.
c) the fourth column.
d) the middle column.
12) Answer (C)
Solution:
Check out the options.
In last row, only one individual can’t be reached by anyone.
In the fourth row, only one individual can’t be reached by anyone.
In the middle column, every individual can be reached by atleast another individual.
In the fourth column, both 1 and 2 cannot be reached by any person. Hence, fourth column is the correct answer.
Question 13: Which of the following statements is true about this layout?
a) Each row has an individual who can be reached by 5 or more individuals.
b) Each row has an individual who cannot be reached by anyone.
c) Each row has at least two individuals who can be reached by an equal number of individuals.
d) All individuals at the height of 9 m can be reached by at least 5 individuals.
13) Answer (C)
Solution:
Let us go by options.
Option A: In first row, 6 is reached by 3, 1 by none, 2 by none, 4 by 3, 3 by none. Hence, ruled out.
Option B: Third row, every individual is approached by other.
Option D: In first row, 6 is reached by 3, 1 by none, 2 by none, 4 by 3, 3 by none. Hence, ruled out.
Hence, Option C is correct.
Instructions
An old woman had the following assets:
(a) Rs. 70 lakh in bank deposits
(b) 1 house worth Rs. 50 lakh
(c) 3 flats, each worth Rs. 30 lakh
(d) Certain number of gold coins, each worth Rs. 1 lakh
She wanted to distribute her assets among her three children; Neeta, Seeta and Geeta.
The house, any of the flats or any of the coins were not to be split. That is, the house went entirely to one child; a flat went to one child and similarly, a gold coin went to one child.
Question 14: Among the three, Neeta received the least amount in bank deposits, while Geeta received the highest. The value of the assets was distributed equally among the children, as were the gold coins.
How much did Seeta receive in bank deposits (in lakhs of rupees)?
a) 30
b) 40
c) 20
d) 10
14) Answer (C)
Solution:
Neeta received least amount in bank deposits implies she received highest amount in property and the vice-versa for Geeta. The assets are 3 flats worth 90 lakh,a house worth 50 lakh, and a deposit worth 70 lakh. The total value of assets is 210 lakhs. They are divided equally, so each will receive assets worth 70 lakh.
No one daughter can get 3 flats as the total value of asset will be 90 lakhs which is greater than actual share.
All three daughters can’t get 1-1 flat each as well. In that case, the daughter who owns 1 flat and the house will have assets worth 30+50 = 80 lakhs which is more than the actual share. Hence, we can conclude the one of the three daughter gets 2 flats and 10 lakhs bank deposit.
Out of the remaining two daughters, one will get the house and bank deposit worth 20 lakhs and the other one must have 1 flat and 40 lakhs in bank deposit. On the basis of bank distribution we can easily determine that property and bank deposits for each Neera, Seeta and Geeta.
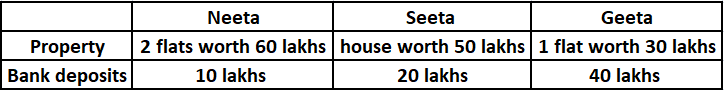
From the table, we can see that Seeta must have received Rs. 20 lakh in bank deposits. Hence, option C is the correct answer.
Question 15: Among the three, Neeta received the least amount in bank deposits, while Geeta received the highest. The value of the assets was distributed equally among the children, as were the gold coins.
How many flats did Neeta receive?
15) Answer: 2
Solution:
Neeta received least amount in bank deposits implies she received highest amount in property and the vice-versa for Geeta. The assets are 3 flats worth 90 lakh,a house worth 50 lakh, and a deposit worth 70 lakh. The total value of assets is 210 lakhs. They are divided equally, so each will receive assets worth 70 lakh.
No one daughter can get 3 flats as the total value of asset will be 90 lakhs which is greater than actual share.
All three daughters can’t get 1-1 flat each as well. In that case, the daughter who owns 1 flat and the house will have assets worth 30+50 = 80 lakhs which is more than the actual share. Hence, we can conclude the one of the three daughter gets 2 flats and 10 lakhs bank deposit.
Out of the remaining two daughters, one will get the house and bank deposit worth 20 lakhs and the other one must have 1 flat and 40 lakhs in bank deposit. On the basis of bank distribution we can easily determine that property and bank deposits for each Neera, Seeta and Geeta.

From the table, we can see that Neeta received 2 flats.
Question 16: The value of the assets distributed among Neeta, Seeta and Geeta was in the ratio of 1:2:3, while the gold coins were distributed among them in the ratio of 2:3:4. One child got all three flats and she did not get the house. One child, other than Geeta, got Rs. 30 lakh in bank deposits.
How many gold coins did the old woman have?
a) 72
b) 90
c) 180
d) 216
16) Answer (B)
Solution:
Let the total number of gold coins with the old woman be ‘9n’.
Total value of the assets with the old woman = 50 + 3*30 + 70 + 9n = 210+9n.
We know that the assets have been distributed in the ratio 1:2:3.
Therefore, Neeta must have received 35+1.5n (by value), Seeta must have received 70+3n and Geeta must have received 105+4.5n.
Further, it has been given that the gold coins distributed were in the ratio 2:3:4.
Therefore, the number of gold coins with Neeta must be ‘2n’, Seeta must be ‘3n’ and Geeta must be ‘4n’.
Seeta has ‘3n’ gold coins. Therefore, the total value of the assets with her must be 70. Seeta could not have inherited all the flats. Therefore, Seeta must have received the house ( worth 50 lakh) and 20 lakh from bank deposits.
We know that Geeta did not receive Rs. 30 lakh from the bank deposits. Therefore, Neeta must have received Rs. 30 lakh.
The remaining 5 lakh must be contributed by the gold coins (Since there is no other asset worth 5 lakh).
=> 5 + 1.5n = 2n
=> 0.5n = 5
=> n = 10
The old-woman must have had 10*9 = 90 gold coins. Therefore, option B is the right answer.
Question 17: The value of the assets distributed among Neeta, Seeta and Geeta was in the ratio of 1:2:3, while the gold coins were distributed among them in the ratio of 2:3:4. One child got all three flats and she did not get the house. One child, other than Geeta, got Rs. 30 lakh in. bank deposits.
How much did Geeta get in bank deposits (in lakhs of rupees)?
17) Answer: 20
Solution:
Let the total number of gold coins with the old woman be ‘9n’.
Total value of the assets with the old woman = 50 + 3*30 + 70 + 9n = 210+9n.
We know that the assets have been distributed in the ratio 1:2:3.
Therefore, Neeta must have received 35+1.5n (by value), Seeta must have received 70+3n and Geeta must have received 105+4.5n.
Further, it has been given that the gold coins distributed were in the ratio 2:3:4.
Therefore, the number of gold coins with Neeta must be ‘2n’, Seeta must be ‘3n’ and Geeta must be ‘4n’.
Seeta has 3n gold coins. Therefore, the total value of the assets with her must be 70. Seeta could not have inherited all the flats. Therefore, Seeta must have received the house ( worth 50 lakh) and 20 lakh from bank deposits.
We know that Geeta did not receive Rs. 30 lakh from the bank deposits. Therefore, Neeta must have received Rs. 30 lakh.
The remaining 5 lakh must be contributed by the gold coins (Since there is no other asset worth 5 lakh).
=> 5 + 1.5n = 2n
=> 0.5n = 5
=> n = 10
The oldwoman must have had 10*9 = 90 gold coins.
Total assets = 210 + 90*1 = 300 lakh
Neeta has received 50 lakh in total, Seeta has received 100 lakh and Geeta has received 150 lakh.
Geeta must have received 90 lakh from 3 flats. Out of the remaining 60 lakh, 4*10 = 40 lakh has been contributed by the gold coins. Geeta must have received 150 – 90 – 40 = 20 lakh from bank deposits. Therefore, 20 is the right answer.
LRDI Videos | Preparation Videos for CAT LRDI
- Try these 3 Cracku Free CAT Mocks, which come with detailed solutions and with video explanations.




![CAT Averages Questions PDF [Important Questions] CAT AVERAGES Questions PDF](https://cracku.in/blog/wp-content/uploads/2022/07/CAT-AVERAGES-Questions-PDF-218x150.png)
