CAT Level Questions on Geometry
Download important CAT Level Questions on Geometry PDF based on previously asked questions in CAT exam. Practice Geometry Questions PDF for CAT exam.
Download CAT Level Questions on Geometry
CAT Crash Couse – Sufficient To Crack The Exam
Download CAT Quant Questions PDF
Question 1: If the lengths of diagonals DF, AG and CE of the cube shown in the adjoining figure are equal to the three sides of a triangle, then the radius of the circle circumscribing that triangle will be?
a) equal to the side of the cube
b) $\sqrt 3$ times the side of the cube
c) 1/$\sqrt 3$ times the side of the cube
d) impossible to find from the given information
Question 2: A circle circumscribes a square. What is the area of the square?I. Radius of the circle is given.II. Length of the tangent from a point 5 cm away from the centre of the circle is given.
a) The question can be answered with the help of any one statement alone but not by the other statement.
b) The question can be answered with the help of either of the statements taken individually.
c) The question can be answered with the help of both statements together.
d) The question cannot be answered even with the help of both statements together.
Question 3:
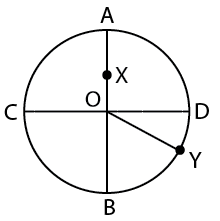
In a circular field, AOB and COD are two mutually perpendicular diameters having length of 4 meters. X is the mid – point of OA. Y is the point on the circumference such that ∠YOD = 30°. Which of the following correctly gives the relation among the three alternate paths from X to Y?
a) XOBY : XODY : XADY :: 5.15 : 4.50 : 5.06
b) XADY : XODY : XOBY :: 6.25 : 5.34 : 4.24
c) XODY : XOBY : XADY :: 4.04 : 5.35 : 5.25
d) XADY : XOBY : XODY :: 5.19 : 5.09 : 4.04
e) XOBY : XADY : XODY :: 5.06 : 5.15 : 4.50
Question 4: In the figure below, AB = AC = CD. If ADB = 20°, what is the value of BAD?
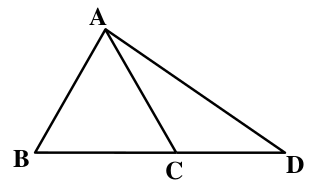
a) 40°
b) 60°
c) 70°
d) 120°
e) 140°
Question 5: The figure below has been obtained by folding a rectangle. The total area of the figure (as visible) is 144 square meters. Had the rectangle not been folded, the current overlapping part would have been a square. What would have been the total area of the original unfolded rectangle?
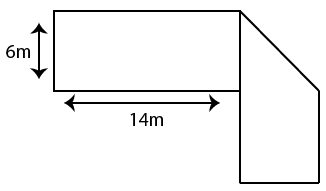
a) 128 square meters
b) 154 square meters
c) 162 square meters
d) 172 square meters
e) None of the above
Download CAT Quant Questions PDF
Question 6: The parallel sides of a trapezoid ABCD are in the ratio of 4 : 5. ABCD is divided into an isosceles triangle ABP and a parallelogram PBCD (as shown below). ABCD has a perimeter equal to 1120 meters and PBCD has a perimeter equal to 1000 meters. Find Sin∠ABC, given 2∠DAB = ∠BCD.
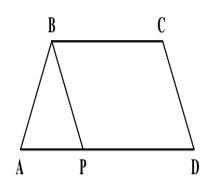
a) 4/5
b) 16/25
c) 5/6
d) 24/25
e) A single solution is not possible
Question 7: What is the value of $c^{2} $ in the given figure, where the radius of the circle is ‘a’ unit.
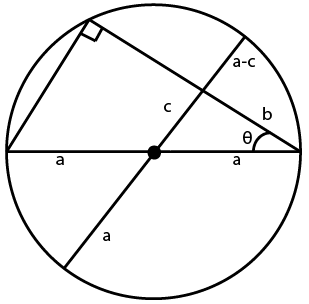
a) $c^{2} = a^{2} + b^{2} – 2ab cos θ$
b) $c^{2} = a^{2} + b^{2} – 2ab sin θ$
c) $c^{2} = a^{2} – b^{2} + 2ab cos θ$
d) None of these
Question 8: If in the figure below, angle XYZ = 90° and the length of the arc XZ = 10π, then the area of the sector XYZ is
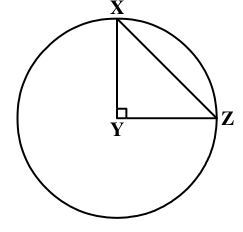
a) 10π
b) 25π
c) 100π
d) None of the above
Question 9: A cone of radius 4 cm with a slant height of 12 cm was sliced horizontally, resulting into a smaller cone (upper portion) and a frustum (lower portion). If the ratio of the curved surface area of the upper smaller cone and the lower frustum is 1:2, what will be the slant height of the frustum?
a) $12-\sqrt{3}$
b) $12-2\sqrt{3}$
c) $12-3\sqrt{3}$
d) $12-4\sqrt{3}$
e) None of the above
Question 10: Let ABCDEF be a regular hexagon with each side of length 1 cm. The area (in sq cm) of a square with AC as one side is
a) $3\sqrt{2}$
b) $3$
c) $4$
d) $\sqrt{3}$
Download CAT Quant Questions PDF
Answers & Solutions:
1) Answer (A)
Consider side of the cube as x.
So diagonal will be of length $\sqrt{3}$ * x.
Now if diagonals are side of equilateral triangle we get area = 3*$\sqrt{3}*x^2$ /4 .
Also in a triangle
4 * Area * R = Product of sides
4* 3*$\sqrt{3}*x^2$ /4 * R = .3*$\sqrt{3}*x^3$
R = x
2) Answer (B)
As the circle circumscribes the square, diameter of the circle= diagonal of the square. Let the side of the circle be s. If we can determine the value of s, then we can find the area of the square $s^2$.
Statement 1: Let the radius be r. 2r = $s \sqrt{2}$. Hence, s = $r\sqrt{2}$. Thus, we can find the area of the circle using this information.
Statement 2: This scenario can be drawn as shown below:

A line drawn from the center of the circle intersects the tangent at an angle of 90°. Hence, the triangle formed is a right-angled triangle with the hypotenuse of 5 cm, one side equal to the radius of the circle and second side equal to the length of the tangent. If the length of the tangent is known then we can calculate the radius using Pythogoras theorem. If the radius is known, the area of the square can be calculated as shown above.
3) Answer (D)
XADY = XA + AD + DY = 2/2 + (2 * 3.14 * 2)/4 + (30/360) * (2 * 3.14 * 2) = 5.19
XOBY = XO + OB + BY = 2/2 + 2 + (60/360) * (2 * 3.14 * 2) = 5.09
XODY = XO + OD + DY = 2/2 + 2 + (30/360) * (2 * 3.14 * 2) = 4.04
Hence, option D is the correct answer.
4) Answer (D)

AB = AC = CD, => $\angle CAD = \angle CDA = 20^{\circ}$
and $\angle ABC = \angle ACB$
In $\triangle$ ACD
=> $\angle ACD + \angle CAD + \angle CDA = 180^{\circ}$
=> $\angle ACD = 180^{\circ} – 20^{\circ} – 20^{\circ} = 140^{\circ}$
=> $\angle ACB = 180^{\circ} – 140^{\circ} = 40^{\circ} = \angle ABC$
Similarly, In $\triangle$ ABC
=> $\angle BAC = 180^{\circ} – 40^{\circ} – 40^{\circ} = 100^{\circ}$
$\therefore \angle BAD = 100^{\circ} + 20^{\circ} = 120^{\circ}$
5) Answer (C)

Area of given figure = 144 sq meter
It is given that BCE becomes square when we will unfold it, so to find the complete area of the figure shown as dotted after unfolding we need to add the area of triangle BCE.
Thus, BC = CE = 6 m
=> Area of $\triangle$ BCE = $\frac{1}{2} \times 6 \times 6 = 18$ sq meter
$\therefore$ Final area of whole figure = 144 + 18 = 162 square meter.
Free CAT Practice – Study Material
Download CAT Quant Formulas PDF
6) Answer (A)
AB + BC + CD + AD = 1120 ————Eqn(I)
PB + BC + CD + PD = 1000 ————-Eqn(II)
Subtracting eqn(II) from (I), we get :
=> AB – PB + (AD – PD) = 120
=> AB – PB + AP = 120
=> AB + AP = 120 + PB
Now, if AB = PB, => AP = 120
=> AD = 600 and BC = 480, then AB + PB + CD = 40, which is not possible (We know that BC = PD. If BC = PD = 480, then BC+PD = 960. PB + BC + CD + PD = 1000.
=> PB+CD = 40. Therefore, AB + PB+CD should be greater than 40).
Similarly, AB = AP is also not possible. Thus $AP = BP$

=> $\angle ABC = x + (180 – 2x) = (180 – x)$
=> $sin \angle ABC = sin (180 – x) = sin x$
Also, perimeter of PBCD = $10y = 1000$ => $y = 100$
and perimeter of ABCD = $AB + 10y = 1120$ => $AB = 120$
Applying cosine rule in $\triangle$ ABP
=> $cos x = \frac{(AB)^2 + (AP)^2 – (BP)^2}{2 AB AP}$
=> $cos x = \frac{(120)^2 + (100)^2 – (100)^2}{2 \times 120 \times 100}$
=> $cos x = \frac{120}{200} = \frac{3}{5}$
$\therefore sin x = \sqrt{1 – (\frac{3}{5})^2} = \sqrt{1 – \frac{9}{25}}$
= $\sqrt{\frac{16}{25}} = \frac{4}{5}$
7) Answer (A)
Applying the cosine rule , we get $c^{2} = a^{2} + b^{2} – 2ab cos θ$
Hence, option A is the correct answer.
8) Answer (C)
Length of arc XZ is the perimeter of quarter circle.
$\frac{\pi r}{2}$ = 10$\pi$
r=20
Area of sector XYZ = Area of quarter circle = $\frac{\pi r^{2}}{4}$
Area = 100$\pi$
9) Answer (D)
The ratio of the curved surface area of the upper cone to the lower frustum is 1:2.
=> the ratio of the curved surface area of the upper cone to the total cone = 1:3.
Curved surface area (CSA) of a cone = $\pi*r*l$
For the given cone, the slant height, $l=12$cm
CSA of the cone = $48*\pi$
CSA of the smaller cone = $16*\pi$
Both the slant height and the radius would have been reduced by the same ratio. Let that ratio be $x$.
$x^2*48*\pi$=$16\pi$
=>$x^2=\frac{1}{3}$
$x=\frac{1}{\sqrt{3}}$
Slant height of the smaller cone = $\frac{12}{\sqrt{3}}$
Slant height of the frustum = $12-\frac{12}{\sqrt{3}}$
= $12*\frac{\sqrt{3}-1}{\sqrt{3}}$
=$12*\frac{(3-\sqrt{3})}{3}$
=$12-4\sqrt{3}$
Therefore, option D is the right answer.
10) Answer (B)
The length of the diagonals of a regular hexagon with side s are $\sqrt{3}s$.
Here length of AC =
$\sqrt{3}s$ = $\sqrt{3}$ cms
Hence area of the square = $\sqrt{3}^2$ = 3 sq cm
Download CAT Previous Papers PDF
Download Free CAT Preparation App
We hope this Geometry Questions PDF for CAT with Solutions will be helpful to you.






![CAT Averages Questions PDF [Important Questions] CAT AVERAGES Questions PDF](https://cracku.in/blog/wp-content/uploads/2022/07/CAT-AVERAGES-Questions-PDF-218x150.png)