DI and LR is one of the most important topics in the Quantitative Ability section of CAT. It is an easy topic and so one must not avoid this topic. You can check out these DI and LR CAT Previous year questions. Practice a good number of questions on CAT DI and LR questions. In this article, we will look into some important DI and LR Questions for CAT. These are a good source for practice; If you want to practice these questions, you can download this CAT DI and LR Questions PDF below, which is completely Free.
Download DI and LR Questions for CAT
Enroll for CAT 2022 Online Course
Instructions
The following table represents addition of two six-digit numbers given in the first and the second rows, while the sum is given in the third row. In the representation, each of the digits 0, 1, 2, 3, 4, 5, 6, 7, 8, 9 has been coded with one letter among A, B, C, D, E, F, G, H, J, K, with distinct letters representing distinct digits.
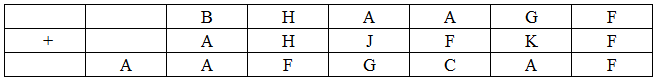
Question 1: Which among the digits 4, 6, 7 and 8 cannot be represented by the letter G?
1) Answer: 6
Solution:
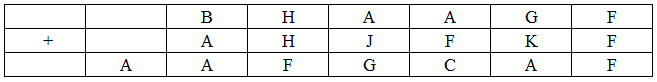
The value of F can only be 0 as F+F=F can only hold if F=0.
Also, A can only be 1(in the second column) because to get a carry of more than 1, B has to be a double-digit number which is not possible. (A carry is a digit that is transferred from one column of digits to another column of more significant digits.)
So the data can be tabulated as follows:
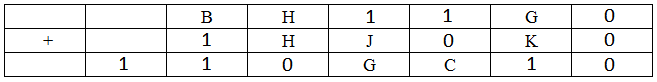
Since the last row in the third column is 0, the carry to the second column must have been 1, Hence B+1+1=11 => B=9
In the 4th column, H+H = 10 since a carry 1 has gone to the 3rd column. Hence H=5.
G+K must be 11 and the carry 1 goes to the next column, so C=1+1=2.
Now, G,K can take values (3,8), (4,7) and (5,6) in any order.
From 5th column G=J+1 => J=G-1
Case: G=3 and K=8, here J =2 which is not possible as C =2
Case: G=8 and K=3, J=7, a possible case.
Case: G=4 and K=7, J=3 possible
Case: G=7 and K=4, J=6 possible
Case: G=5 and K=6, J=4 not possible as H =5.
Case: G=6 and K=5, J=5 both J and K are same, not possible.
Hence the cases can be tabulated as follows:
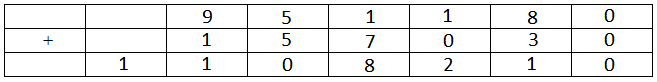
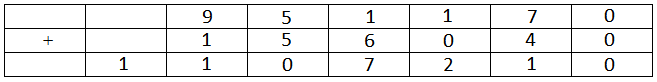
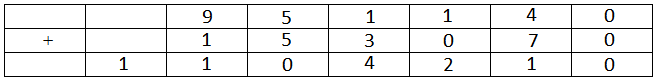
From the table it is clear that 6 cannot be represented by G.
Question 2: Which among the digits 3, 4, 6 and 7 cannot be represented by the letter D?
2) Answer: 7
Solution:

The value of F can only be 0 as F+F=F can only hold if F=0.
Also, A can only be 1(in the second column) because to get a carry of more than 1, B has to be a double-digit number which is not possible. (A carry is a digit that is transferred from one column of digits to another column of more significant digits.)
So the data can be tabulated as follows:

Since the last row in the third column is 0, the carry to the second column must have been 1, Hence B+1+1=11 => B=9
In the 4th column, H+H = 10 since a carry 1 has gone to the 3rd column. Hence H=5.
G+K must be 11 and the carry 1 goes to the next column, so C=1+1=2.
Now, G,K can take values (3,8), (4,7) and (5,6) in any order.
From 5th column G=J+1 => J=G-1
Case: G=3 and K=8, here J =2 which is not possible as C =2
Case: G=8 and K=3, J=7, a possible case.
Case: G=4 and K=7, J=3 possible
Case: G=7 and K=4, J=6 possible
Case: G=5 and K=6, J=4 not possible as H =5.
Case: G=6 and K=5, J=5 both J and K are same, not possible.
Hence the cases can be tabulated as follows:



In all possible cases 7 is already represented by a letter other than D. Hence 7 is the answer.
Question 3: Which digit does the letter B represent?
3) Answer: 9
Solution:

The value of F can only be 0 as F+F=F can only hold if F=0.
Also, A can only be 1(in the second column) because to get a carry of more than 1, B has to be a double-digit number which is not possible. (A carry is a digit that is transferred from one column of digits to another column of more significant digits.)
So the data can be tabulated as follows:

Since the last row in the third column is 0, the carry to the second column must have been 1, Hence B+1+1=11 => B=9
In the 4th column, H+H = 10 since a carry 1 has gone to the 3rd column. Hence H=5.
G+K must be 11 and the carry 1 goes to the next column, so C=1+1=2.
Now, G,K can take values (3,8), (4,7) and (5,6) in any order.
From 5th column G=J+1 => J=G-1
Case: G=3 and K=8, here J =2 which is not possible as C =2
Case: G=8 and K=3, J=7, a possible case.
Case: G=4 and K=7, J=3 possible
Case: G=7 and K=4, J=6 possible
Case: G=5 and K=6, J=4 not possible as H =5.
Case: G=6 and K=5, J=5 both J and K are same, not possible.
Hence the cases can be tabulated as follows:



The letter B represents 9.
Question 4: Which digit does the letter A represent?
4) Answer: 1
Solution:

The value of F can only be 0 as F+F=F can only hold if F=0.
Also, A can only be 1(in the second column) because to get a carry of more than 1, B has to be a double-digit number which is not possible. (A carry is a digit that is transferred from one column of digits to another column of more significant digits.)
So the data can be tabulated as follows:

Since the last row in the third column is 0, the carry to the second column must have been 1, Hence B+1+1=11 => B=9
In the 4th column, H+H = 10 since a carry 1 has gone to the 3rd column. Hence H=5.
G+K must be 11 and the carry 1 goes to the next column, so C=1+1=2.
Now, G,K can take values (3,8), (4,7) and (5,6) in any order.
From 5th column G=J+1 => J=G-1
Case: G=3 and K=8, here J =2 which is not possible as C =2
Case: G=8 and K=3, J=7, a possible case.
Case: G=4 and K=7, J=3 possible
Case: G=7 and K=4, J=6 possible
Case: G=5 and K=6, J=4 not possible as H =5.
Case: G=6 and K=5, J=5 both J and K are same, not possible.
Hence the cases can be tabulated as follows:



The letter A represents 1.
Instructions
_pluB7JG.png)
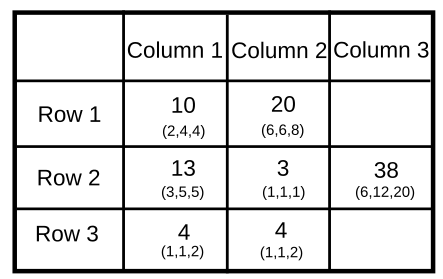
Three pouches (each represented by a filled circle) are kept in each of the nine slots in a 3 × 3 grid, as shown in the figure. Every pouch has a certain number of one-rupee coins. The minimum and maximum amounts of money (in rupees) among the three pouches in each of the nine slots are given in the table. For example, we know that among the three pouches kept in the second column of the first row, the minimum amount in a pouch is Rs. 6 and the maximum amount is Rs. 8.
There are nine pouches in any of the three columns, as well as in any of the three rows. It is known that the average amount of money (in rupees) kept in the nine pouches in any column or in any row is an integer. It is also known that the total amount of money kept in the three pouches in the first column of the third row is Rs. 4.
Question 5: The number of slots for which the total amount in its three pouches strictly exceeds Rs. 10 is
5) Answer: 3
Solution:
We can make the following table from “the total amount of money kept in the three pouches in the first column of the third row is Rs. 4.”
If the minimum and maximum value are 1, then the sum of the three pouches in the middle will be Rs 3.
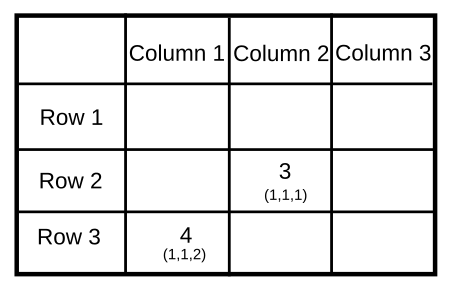
If we calculate the maximum and minimum value possible for each slot in column 1. For the slot, column 1 and row 1, the maximum value possible is 10{2,4,4} while the minimum value possible is 8{2,2,4}.
Similarly, for the slot, column 1 and row 2, the maximum value possible is 13{3,5,5} while the minimum value possible is 11{3,3,5}.
It is known that the average amount of money (in rupees) kept in the nine pouches in any column or in any row is an integer. Thus the sum of coins in a row or column must be a multiple of 9.
So, we can iterate that 10,13,4 …{27} is the only sum possible for the slots of column 1.
We now know two elements of row 2, thus we can iterate from the maximum and the minimum value possible for the slot {cloumn 3, row 2} that 38 is the only value possible for the slot.
We can make the following table:
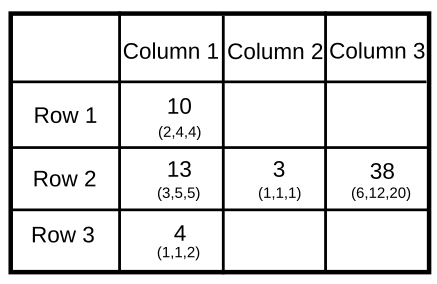
Similarly, we can find the amount for Column 2.
For the slot, column 2 and row 1, the maximum value possible is 22{6,8,8} while the minimum value possible is 20{6,6,8}.
For the slot, column 2 and row 3, the maximum value possible is 5{1,2,3} while the minimum value possible is 4{1,1,2}.
Thus {20,3,4} is the only solution possible.
We can similarly make the following table for the last column.
.png)
Answer 3
Instructions
A farmer had a rectangular land containing 205 trees. He distributed that land among his four daughters – Abha, Bina, Chitra and Dipti by dividing the land into twelve plots along three rows (X,Y,Z) and four Columns (1,2,3,4) as shown in the figure below:
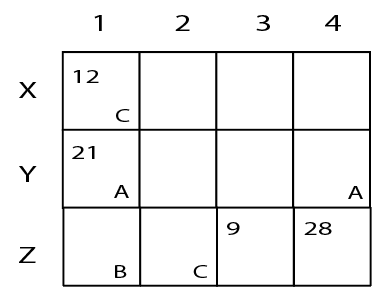
The plots in rows X, Y, Z contained mango, teak and pine trees respectively. Each plot had trees in non-zero multiples of 3 or 4 and none of the plots had the same number of trees. Each daughter got an even number of plots. In the figure, the number mentioned in top left corner of a plot is the number of trees in that plot, while the letter in the bottom right corner is the first letter of the name of the daughter who got that plot (For example, Abha got the plot in row Y and column 1 containing 21 trees). Some information in the figure got erased, but the following is known:
1. Abha got 20 trees more than Chitra but 6 trees less than Dipti.
2. The largest number of trees in a plot was 32, but it was not with Abha.
3. The number of teak trees in Column 3 was double of that in Column 2 but was half of that in Column 4.
4. Both Abha and Bina got a higher number of plots than Dipti.
5. Only Bina, Chitra and Dipti got corner plots.
6. Dipti got two adjoining plots in the same row.
7. Bina was the only one who got a plot in each row and each column.
8. Chitra and Dipti did not get plots which were adjacent to each other (either in row / column / diagonal).
9. The number of mango trees was double the number of teak trees.
Checkout: CAT Free Practice Questions and Videos
Question 6: Which column had the highest number of trees?
a) 4
b) 3
c) Cannot be determined
d) 2
6) Answer (A)
Solution:
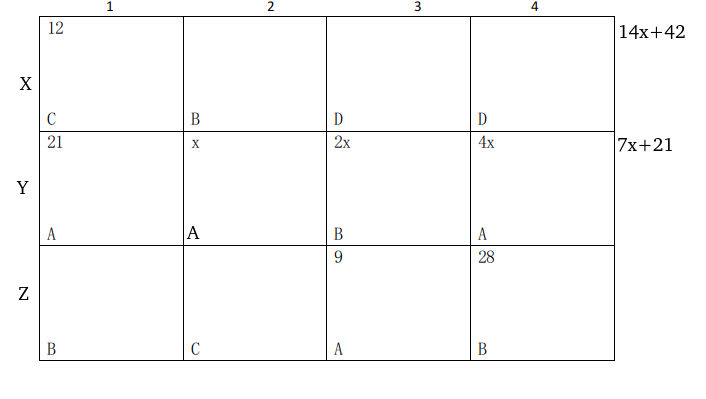
There are 12 plots and each of them got even number of plots. So, possible cases are 4,4,2,2 or 6,2,2,2.
From 4, A and B got more plots than D. So, the only possible case is A, B each got 4 and C,D each got 2.
From 6, D has to get two adjacent plots and From 8, plots of C, D are
nit adjacent to each other => D must have got plots in X3, X4.
C already has two plots in X1, Z2. So, the corner plot Z4 should belong to B.
From 7, B has a plot in each row and each column. So, X2 should belong to B.
Now, out of the remaining Y2, Y3, Y4 and Z3 three plots belong to A and one belongs to B.
Till now B hasn’t got any plot in Third column and 2nd row.
So, Y3 belongs to B and Y2, Y4, Z3 belongs to A.
Let the number of trees in Y4 be 4x from 3, number of trees in Y3, Y2 will be 2x, x respectively.
The number of teak trees=7x+21
.’. Number of mango trees=14x+42
The table now looks like:
Each plot had trees in non-zero multiples of 3 or 4 and none of the
plots had the same number of trees and from 2, B didn’t have the largest
number of trees in a plot => x<8.
x can’t be 7,5,3,2,1 as for these cases at least one of x,2x,4x is neither multiple of 3 or 4.
x can be 6 or 4.
If x=6, number of Teak trees will be 63 and Mango trees will be 126
=> Number of Pine trees= 205-126-63=16 but number of trees in
Z3+Z4>16 so, x$\ne\ $6.
If x=4, Number of Teak trees=49 and Mango trees=98 => Number of Pine trees=58. Valid case.
Number of trees with A= 30+5x=50.
From 1, number of trees with C, D= 30, 56 respectively.
So, number of trees in Z2= 18.
.’. Number of trees with B= 205-50-30-56=69.
From 2, largest number of trees in a plot is 32. They can be in the
plot of either B or D. If they are from B, they have to be from X2 but
in that case number of trees in Z1=1 which is neither a multiple of 3 or
4.
So, highest number of trees in a plot are with D and it is 32 -=> number of trees in X3, X4 are 32, 24 in any order.
So, number of trees in X2= 98-56-12=30
.’. Number of trees in Z1=69-30-28-8=3.
The final table will look like:
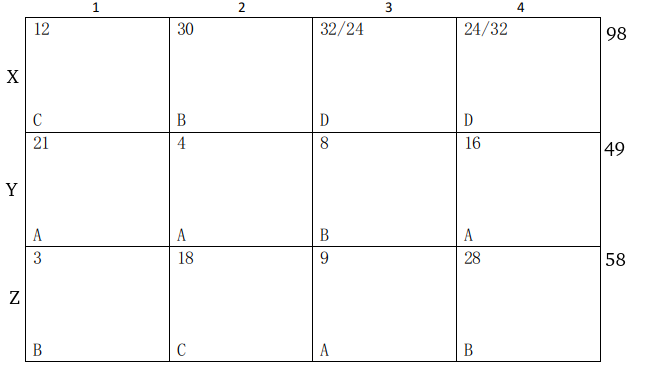
Column 1,2,3,4 have 36, 52, 49, 68 trees respectively.
Hence A is correct answer.
Question 7: Which of the following statements is NOT true?
a) Chitra got 12 mango trees
b) Bina got 32 pine trees.
c) Abha got 41 teak trees.
d) Dipti got 56 mango trees
7) Answer (B)
Solution:
There are 12 plots and each of them got even number of plots. So, possible cases are 4,4,2,2 or 6,2,2,2.
From 4, A and B got more plots than D. So, the only possible case is A, B each got 4 and C,D each got 2.
From 6, D has to get two adjacent plots and From 8, plots of C, D are
nit adjacent to each other => D must have got plots in X3, X4.
C already has two plots in X1, Z2. So, the corner plot Z4 should belong to B.
From 7, B has a plot in each row and each column. So, X2 should belong to B.
Now, out of the remaining Y2, Y3, Y4 and Z3 three plots belong to A and one belongs to B.
Till now B hasn’t got any plot in Third column and 2nd row.
So, Y3 belongs to B and Y2, Y4, Z3 belongs to A.
Let the number of trees in Y4 be 4x from 3, number of trees in Y3, Y2 will be 2x, x respectively.
The number of teak trees=7x+21
.’. Number of mango trees=14x+42
The table now looks like:

Each plot had trees in non-zero multiples of 3 or 4 and none of the
plots had the same number of trees and from 2, B didn’t have the largest
number of trees in a plot => x<8.
x can’t be 7,5,3,2,1 as for these cases at least one of x,2x,4x is neither multiple of 3 or 4.
x can be 6 or 4.
If x=6, number of Teak trees will be 63 and Mango trees will be 126
=> Number of Pine trees= 205-126-63=16 but number of trees in
Z3+Z4>16 so, x$\ne\ $6.
If x=4, Number of Teak trees=49 and Mango trees=98 => Number of Pine trees=58. Valid case.
Number of trees with A= 30+5x=50.
From 1, number of trees with C, D= 30, 56 respectively.
So, number of trees in Z2= 18.
.’. Number of trees with B= 205-50-30-56=69.
From 2, largest number of trees in a plot is 32. They can be in the
plot of either B or D. If they are from B, they have to be from X2 but
in that case number of trees in Z1=1 which is neither a multiple of 3 or
4.
So, highest number of trees in a plot are with D and it is 32 -=> number of trees in X3, X4 are 32, 24 in any order.
So, number of trees in X2= 98-56-12=30
.’. Number of trees in Z1=69-30-28-8=3.
The final table will look like:

Bina got 28 pine trees, Option B is correct answer.
Question 8: Who got the plot with the smallest number of trees and how many trees did that plot have?
a) Dipti, 6 trees
b) Bina, 3 trees
c) Bina, 4 trees
d) Abha, 4 trees
8) Answer (B)
Solution:
There are 12 plots and each of them got even number of plots. So, possible cases are 4,4,2,2 or 6,2,2,2.
From 4, A and B got more plots than D. So, the only possible case is A, B each got 4 and C,D each got 2.
From 6, D has to get two adjacent plots and From 8, plots of C, D are
nit adjacent to each other => D must have got plots in X3, X4.
C already has two plots in X1, Z2. So, the corner plot Z4 should belong to B.
From 7, B has a plot in each row and each column. So, X2 should belong to B.
Now, out of the remaining Y2, Y3, Y4 and Z3 three plots belong to A and one belongs to B.
Till now B hasn’t got any plot in Third column and 2nd row.
So, Y3 belongs to B and Y2, Y4, Z3 belongs to A.
Let the number of trees in Y4 be 4x from 3, number of trees in Y3, Y2 will be 2x, x respectively.
The number of teak trees=7x+21
.’. Number of mango trees=14x+42
The table now looks like:

Each plot had trees in non-zero multiples of 3 or 4 and none of the
plots had the same number of trees and from 2, B didn’t have the largest
number of trees in a plot => x<8.
x can’t be 7,5,3,2,1 as for these cases at least one of x,2x,4x is neither multiple of 3 or 4.
x can be 6 or 4.
If x=6, number of Teak trees will be 63 and Mango trees will be 126
=> Number of Pine trees= 205-126-63=16 but number of trees in
Z3+Z4>16 so, x$\ne\ $6.
If x=4, Number of Teak trees=49 and Mango trees=98 => Number of Pine trees=58. Valid case.
Number of trees with A= 30+5x=50.
From 1, number of trees with C, D= 30, 56 respectively.
So, number of trees in Z2= 18.
.’. Number of trees with B= 205-50-30-56=69.
From 2, largest number of trees in a plot is 32. They can be in the
plot of either B or D. If they are from B, they have to be from X2 but
in that case number of trees in Z1=1 which is neither a multiple of 3 or
4.
So, highest number of trees in a plot are with D and it is 32 -=> number of trees in X3, X4 are 32, 24 in any order.
So, number of trees in X2= 98-56-12=30
.’. Number of trees in Z1=69-30-28-8=3.
The final table will look like:

.’. Number of trees per plot is least for Benna=3.
Question 9: How many pine trees did Chitra receive?
a) 18
b) 30
c) 21
d) 15
9) Answer (A)
Solution:
There are 12 plots and each of them got even number of plots. So, possible cases are 4,4,2,2 or 6,2,2,2.
From 4, A and B got more plots than D. So, the only possible case is A, B each got 4 and C,D each got 2.
From 6, D has to get two adjacent plots and From 8, plots of C, D are
nit adjacent to each other => D must have got plots in X3, X4.
C already has two plots in X1, Z2. So, the corner plot Z4 should belong to B.
From 7, B has a plot in each row and each column. So, X2 should belong to B.
Now, out of the remaining Y2, Y3, Y4 and Z3 three plots belong to A and one belongs to B.
Till now B hasn’t got any plot in Third column and 2nd row.
So, Y3 belongs to B and Y2, Y4, Z3 belongs to A.
Let the number of trees in Y4 be 4x from 3, number of trees in Y3, Y2 will be 2x, x respectively.
The number of teak trees=7x+21
.’. Number of mango trees=14x+42
The table now looks like:

Each plot had trees in non-zero multiples of 3 or 4 and none of the
plots had the same number of trees and from 2, B didn’t have the largest
number of trees in a plot => x<8.
x can’t be 7,5,3,2,1 as for these cases at least one of x,2x,4x is neither multiple of 3 or 4.
x can be 6 or 4.
If x=6, number of Teak trees will be 63 and Mango trees will be 126
=> Number of Pine trees= 205-126-63=16 but number of trees in
Z3+Z4>16 so, x$\ne\ $6.
If x=4, Number of Teak trees=49 and Mango trees=98 => Number of Pine trees=58. Valid case.
Number of trees with A= 30+5x=50.
From 1, number of trees with C, D= 30, 56 respectively.
So, number of trees in Z2= 18.
.’. Number of trees with B= 205-50-30-56=69.
From 2, largest number of trees in a plot is 32. They can be in the
plot of either B or D. If they are from B, they have to be from X2 but
in that case number of trees in Z1=1 which is neither a multiple of 3 or
4.
So, highest number of trees in a plot are with D and it is 32 -=> number of trees in X3, X4 are 32, 24 in any order.
So, number of trees in X2= 98-56-12=30
.’. Number of trees in Z1=69-30-28-8=3.
The final table will look like:

Number of PIne trees received by Chitra = 18.
Question 10: Which of the following is the correct sequence of trees received by Abha, Bina, Chitra and Dipti in that order?
a) 50, 69, 30, 56
b) 54, 57, 34, 60
c) 44, 87, 24, 50
d) 60, 39, 40, 66
10) Answer (A)
Solution:
There are 12 plots and each of them got even number of plots. So, possible cases are 4,4,2,2 or 6,2,2,2.
From 4, A and B got more plots than D. So, the only possible case is A, B each got 4 and C,D each got 2.
From 6, D has to get two adjacent plots and From 8, plots of C, D are
nit adjacent to each other => D must have got plots in X3, X4.
C already has two plots in X1, Z2. So, the corner plot Z4 should belong to B.
From 7, B has a plot in each row and each column. So, X2 should belong to B.
Now, out of the remaining Y2, Y3, Y4 and Z3 three plots belong to A and one belongs to B.
Till now B hasn’t got any plot in Third column and 2nd row.
So, Y3 belongs to B and Y2, Y4, Z3 belongs to A.
Let the number of trees in Y4 be 4x from 3, number of trees in Y3, Y2 will be 2x, x respectively.
The number of teak trees=7x+21
.’. Number of mango trees=14x+42
The table now looks like:

Each plot had trees in non-zero multiples of 3 or 4 and none of the
plots had the same number of trees and from 2, B didn’t have the largest
number of trees in a plot => x<8.
x can’t be 7,5,3,2,1 as for these cases at least one of x,2x,4x is neither multiple of 3 or 4.
x can be 6 or 4.
If x=6, number of Teak trees will be 63 and Mango trees will be 126
=> Number of Pine trees= 205-126-63=16 but number of trees in
Z3+Z4>16 so, x$\ne\ $6.
If x=4, Number of Teak trees=49 and Mango trees=98 => Number of Pine trees=58. Valid case.
Number of trees with A= 30+5x=50.
From 1, number of trees with C, D= 30, 56 respectively.
So, number of trees in Z2= 18.
.’. Number of trees with B= 205-50-30-56=69.
From
2, largest number of trees in a plot is 32. They can be in the plot of
either B or D. If they are from B, they have to be from X2 but in that
case number of trees in Z1=1 which is neither a multiple of 3 or 4.
So, highest number of trees in a plot are with D and it is 32 -=> number of trees in X3, X4 are 32, 24 in any order.
So, number of trees in X2= 98-56-12=30
.’. Number of trees in Z1=69-30-28-8=3.
The final table will look like:

Sequence of trees received by Abha, Bina, Chitra and Dipti is 50,69,30,56.
DI & LR Videos | Preparation Videos for CAT LRDI
- Try these 3 Cracku Free CAT Mocks, which come with detailed solutions and with video explanations.




![CAT Averages Questions PDF [Important Questions] CAT AVERAGES Questions PDF](https://cracku.in/blog/wp-content/uploads/2022/07/CAT-AVERAGES-Questions-PDF-218x150.png)
