Boat and Stream Questions For IBPS PO

Question 1: A boat takes 12 hours to reach point B from point A. It takes 6 hours to reach point A from point B. The river flows from A to B. How much time will it take to travel from A to B if the river remains still?

a) 9 hours

b) 10 hours

c) 8 hours

d) 7.5 hours

e) 10.5 hours

Question 2: A boat develops a leak 18 km from the shore. The ship is still able to move at 12 km/hr and can move only for 25 minutes. A rescue boat which takes 5 minutes to evacuate the crew of the boat leaves the shore at the same time. What should be the minimum speed of the rescue boat to successfully evacuate the crew from the boat in time?

a) 36 kmph

b) 44 kmph

c) 38 kmph

d) 42 kmph

e) 40 kmph

Question 3: There is a river which flows from west to east at a speed of 5 m/s. Arjun, is standing at one bank of the river. He decides to use his motorboat to reach the other bank of the river. His boat travels at a constant speed of 10 m/s. He wants to travel in such a manner that he reaches a point on the other side which is perpendicularly opposite to his starting point. Find the time he takes to reach other side of the bank if the width of the river is 60 meters.

a) $5\sqrt{2}$ seconds

b) $6$ seconds

c) $12$ seconds

d) $4\sqrt{3}$ seconds

e) $6\sqrt{2}$ seconds

IBPS PO Quant Formulas & Shortcuts

IBPS PO Previous papers

Question 4: A boat takes 2 hours to travel from point A to B in still water .To find out it’s speed up-stream ,which of the following information is needed.
i. Distance between point A and B
Ii.Time taken to travel down stream from B to A
iii. Speed of the stream of the water
iv. Effective speed of Boat while traveling Downstream from B to A

a) All are required

b) Even these we cannot found the answer

c) Only i,iii, and either ii or iv

d) Only i and iii

e) None of these

Question 5: The speed of the boat in still water is 24 kmph and the speed of the stream is 41cm/h. The time taken by the boat to travel from A to B downstream is 36 minutes less than the time taken by the same boat to travel from B to’C upstream. If the distance between A and B is 4 km more than the distance between B and C, what is the distance between A and B?

a) 112 km

b) 1401cm

c) 56 km

d) 841cm

e) 281cm

Question 6: A man takes 2.2 times as long to row a distance upstream as to row the same distance downstream. If he can row 55 km downstream in 2 hours 30 minutes, what is the speed of the boat in still water? (in km/h)

a) 40

b) 8

c) 16

d) 24

e) 32

IBPS PO Free Mock Test

Question 7: A boat takes a total time of twelve hours to travel 105 kms upstream and the same distance downstream. The speed of the boat in still water is six times of the speed of the current. What is the speed of the boat in still water? (in km/hr)

a) 12

b) 30

c) 18

d) 24

e) 36

Question 8: A boat takes a total time of eight hours to travel 63 kms upstream and the same distance downstream. The speed of the current is ${1 \over 8}$th of the speed of the boat in still water. What is the speed of the boat in still water? (in km/hr)

a) 32

b) 24

c) 16

d) 8

e) 38

Question 9: A boat takes six hours to travel a certain distance downstream and five hours to travel a certain distance upstream. The distance travelled upstream is half of the travelled downstream. If the speed of the current is 4 km/hr, what is the speed of the boat in still water? (in km/hr)

a) 16

b) 20

c) 24

d) 10

e) 18

IBPS PO Previous Papers (Download Pdf)

Question 10: A boatman can row a boat downstream at 13 kmph and upstream at 9 kmph. What will be the speed of boat in still water ? (in kmph).

a) 12

b) 10.5

c) 11

d) 10

e) 11.5

Question 11: Mukesh swims in a flowing river and takes total 32 minutes to swim from point 1 to point 2 and then again back to point 1. If the distance between point 1 and point 2 is 240 m and Mukesh covers 360 m upstream distance in 30 minutes. What is the speed of the river stream?

a) 5.5 m/min

b) 3.5 m/min

c) 3 m/min

d) 5 m/min

e) 4 m/min

Question 12: Sumit rows downstream from Allahabad to Varanasi in 10 hours. The distance between Allahabad and Varanasi is 180 km and Sumit’s speed in still water is eight times the speed of the stream. Sumit decides to go back to Allahabad along the same route. However, due to strong winds the speed of the stream has increased by 100 percent. If Sumit rows at his earlier speed, then how much time will it take for him to reach Allahabad?

a) 12 hours

b) 20 hours

c) 18 hours

d) 15 hours

e) 24 hours

IBPS PO Important Questions PDF

Question 13: A boat takes 2 hours to travel from point A to B in still water .To find out it’s speed up-stream ,which of the following information is needed.
i. Distance between point A and B
Ii.Time taken to travel down stream from B to A
iii. Speed of the stream of the water
iv. Effective speed of Boat while traveling Downstream from B to A

a) All are required

b) Even these we cannot found the answer

c) Only i,iii, and either ii or iv

d) Only i and iii

e) None of these

Question 14: The speed of the boat in still water is 24 kmph and the speed of the stream is 41cm/h. The time taken by the boat to travel from A to B downstream is 36 minutes less than the time taken by the same boat to travel from B to’C upstream. If the distance between A and B is 4 km more than the distance between B and C, what is the distance between A and B?

a) 112 km

b) 1401cm

c) 56 km

d) 841cm

e) 281cm

Question 15: A man takes 2.2 times as long to row a distance upstream as to row the same distance downstream. If he can row 55 km downstream in 2 hours 30 minutes, what is the speed of the boat in still water? (in km/h)

a) 40

b) 8

c) 16

d) 24

e) 32

Question 16: A boat takes a total time of twelve hours to travel 105 kms upstream and the same distance downstream. The speed of the boat in still water is six times of the speed of the current. What is the speed of the boat in still water? (in km/hr)

a) 12

b) 30

c) 18

d) 24

e) 36

Question 17: A boat takes a total time of eight hours to travel 63 kms upstream and the same distance downstream. The speed of the current is ${1 \over 8}$th of the speed of the boat in still water. What is the speed of the boat in still water? (in km/hr)

a) 32

b) 24

c) 16

d) 8

e) 38

Question 18: A boat takes six hours to travel a certain distance downstream and five hours to travel a certain distance upstream. The distance travelled upstream is half of the travelled downstream. If the speed of the current is 4 km/hr, what is the speed of the boat in still water? (in km/hr)

a) 16

b) 20

c) 24

d) 10

e) 18

Question 19: A boatman can row a boat downstream at 13 kmph and upstream at 9 kmph. What will be the speed of boat in still water ? (in kmph).

a) 12

b) 10.5

c) 11

d) 10

e) 11.5

Question 20: A boat goes downstream at the rate of 9 kmph and upstream at the rate of 6 kmph. Find the speed of current.

a) 1.5 kmph

b) 2 kmph

c) 7.5 kmph

d) 4.5 kmph

e) None of these

Free Banking Study Material (15,000 Solved Questions

Daily Free Banking Online Test

Answers & Solutions:

1) Answer (C)

Let the speed of the boat be ‘b’ and the speed of the stream be ‘s’.
Let the distance between A and B be ‘D’.

We know that D/(b-s) = 12.
D = 12b – 12s ——–(1).

We also know that D/(b+s) = 6.
D = 6b + 6s ———-(2)

Equating (1) and (2), we get,
12b – 12s = 6b + 6s
=> 6b = 18s
b = 3s.
D = 12*3s – 12*s
D = 24s.
Time taken to travel from A to B in still water = D/b = 24s/3s = 8 hours.
Therefore, option C is the right answer.

2) Answer (D)

Distance between Boat and shore = 18 km
The rescue boat must reach the leaking boat in 20 minutes as 5 minutes is needed for evacuation.
Let the relative velocity of the 2 boats be ‘v’
So, v = $\frac{18}{20}$ km per min = 18*60/20 kmph = 54 kmph
So minimum speed of the rescue boat = 54 – 12 = 42 kmph
Hence, option D is correct option.

3) Answer (D)

Since he wants to reach a point which is perpendicularly opposite, he should ensure that the horizontal displacement is zero.

Hence, his horizontal component of the speed should be 5 m/s east to west.

Let’s represent it in a diagram,

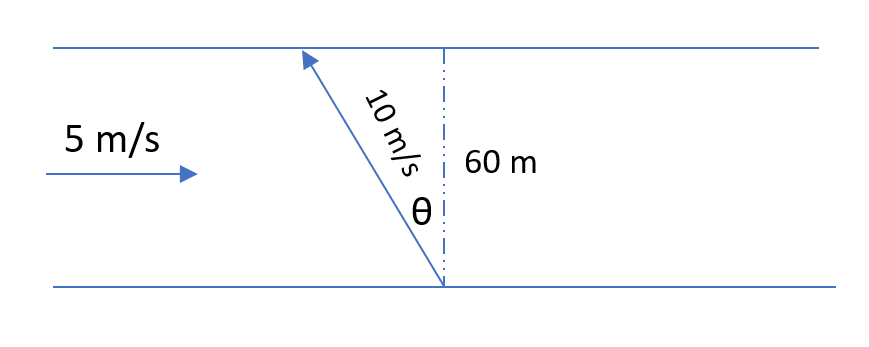
His speed in the horizontal direction = $10 \sin \theta$

His speed in the vertical direction = $10 \cos \theta$

$10 \sin \theta = 5$

Hence, $\theta$ = 30°

His speed in vertical direction = $10 \cos 30$ = $5 \sqrt{3}$

Time taken to cover a vertical distance of 60m = $\frac{60}{5\sqrt{3}}$ = $4\sqrt{3}$ seconds.

4) Answer (D)

Time taken by boat to travel from point A to B in still water = 2 hours

To find the upstream speed, we definitely need the speed of stream, thus statement (iii) is mandatory.

Also, the distance between points A and B or the speed of boat in still water is needed.

Thus, statements (i) and (iii) are required to find the upstream speed of the boat.

=> Ans – (D)

5) Answer (C)

6) Answer (C)

Let speed of boat in still water = $x$ km/hr

=> Speed of current = $y$ km/hr

Let distance travelled = $d$ km

Acc. to ques, => $2.2 (\frac{d}{x + y}) = \frac{d}{x – y}$

=> $2.2x – 2.2y = x + y$

=> $2.2x – x = y + 2.2y$

=> $3x = 8y$ ————-(i)

Also, the man takes 2 hrs 30 mins in travelling 55 km downstream.

=> $\frac{55}{x + y} = 2 + \frac{1}{2}$

=> $\frac{55}{x + y} = \frac{5}{2}$

=> $x + y = 22$

Multiplying both sides by 8, and using eqn(i), we get :

=> $8x + 3x = 22 \times 8$

=> $x = \frac{22 \times 8}{11} = 16$ km/hr

7) Answer (C)

Let speed of current = $x$ km/hr

=> Speed of boat in still water = $6x$ km/hr

Acc. to ques, => $\frac{105}{7x} + \frac{105}{5x} = 12$

=> $\frac{15}{x} + \frac{21}{x} = 12$

=> $\frac{36}{x} = 12$

=> $x = \frac{36}{12} = 3$

$\therefore$ Speed of boat in still water = $6 \times 3 = 18$ km/hr

8) Answer (C)

Let speed of current = $x$ km/hr

=> Speed of boat in still water = $8x$ km/hr

Acc. to ques, => $\frac{63}{9x} + \frac{63}{7x} = 8$

=> $\frac{7}{x} + \frac{9}{x} = 8$

=> $\frac{16}{x} = 8$

=> $x = \frac{16}{8} = 2$

$\therefore$ Speed of boat in still water = $8 \times 2 = 16$ km/hr

9) Answer (A)

Let speed of boat in still water = $x$ km/hr

Let distance travelled downstream = $2d$ km

=> Distance travelled upstream = $d$ km

Using, $time = \frac{distance}{speed}$

=> $6 = \frac{2d}{x + 4}$ ———(i)

and $5 = \frac{d}{x – 4}$ ———(ii)

Dividing eqn(i) from (ii), we get :

=> $\frac{6}{5} = \frac{\frac{2d}{x + 4}}{\frac{d}{x – 4}}$

=> $\frac{6}{5} = \frac{2 (x – 4)}{x + 4}$

=> $6x + 24 = 10x – 40$

=> $10x – 6x = 24 + 40 = 64$

=> $x = \frac{64}{4} = 16$ km/hr

10) Answer (C)

Speed of boat in still water

= $\frac{1}{2}$ (downstream + upstream)

= $\frac{1}{2} (13 + 9) = \frac{22}{2}$

= $11$ kmph

11) Answer (E)

Let the speed of Mukesh and river be ‘m’ and ‘r’ respectively.
We have, $\frac{240}{m+r} + \frac{240}{m-r} = 32$
Also, $\frac{360}{m-r} = 30$
m – r = 12 m/min
Thus we get, $\frac{240}{m+r} + \frac{240}{12} = 32$
m + r = 20 m/min
Solving both the equations we get,
m = 16 m/min
r = 4 m/min
Hence, option E is the right choice.

12) Answer (D)

It took total of 10 hours to travel 180 km. Hence the effective speed was 18 km/hr. Let the speed of the stream was ‘x’. So the speed of the Sumit will be 8x.
Hence, 9x = 18
=> x = 2
Now while coming back the speed of the stream has doubled. So it has become 4. He will now be rowing upstream. So effective speed will be 16 – 4 = 12
Hence, required time = 180/12 = 15 hours

13) Answer (D)

Time taken by boat to travel from point A to B in still water = 2 hours

To find the upstream speed, we definitely need the speed of stream, thus statement (iii) is mandatory.

Also, the distance between points A and B or the speed of boat in still water is needed.

Thus, statements (i) and (iii) are required to find the upstream speed of the boat.

=> Ans – (D)

14) Answer (C)

15) Answer (C)

Let speed of boat in still water = $x$ km/hr

=> Speed of current = $y$ km/hr

Let distance travelled = $d$ km

Acc. to ques, => $2.2 (\frac{d}{x + y}) = \frac{d}{x – y}$

=> $2.2x – 2.2y = x + y$

=> $2.2x – x = y + 2.2y$

=> $3x = 8y$ ————-(i)

Also, the man takes 2 hrs 30 mins in travelling 55 km downstream.

=> $\frac{55}{x + y} = 2 + \frac{1}{2}$

=> $\frac{55}{x + y} = \frac{5}{2}$

=> $x + y = 22$

Multiplying both sides by 8, and using eqn(i), we get :

=> $8x + 3x = 22 \times 8$

=> $x = \frac{22 \times 8}{11} = 16$ km/hr

16) Answer (C)

Let speed of current = $x$ km/hr

=> Speed of boat in still water = $6x$ km/hr

Acc. to ques, => $\frac{105}{7x} + \frac{105}{5x} = 12$

=> $\frac{15}{x} + \frac{21}{x} = 12$

=> $\frac{36}{x} = 12$

=> $x = \frac{36}{12} = 3$

$\therefore$ Speed of boat in still water = $6 \times 3 = 18$ km/hr

17) Answer (C)

Let speed of current = $x$ km/hr

=> Speed of boat in still water = $8x$ km/hr

Acc. to ques, => $\frac{63}{9x} + \frac{63}{7x} = 8$

=> $\frac{7}{x} + \frac{9}{x} = 8$

=> $\frac{16}{x} = 8$

=> $x = \frac{16}{8} = 2$

$\therefore$ Speed of boat in still water = $8 \times 2 = 16$ km/hr

18) Answer (A)

Let speed of boat in still water = $x$ km/hr

Let distance travelled downstream = $2d$ km

=> Distance travelled upstream = $d$ km

Using, $time = \frac{distance}{speed}$

=> $6 = \frac{2d}{x + 4}$ ———(i)

and $5 = \frac{d}{x – 4}$ ———(ii)

Dividing eqn(i) from (ii), we get :

=> $\frac{6}{5} = \frac{\frac{2d}{x + 4}}{\frac{d}{x – 4}}$

=> $\frac{6}{5} = \frac{2 (x – 4)}{x + 4}$

=> $6x + 24 = 10x – 40$

=> $10x – 6x = 24 + 40 = 64$

=> $x = \frac{64}{4} = 16$ km/hr

19) Answer (C)

Speed of boat in still water

= $\frac{1}{2}$ (downstream + upstream)

= $\frac{1}{2} (13 + 9) = \frac{22}{2}$

= $11$ kmph

20) Answer (A)

Speed of current = $\frac{1}{2}$ (downstream – upstream)

= $\frac{1}{2} (9 – 6)$

= $\frac{3}{2} = 1.5$ kmph

 

IBPS PO Previous Papers (Download PDF)

Download IBPS PO Free Preparation App