Triangles Questions for NMAT:

Download Triangles Questions for NMAT PDF – NMAT Classification questions pdf by Cracku. Top 10 very important Triangles Questions for NMAT based on asked questions in previous exam papers.

Download Triangles Questions for NMAT

Get 5 NMAT Mocks for Rs. 499

Take NMAT mock test

Question 1: One side of an equilateral triangle is 24 cm. The midpoints of its sides are joined to form another triangle whose midpoints are in turn joined to form still another triangle. This process continues indefinitely. Find the sum of the perimeters of all the triangles.

a) 144 cm

b) 72 cm

c) 536 cm

d) 676 cm

Question 2: The figure below shows two right angled triangles ∆OAB and ∆OQP with right angles at vertex A and P, respectively, having the common vertex O, The lengths of some of the sides are indicated in the figure. (Note that the figure is not drawn to scale.) AB and OP are parallel.
What is ∠QOB?

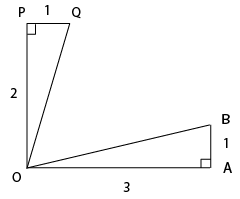
a) $tan^{-1}(2/3)$

b) $45°$

c) $30°$

d) $tan^{-1}(3/2)$

e) $60°$

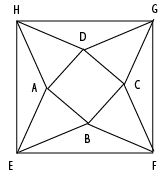
Question 3: In the picture below, EFGH, ABCD are squares, and ABE, BCF, CDG, DAH are equilateral triangles. What is the ratio of the area of the square EFGH to that of ABCD?

a) $\sqrt{3}+2$

b) $\sqrt{2}+\sqrt{3}$

c) $1+\sqrt{3}$

d) $\sqrt{2}+2$

e) $3+\sqrt{2}$

Question 4: Given an equilateral triangle T1 with side 24 cm, a second triangle T2 is formed by joining the midpoints of the sides of T1. Then a third triangle T3 is formed by joining the midpoints of the sides of T2. If this process of forming triangles is continued, the sum of the areas, in sq cm, of infinitely many such triangles T1, T2, T3,… will be

a) $188\sqrt{3}$

b) $248\sqrt{3}$

c) $164\sqrt{3}$

d) $192\sqrt{3}$

Question 5: Eight points lie on the circumference of a circle. The difference between the number of triangles and the number of quadrilaterals that can be formed by connecting these points is

a) 7

b) 14

c) 32

d) 84

Question 6: In a square of side 2 meters, isosceles triangles of equal area are cut from the corners to form a regular octagon. Find the perimeter and area of the rectangular octagon.

a) $\frac{16}{2+\sqrt{2}};\frac{4(1+\sqrt{2})}{3+2\sqrt{2}}$

b) $\frac{8}{2+\sqrt{2}};\frac{2(1+\sqrt{2})}{3+2\sqrt{2}}$

c) $\frac{16}{1+\sqrt{2}};\frac{3(1+\sqrt{2})}{3+2\sqrt{2}}$

d) None of these

Question 7: $\triangle$ABC and $\triangle$XYZ are equilateral triangles of 54 cm sides. All smaller triangles like $\triangle$ANM, $\triangle$OCP, $\triangle$QPX etc. are also equilateral triangles. Find the area of the shape MNOPQRM.

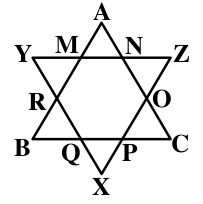
a) 243√3 sq. cm.

b) 486√3 sq. cm.

c) 729√3 sq. cm.

d) 4374√3 sq. cm.

e) None of the above

Question 8: Ten points are marked on a straight line and eleven points are marked on another straight line. How many triangles can be constructed with vertices from among the above points?

a) 495

b) 550

c) 1045

d) 2475

Question 9: What is the number of distinct triangles with integral valued sides and perimeter 14?

a) 6

b) 5

c) 4

d) 3

Question 10: Consider obtuse-angled triangles with sides 8 cm, 15 cm and x cm. If x is an integer then how many such triangles exist?

a) 5

b) 21

c) 10

d) 15

e) 14

Join 7K MBA Aspirants Telegram Group

Download Highly Rated CAT preparation App

Answers & Solutions:

1) Answer (A)

Side of an equilateral triangle= 24 cm

The mid-points of its sides are joined to form another triangle whose mid-points are joined to form another triangle. This process continues indefinitely.

Side of 2nd equilateral triangle=12 cm [ Line segment joining midpoint of two sides of a triangle is parallel to the third side and half of it.]

Similarly, Side of third equilateral triangle=6 cm

Sides are 3, 1.5,3/4,….

Perimeter of 1st triangle=24+24+24=72 cm

Perimeter of 2nd triangle=12+12+12=36 cm

Perimeter of 3rd triangle=6+6+6=18 cm

……….

The sum of the perimeter of all the triangles=72+36+18+9+9/2+9/4+……..

This is a geometric progression having a common ratio =36/72=1/2

Sum of an infinite G.P=

= 72 × 2

=144 which is the sum of the perimeter of all triangles.

A is the correct answer.

2) Answer (B)

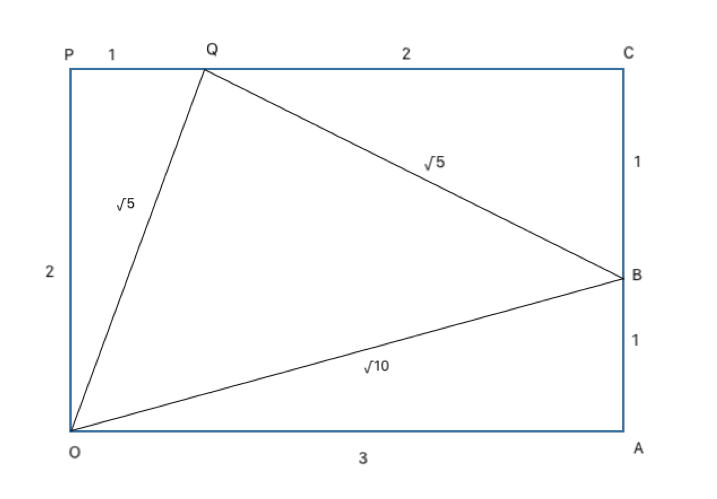
The figure can be redrawn as shown above.

$\because $ AB and OP are parallel, POAC is a rectangle.

In right $\triangle$QCB, QB = $\sqrt{5}$

$\triangle$QBO is an isosceles right angled triangle with $\angle $OQB = $ 90°$ (By Pythagoras theorem)

$\therefore $ $\angle $QOB =  $45°$

3) Answer (A)

Let the sides of the smaller square be $x$ units and the sides of the larger square be $y$ units.

Construct diagonal HF on the larger square. Let the point of intersection of AD and diagonal be I and point of intersection of BC and diagonal be J.

We know that since EFGH is a square, the diagonal will bisect the angle. Therefore $\angle EHF=45^{o}$

Observe $\triangle AEH$ and $\triangle DHG$ :

AE=AH=DH=DG = $x$ units and EH=HG=$y$ units.

Thus by SSS property, $\triangle AEH \cong \triangle DHG$

Thus, we know that $\angle$AHE=$\angle$DHG

Also, $\angle$AHE+$\angle$DHG+$\angle$AHD= $90^{o}$ (angle of a square)

$ \Rightarrow (2 \times \angle AHE)+ 60^{o} = 90^{o}$ (angle of an equilateral triangle)

$ \Rightarrow (2 \times \angle AHE) = 30^{o}$

$ \Rightarrow \angle AHE = 15^{o}$

Since $\angle AHE = 15^{o}, \angle AHI = 45^{o}-15^{o} = 30^{o} $

Since $30^{o}= \dfrac{60^{o}}{2}$ we can say that HF is the angle bisector of $\angle$AHD

Since $\triangle$AHD is an equilateral triangle, we know that the angle bisector, median and altitude will all be the same line ie HI in this case.

By symmetry, we know that JF will also be the angle bisector, median and altitude.

Also, length of altitude of equilateral triangle ie HI and JF = $\dfrac{\sqrt{3} \times x}{2} $

Length of diagonal HF = $\sqrt{2} \times y$

From the figure, we can express the length of diagonal HF as given :

$ \sqrt{2} \times y = \dfrac{\sqrt{3} \times x}{2} + x + \dfrac{\sqrt{3} \times x}{2} $

$ \Rightarrow \sqrt{2} \times y = (\sqrt{3} \times x) + x $

$ \Rightarrow \sqrt{2} \times y = x \times (\sqrt{3} + 1) $

$ \Rightarrow y = x \times (\dfrac{\sqrt{3} + 1}{\sqrt{2}}) $

Ratio of areas asked = $(\dfrac{y}{x})^{2} = (\dfrac{\sqrt{3} + 1}{\sqrt{2}})^{2} = \dfrac{4+2\sqrt{3}}{2} = 2+\sqrt{3}$

Alternate Explanation: 

Let AB=BC=CD=DA=x, then the area the area of square ABCD will be $x^2$

Since AHD, DGC, CFB, and BAE are equilateral triangles with side x, the sum of area of these traingles will be $4\times\ \frac{\sqrt{\ 3}}{4}\times\ x^2=\sqrt{\ 3}x^2$

We know that angle HAD=60, angle DAB=90, and angle BAE=60, thus angle HAE=360-(60+90+60)=150

Area of triangles HAE+HDG+GCF+FBE=4* area of triangle HAE

In HAE, AH=AE=x, and angle HAE=150, thus area will be $\frac{1}{2}\times\ x\times\ x\times\ \sin\ 150$

sin 150=sin(180-150)=sin 30=0.5

Thus area of 4 triangles will be 4*0.5*x*x*0.5=$x^2$

Thus, total area= $x^2+\sqrt{\ 3\ }\ x^2+x^2$=$x^2\left(2+\sqrt{\ 3}\right)$

Thus area of EFGH/area of ABCD=$2+\sqrt{\ 3}$

4) Answer (D)

We can see that T$_{2}$ is formed by using the mid points of T$_{1}$. Hence, we can say that area of triangle of T$_{2}$ will be (1/4)th of the area of triangle T$_{1}$.

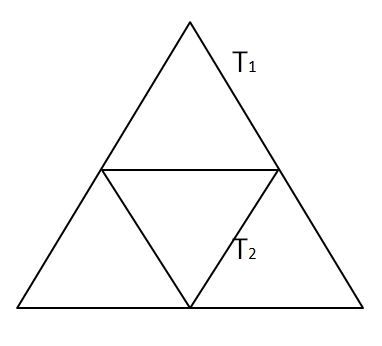
Area of triangle T$_{1}$ = $\dfrac{\sqrt{3}}{4}*(24)^2$ = $144\sqrt{3}$ sq. cm

Area of triangle T$_{2}$ = $\dfrac{144\sqrt{3}}{4}$ = $36\sqrt{3}$ sq. cm

Sum of the area of all triangles =  T$_{1}$ + T$_{2}$ + T$_{3}$ + …

$\Rightarrow$ T$_{1}$ + T$_{1}$/$4$ + T$_{1}$/$4^2$ + …

$\Rightarrow$ $\dfrac{T_{1}}{1 – 0.25}$

$\Rightarrow$ $\dfrac{4}{3}*T_{1}$

$\Rightarrow$ $\dfrac{4}{3}*144\sqrt{3}$

$\Rightarrow$ $192\sqrt{3}$

Hence, option D is the correct answer.

5) Answer (B)

The number of quadrilaterals that can be made using any 4 points out of total 8 points on the given circle = $^8C_4$ = 70
The number of triangle that can be made using any 4 points out of total 8 points on the given circle = $^8C_3$ = 56
Thus, the required difference = 70-56 = 14
Hence, option B is the correct answer.

6) Answer (D)

Let us assume that ‘a’ is the length of the side of equal sides of the isosceles triangle.

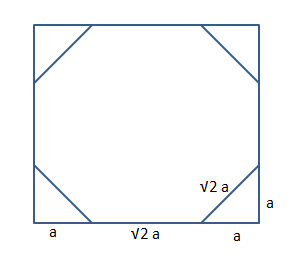
We can see that $a+\sqrt{2}*a+a=2$

$\Rightarrow$ a = $\dfrac{2}{2+\sqrt{2}}$

Therefore, the perimeter of the regular octagon = $8*a*\sqrt{2}$

$\Rightarrow$ $\dfrac{8\sqrt{2}*2}{2+\sqrt{2}}$

$\Rightarrow$ $\dfrac{16}{1+\sqrt{2}}$ … (1)

Area of regular octagon = $2x^2(1+\sqrt{2})$ where ‘x’ is the length of each side.

Here, x = $a\sqrt{2}$ = $\dfrac{2\sqrt{2}}{2+\sqrt{2}}$ = $\dfrac{2}{1+\sqrt{2}}$

Therefore, area of the regular octagon = $2*(\dfrac{2}{1+\sqrt{2}})^2(1+\sqrt{2})$ = $\dfrac{8}{1+\sqrt{2}}$ =$\dfrac{8}{1+\sqrt{2}}*\dfrac{1+\sqrt{2}}{1+\sqrt{2}}$ = $\dfrac{8*(1+\sqrt{2})}{3+2\sqrt{2}}$

Hence, we can say that none of the given answer is correct.

7) Answer (B)

AB = 54 cm and $\triangle$ANM , $\triangle$OCP , $\triangle$OPX are equilateral triangles.

=> MN = MR = NO = OP = PQ = QR = $\frac{54}{3} = 18$ cm

Thus,  MNOPQRM is a regular hexagon with side 18 cm

$\therefore$ Area of  MNOPQRM = $\frac{3 \sqrt{3}}{2} (side)^2$

= $\frac{3 \sqrt{3}}{2} \times 18^2 = 486 \sqrt{3} cm^2$

8) Answer (C)

For a triangle to be formed, we need three points.

Case 1: Select 2 points on the line that has 10 points and 1 point on the line that ha 11 points.

This can be done in $^{10}C_2$*$^{11}C_1$ ways = 495 ways.

Case 2: Select 2 points on the line that has 11 points and 1 point on the line that ha 10 points.

This can be done in $^{11}C_2$*$^{10}C_1$ ways = 550 ways.

495 + 550 = 1045 ways.

9) Answer (C)

Let the sides be x, y and 14-(x+y)
x+y > 14-(x+y) => x+y > 7
x+14-x-y > y => y < 7
Similarly, x < 7
If x = 1, y = 7 (not possible)
So, if x = 2, y = 6
if x = 3, y = 5
if x = 4, y = 4, 5

The cases for x = 5 and 6 are already taken care of by y.
Number of possible cases = 4

10) Answer (C)

For obtuse-angles triangle, $c^2 > a^2 + b^2$ and c < a+b
If 15 is the greatest side, 8+x > 15 => x > 7 and $225 > 64 + x^2$ => $x^2$ < 161 => x <= 12
So, x = 8, 9, 10, 11, 12
If x is the greatest side, then 8 + 15 > x => x < 23
$x^2 > 225 + 64 = 289$ => x > 17
So, x = 18, 19, 20, 21, 22
So, the number of possibilities is 10

We hope these Triangle Questions for NMAT pdf for the NMAT exam will be highly useful for your Preparation.