Number System Questions for TISSNET 2022 – Download PDF

Here you can download TISSNET 2022 Number System [PDF] by Cracku. Very Important Number System Questions for TISSNET 2022 based on asked questions in previous exam papers. These questions will help your TISSNET exam preparation. So kindly download the PDF for reference and do more practice.

Download Number System Questions for TISSNET

Get 5 TISSNET mocks at just Rs.299

Download TISSNET previous papers PDF

Question 1: In a Green view apartment, the houses of a row are numbered consecutively from 1 to 49. Assuming that there is a value of ‘x’ such that the sum of the numbers of the houses preceding the house numbered ‘x’ is equal to the sum of the numbers of the houses following it. Then what will be the value of ‘x’?

a) 21

b) 30

c) 35

d) 42

Question 2: How many ‘zeroes’ are there in the following sequence which are immediately preceded by a nine but not immediately followed by seven?
7090070890702030045703907

a) One

b) Two

c) Three

d) Four

Question 3: Arrange the following letters to form a meaningful word.

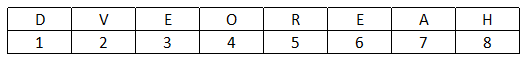
a) 1 3 5 2 4 6 8 7

b) 8 6 7 1 4 2 3 5

c) 4 2 3 5 8 6 7 1

d) 5 3 7 1 8 4 2 6

Question 4: There are four prime numbers written in ascending order of magnitude. The product of the first three is 7429 and last three is 12673. Find the first number.

a) 19

b) 17

c) 13

d) None of the above

Question 5: In the following series, what numbers should replace the question marks?
-1, 0, 1, 0, 2, 4, 1, 6, 9, 2, 12, 16, ? ? ?

a) 11, 18, 27

b) -1, 0, 3

c) 3, 20, 25

d) Cannot be ascertained

Download TISSNET Previous Papers PDF

Question 6: If k is an integer and $0.0010101\times10^{k}$ is greater than 1000, what is the least possible value of k?

a) 4

b) 5

c) 6

d) 7

Question 7: In 2011, Plasma – a pharmaceutical company – allocated $Rs.4.5 \times 10^{7}$ for Research and Development. In 2012, the company allocated Rs.60,000,000 for Research and Development. If each year the funds are evenly divided among $2 \times 10^{2}$ departments, how much more will each department receive this year than it did last year?

a) $Rs.2.0 \times 10^{5}$

b) $Rs.7.5 \times 10^{5}$

c) $Rs.7.5 \times 10^{4}$

d) $Rs.2.5 \times 10^{7}$

Question 8: Z is the product of first 31 natural numbers. If X = Z + 1, then the numbers of primes among X + 1, X + 2, …, X + 29, X + 30 is

a) 30

b) 2

c) Cannot be determined

d) None of the above

Question 9: A sum of Rs.1400 is divided amongst A, B, C and D such that A’s share : B’s share = B’s share : C’s share = C’s share = D’s share = $\frac{3}{4}$ how much is C’s share?

a) Rs.72

b) Rs.288

c) Rs.216

d) Rs.384

Question 10: If the product of the integers a, b, c and d is 3094 and if 1 < a < b < c < d, what is the product of b and c?

a) 26

b) 91

c) 133

d) 221

Question 11: A rod is cut into 3 equal parts. The resulting portions are then cut into 12, 18 and 32 equal parts, respectively. If each of the resulting portions have integer length, the minimum length of the rod is

a) 6912 units

b) 864 units

c) 288 units

d) 240 units

Question 12: The sum of $1 – \frac{1}{6} + (\frac{1}{6} \times \frac{1}{4})-(\frac{1}{6} \times \frac{1}{4} \times \frac{5}{18})+$ …. is

a) $\frac{2}{3}$

b) $\frac{2}{\sqrt{3}}$

c) $\sqrt{\frac{2}{3}}$

d) $\frac{\sqrt{3}}{2}$

Question 13: $2-\frac{\sqrt{6407522209}}{\sqrt{3600840049}}=$

a) 0.666039

b) 0.666029

c) 0.666009

d) None of the above

Question 14: If $N = (11^{p + 7})(7^{q – 2})(5^{r + 1})(3^{s})$ is a perfect cube, where $p, q, r$ and $s$ are positive integers, then the smallest value of $p + q + r + s$ is :

a) 5

b) 6

c) 7

d) 8

e) 9

Question 15: X and Y are the digits at the unit’s place of the numbers (408X) and (789Y) where X ≠ Y. However, the digits at the unit’s place of the numbers $(408X)^{63}$ and $(789Y)^{85}$ are the same. What will be the possible value(s) of (X + Y)?

a) 9

b) 10

c) 11

d) 12

e) None of the above

Question 16: Find the value of the expression: $10 + 10^3 + 10^6 + 10^9$

a) 1010101010

b) 1001000010

c) 1001000110

d) 1001001010

e) 100010001010

Question 17: If the sum of squares of two numbers is 97, then which one of the following cannot be their product?

a) -32

b) 16

c) 48

d) 64

Question 18: If one third of one-fourth of a number is 15, then three-tenth of that number is

a) 61

b) 54

c) 46

d) 38

Question 19: The number of integers between 300 and 1100 which are divisible by either 7 or 13 but not both is

a) 149

b) 158

c) 167

d) 176

Question 20: How many zeros would be there in $1024!$

a) 240

b) 248

c) 256

d) 253

Take TISSNET Mock Tests

Answers & Solutions:

1) Answer (C)

It is given that sum of the first $(x – 1)$ numbers is equal to sum of the numbers from $(x + 1)$ to 49
or, sum of $(x – 1)$ numbers = sum of first 49 numbers – sum of first $x$ numbers
$\dfrac{x(x – 1)}{2} = \dfrac{49 * 50}{2} – \dfrac{x(x + 1)}{2}$
On solving, we get $x$ = 35
Hence, option C is the correct answer.

2) Answer (A)

We can see that only one zero present in the number such that it is immediately preceded by a nine but not immediately followed by seven. It is shown in round braces. 4th from the left.
709(0)070890702030045703907

Therefore, option A is the correct answer.

3) Answer (C)

OVERHEAD is the word that can be formed from the given letters.
Hence, option C is the correct answer.

4) Answer (B)

Let ‘a’, ‘b’, ‘c’ and ‘d’ be the prime number in ascending order. The product of the first three is 7429 and last three is 12673. Find the first number.

Given that $a*b*c =  7429$ and $b*c*d =  12673$

Therefore, $\dfrac{b*c*d}{a*b*c} = \dfrac{12673}{7429}$

$\Rightarrow$ $\dfrac{d}{a} = \dfrac{29}{17}$

Since a and d are prime number there won’t be any common factor which can divide both. Hence, a = 17, b = 19, c = 23 and d = 29.

Therefore, option B is the correct answer.

5) Answer (C)

The given series is a combination of 3 series:-
1st containing all 3n+1 terms i.e. 1st, 4th, 7th and so on terms:-
-1, 0, 1, 2 and thus, the next number will be 3.
2nd containing all 3n+2 terms i.e. 2nd, 5th, 8th and so on terms:-
0, 2, 6, 12 as we can see 2n is being added to each term to get the next term and thus the next term will be 20.
3rd containing all 3n terms i.e. 3rd, 6th, 9th and so on terms:-
1, 4, 9, 16 an we can see these are squares of the natural numbers and thus, the next term will be 25.
Hence, ?,?,? will be 3, 20, 25
Hence, option C is the correct answer.

6) Answer (C)

0.0010101 to make it greater than 1000 we will have to shift the decimal point by 6 places.
Thus, we will have to multiply the given number by $10^6$.
Hence, option C is the correct answer.

7) Answer (C)

Total amount allotted in 2011 = 4.5*10$^7$
Total number of departments = 200
Thus, the amount received by each department = 225000
Total amount allotted in 2012 = 60000000
The amount received by each department = 300000
Thus the excess of amount each department is getting = 300000 – 225000 = 750000  = Rs. 7.5*10$^4$
Hence, option C is the correct answer.

8) Answer (D)

It is given that Z = 31!
X = 31! + 1
X+1 = 31!+2 this is divisible by 2
X+2 = 31!+3 this is divisible by 3
X+3 = 31!+4 this is divisible by 4
.
.
.
.
X+29 = 31!+30 this is divisible by 30
X+30 = 31!+31 this is divisible by 31
Hence, none of X + 1, X + 2, …, X + 29, X + 30 is a prime number.
Hence, option D is the correct answer.

9) Answer (D)

A:B = B:C = C:D = 3:4
Let C be 3x and D be 4x
B : C = 3 : 4 and C = 3x So, B = 9x/4
A : B = 3 : 4 and B = 9x/4 So, A = 27x/16
Therefore, A : B : C : D is 27x/16 : 9x/4 : 3x : 4x
Also, A + B + C + D = Rs.  1400
On solving, we get x = 128
Therefore, C’s share = 3x = Rs. 384
Hence, option D is the correct answer.

10) Answer (B)

3094 = 2*7*13*17
Given, 1 < a < b < c < d
Thus, a = 2, b = 7, c = 13 and d = 17
Hence, b*c = 7*13 = 91
Hence, option B is the correct answer.

11) Answer (B)

The rod is cut into 3 equal parts thus the length of the rod will be a multiple of 3.
Each part is then cut into $12 = 2^2*3$
$18 = 2*3^2$ and $32 = 2^5$ parts and thus, each part of rod has to be a multiple of $2^5*3^2 = 288$
Thus, the rod will be a multiple of $288*3 = 864$
Thus, the minimum length of the rod is 864 units.
Hence, option B is the correct answer.

12) Answer (D)

We can see that the magnitude in each succeeding term is less than that of preceding term.

Hence, we can say that for S = $1 – \frac{1}{6} + (\frac{1}{6} \times \frac{1}{4})-(\frac{1}{6} \times \frac{1}{4} \times \frac{5}{18})+$ …

The value will lie between (5/6, 1). We can check with option choices.

Option A: $\frac{2}{3}$ < $\frac{5}{6}$. Hence, this can’t be the answer.

Option B: $\frac{2}{\sqrt{3}}$ = 1.155 > $1$. Hence, this can’t be the answer.

Option C: $\sqrt{\frac{2}{3}}$ = 0.8164 < $\frac{5}{6}$. Hence, this can’t be the answer.

Option D: $\frac{\sqrt{3}}{2}$ = 0.866. This lies between (5/6, 1).Hence, this is the correct answer.

13) Answer (A)

$2-\frac{\sqrt{6407522209}}{\sqrt{3600840049}}=2-\frac{80047}{60007}$
=$2-1.3339610$
$=0.666039$
Therefore, option A is the right answer.

14) Answer (E)

It has been given that $N = (11^{p + 7})(7^{q – 2})(5^{r + 1})(3^{s})$ is a perfect cube. All the factors given are prime. Therefore, the power of each number should be a multiple of 3 or 0.

$p,q,r$ and $s$ are positive integers. Therefore, only the power of the expressions in which some number is subtracted from these variables or these variables are subtracted from some number can be made $0$.

$11^{p + 7}$:

This expression must be made a perfect cube. The nearest perfect cube is $11^9$. Therefore, the least value that $p$ can take is $9-7=2$.

$7^{q – 2}$

The least value that $q$ can take is 2. If $q=2$, then the value of the expression $7^{q-2}$ will become $7^0=1$, without preventing the product from becoming a perfect cube.

$5^{r+1}$:

The least value that $r$ can take is $2$.

$3^{s})$:

The least value that $s$ can take is $3$.

Therefore, the least value of the expression $p + q + r + s$ is $2+2+2+3=9$.
Therefore, option E is the right answer.

15) Answer (B)

All numbers from 1 to 9 repeat their last digits over a cycle of 4.
63 can be written as 4k+3.
85 can be written as 4k+1.
Some number’s third power should yield the first digit as some number’s first power.
$2^3$ will yield $8$ as the last digit (2 and 8 is a possible solution).X+Y = 10
$3^3$ will yield $7$ as the last digit (3 and 7 is a possible solution).X + Y = 10
$4^3$ will yield $4$ as the last digit and hence, can be eliminated.
$5$ and $6$ yield $5$ and $6$ respectively as the last digit for any power and hence, can be eliminated.
$7^3$ will yield $3$ as the last digit (7 and 3 is a possible solution). X+Y=10.
$8^3$ will yield $2$ as the last digit. 8+2 =10.
As we can see, X+Y = 10 in all the cases. Therefore, option B is the right answer.

16) Answer (D)

$10 + 10^3 + 10^6 + 10^9 = 10 + 1000 + 1000000 + 1000000000$
=$1001001010$
Therefore, option D is the right answer.

17) Answer (D)

Let ‘a’ and ‘b’ are those two numbers.

$\Rightarrow$ $a^2+b^2 = 97$

$\Rightarrow$ $a^2+b^2-2ab = 97-2ab$

$\Rightarrow$ $(a-b)^2 = 97-2ab$

We know that $(a-b)^2$ $\geq$ 0

$\Rightarrow$ 97-2ab $\geq$ 0

$\Rightarrow$ ab $\leq$ 48.5

Hence, ab $\neq$ 64. Therefore, option D is the correct answer.

18) Answer (B)

Let the number be ‘x’

one-fourth of the number = $\frac{1}{4}\times x = \frac{x}{4}$

one-third of one-fourth of the number = $\frac{1}{3}\times \frac{x}{4} = \frac{x}{12}$

Given, one-third of one-fourth of the number is = 15

$\Rightarrow \frac{x}{12} = 15$

$\Rightarrow x = 180$

Hence the number is 180.

Then three-tenth of the number = $\frac{3}{10}\times x = \frac{3}{10}\times 180 = 54$

19) Answer (B)

Multiples of 7 are {301,308 …………………………..1099} n=115

Multiples of 13 are {312,323,……………………………1092} n = 61

Multiples of 91 are {364,455……………………………1092} n= 9

Number of integers which are divisible by either 7 or 13 but not both is 115+61-2*9

=158

Hence B is the correct answer.

20) Answer (D)

The number of zeros in n! = highest power of 5 in n!

Highest power of 5 in 1024! = [$\frac{1024}{5}$] + [$\frac{1024}{25}$] +[$\frac{1024}{125}$] + [$\frac{1024}{625}$] where [ ]is the greatest integer function.

Highest power of 5 in 1024! = 204+ 40+ 8+ 1 = 253

Enroll to CAT 2022 Complete course

Download TISSNET Previous Papers PDF