Complete Modern Maths Previous Questions (PYQs 2017-22) [Download PDF]
Modern Mathematics is a vital component of the Quantitative Aptitude section in the CAT (Common Admission Test) exam. Aspiring CAT candidates understand the importance of conquering this section to excel in the test. If you’re starting your CAT preparation journey and aiming to tackle Modern Mathematics, you’ve come to the right place. In this blog post, we’re thrilled to present an extensive collection of Modern Mathematics questions from the CAT papers of 2017 to 2022. These questions are not just practice exercises; they are actual CAT questions meticulously chosen to provide you with a glimpse of what you might encounter on the big day.
Modern Mathematics in CAT can seem complex, but with the right guidance and practice, you can master it. Our blog will simplify the intricacies of CAT Modern Mathematics by diving into Previous Year Questions (PYQs) from 2017 to 2022. We’ll offer detailed step-by-step solutions and expert insights, ensuring that you not only solve the problems but also build the confidence to handle any Modern Mathematics question that the CAT exam throws at you.
Prepare to explore the world of CAT Modern Mathematics, equip yourself with effective tools and strategies, and embark on a successful journey toward CAT achievement. Let’s get started!
Download Complete Modern Maths Previous Questions (CAT PYQs 2017-22)
Check out here for Detailed video solutions for Complete Modern Maths CAT PYQs (2017-22)
Question 1:Â Suppose, $\log_3 x = \log_{12} y = a$, where $x, y$ are positive numbers. If $G$ is the geometric mean of x and y, and $\log_6 G$ is equal to
a)Â $\sqrt{a}$
b)Â 2a
c)Â a/2
d)Â a
1) Answer (D)
Solution:
We know that $\log_3 x = a$ and $\log_{12} y=a$
Hence, $x = 3^a$ and $y=12^a$
Therefore, the geometric mean of $x$ and $y$ equals $\sqrt{x \times y}$
This equals $\sqrt{3^a \times 12^a} = 6^a$
Hence, $G=6^a$ Or, $\log_6 G = a$
Question 2:Â The value of $\log_{0.008}\sqrt{5}+\log_{\sqrt{3}}81-7$ is equal to
a)Â 1/3
b)Â 2/3
c)Â 5/6
d)Â 7/6
2) Answer (C)
Solution:
$\log_{0.008}\sqrt{5}+\log_{\sqrt{3}}81-7$
$81 = 3^4$ and $0.008 = \frac{8}{1000} = \frac{2^{3}}{10^{3}} = \frac{1}{5^{3}} = 5^{-3} $
Hence,
$\log_{0.008}\sqrt{5}+ 8 -7 $
$ \log_{5^{-3}}5^{\frac{1}{2}}+ 8 -7 $
$\frac{log 5^{0.5}}{log 5^{-3}} + 1$
$ – \frac{1}{6} + 1$
= $\frac{5}{6}$
Question 3:Â If $9^{2x-1}-81^{x-1}=1944$, then $x$ is
a)Â 3
b)Â 9/4
c)Â 4/9
d)Â 1/3
3) Answer (B)
Solution:
$\frac{81^x}{9} – \frac{81^x}{81} = 1944$
$81^x * [\frac{1}{9}- \frac{1}{81}] = 1944$
$81^x * [\frac{1}{81}] = 243$
$3^{4x} = 3^9$
$x = \frac{9}{4}$
Question 4:Â Let AB, CD, EF, GH, and JK be five diameters of a circle with center at 0. In how many ways can three points be chosen out of A, B, C, D, E, F, G, H, J, K, and O so as to form a triangle?
4)Â Answer:Â 160
Solution:
The total number of given points are 11. (10 on circumference and 1 is the center)
So total possible triangles = 11C3 = 165.
However, AOB, COD, EOF, GOH, JOK lie on a straight line. Hence, these 5 triangles are not possible. Thus, the required number of triangles = 165 – 5 = 160
Question 5:Â If the square of the 7th term of an arithmetic progression with positive common difference equals the product of the 3rd and 17th terms, then the ratio of the first term to the common difference is
a)Â 2:3
b)Â 3:2
c)Â 3:4
d)Â 4:3
5) Answer (A)
Solution:
The seventh term of an AP = a + 6d. Third term will be a + 2d and second term will be a + 16d. We are given that
$ (a + 6d)^2 = (a + 2d)(a + 16d)$
=> $ a^2 $ + $36d^2$ +Â 12ad = $ a^2 + 18ad + 32d^2 $
=> $4d^2 = 6ad$
=> $ d:a = 3:2$
We have been asked about a:d. Hence, it would be 2:3
Question 6:Â In how many ways can 7 identical erasers be distributed among 4 kids in such a way that each kid gets at least one eraser but nobody gets more than 3 erasers?
a)Â 16
b)Â 20
c)Â 14
d)Â 15
6) Answer (A)
Solution:
We have been given that a + b + c + d = 7
Total ways of distributing 7 things among 4 people so that each one gets at least one = $^{n-1}C_{r-1}$ = 6C3 = 20
Now we need to subtract the cases where any one person got more than 3 erasers. Any person cannot get more than 4 erasers since each child has to get at least 1. Any of the 4 childs can get 4 erasers. Thus, there are 4 cases. On subtracting these cases from the total cases we get the required answer. Hence, the required value is 20 – 4 = 16
Question 7: Let $a_1$, $a_2$,…………., $a_{3n}$ be an arithmetic progression with $a_1$ = 3 and $a_{2}$ = 7. If $a_1$+ $a_{2}$ +…+ $a_{3n}$= 1830, then what is the smallest positive integer m such that m($a_1$+ $a_{2}$ +…+ $a_n$) > 1830?
a)Â 8
b)Â 9
c)Â 10
d)Â 11
7) Answer (B)
Solution:
$a_{1}$ = 3 and $a_{2}$ = 7. Hence, the common difference of the AP is 4. If we assume, k=3n
We have been given that the sum up to 3n terms of this AP is 1830. Hence, $1830 = \frac{k}{2}[2*3 + (k – 1)*4$
=> 1830*2 = k(6 + 4k – 4)
=> 3660 = 2k + 4k$^2$
=> $2k^2 + k – 1830 = 0$
=> (k – 30)(2k + 61) = 0
=> k = 30 or k = -61/2
Since k is the number of terms so k cannot be negative. Hence, must be 30
So, 3n = 30
n = 10
Sum of the first ’10’ terms of the given AP = 5*(6 + 9*4) = 42*5 = 210
m($a_1$+Â $a_{2}$Â +…+ $a_n$) > 1830
=> 210m >Â 1830
=> m > 8.71
Hence, smallest integral value of ‘m’ is 9.
Question 8:Â The numbers 1, 2, …, 9 are arranged in a 3 X 3 square grid in such a way that each number occurs once and the entries along each column, each row, and each of the two diagonals add up to the same value.
If the top left and the top right entries of the grid are 6 and 2, respectively, then the bottom middle entry is
8)Â Answer:Â 3
Solution:
According to the question each column, each row, and each of the two diagonals of the 3X3 matrix add up to the same value. This value must be 15.
Let us consider the matrix as shown below:
Now we’ll try substituting values from 1 to 9 in the exact middle grid shown as ‘x’.
If x = 1 or 3, then the value in the left bottom grid will be more than 9 which is not possible.
x cannot be equal to 2.
If x = 4, value in the left bottom grid will be 9. But then addition of first column will come out to be more than 15. Hence, not possible.
If x=5, we get the grid as shown below:
Hence, for x = 5 all conditions are satisfied. We see that the bottom middle entry is 3.
Hence, 3 is the correct answer.
Question 9:Â If x is a real number such that $\log_{3}5= \log_{5}(2 + x)$, then which of the following is true?
a)Â 0 < x < 3
b)Â 23 < x < 30
c)Â x > 30
d)Â 3 < x < 23
9) Answer (D)
Solution:
$1 < \log_{3}5 < 2$
=> $ 1 < \log_{5}(2 + x) < 2 $
=> $ 5 < 2 + x < 25$
=> $ 3 < x < 23$
Question 10:Â If $9^{x-\frac{1}{2}}-2^{2x-2}=4^{x}-3^{2x-3}$, then $x$ is
a)Â 3/2
b)Â 2/5
c)Â 3/4
d)Â 4/9
10) Answer (A)
Solution:
It is given that $9^{x-\frac{1}{2}}-2^{2x-2}=4^{x}-3^{2x-3}$
Let us try to reduce them to powers of $3$ and $2$
The given equation can be reduced to $3^{2x-1} + 3^{2x-3} = 2^{2x} + 2^{2x-2}$
Hence, $3^{2x-3} \times 10 = 2^{2x-2} \times 5$
Therefore, $3^{2x-3} = 2^{2x-3}$
This is possible only if $2x-3=0$ or $x=3/2$
Question 11:Â If $log(2^{a}\times3^{b}\times5^{c} )$is the arithmetic mean of $log ( 2^{2}\times3^{3}\times5)$, $log(2^{6}\times3\times5^{7} )$, and $log(2 \times3^{2}\times5^{4} )$, then a equals
11)Â Answer:Â 3
Solution:
$log(2^{a}\times3^{b}\times5^{c} )$ = $ \frac{log ( 2^{2}\times3^{3}\times5) + log(2^{6}\times3\times5^{7} ) + log(2 \times3^{2}\times5^{4} ) }{3} $
$log(2^{a}\times3^{b}\times5^{c} )$ = $ \frac{log ( 2^{2+6+1}\times3^{3+1+2}\times5^{1+7+4}) }{3} $
$log(2^{a}\times3^{b}\times5^{c} )$ = $ \frac{log ( 2^{9}\times3^{6}\times5^{12}) }{3} $
$3log(2^{a}\times3^{b}\times5^{c} )$ = $ log ( 2^{9}\times3^{6}\times5^{12}) $
Hence, 3a = 9 or a = 3
Question 12:Â Let $a_{1},a_{2},a_{3},a_{4},a_{5}$ be a sequence of five consecutive odd numbers. Consider a new sequence of five consecutive even numbers ending with $2a_{3}$
If the sum of the numbers in the new sequence is 450, then $a_{5}$ is
12)Â Answer:Â 51
Solution:
Sum of the sequence of even numbers is $2a_{3} + (2a_{3} – 2) + (2a_{3} – 4)$ $+ (2a_{3} – 6) + (2a_{3} – 8) = 450$
=> $10a_{3} – 20 = 450$
=> $a_{3} = 47$
Hence $a_{5} = 47 + 4 = 51$
Question 13:Â In how many ways can 8 identical pens be distributed among Amal, Bimal, and Kamal so that Amal gets at least 1 pen, Bimal gets at least 2 pens, and Kamal gets at least 3 pens?
13)Â Answer:Â 6
Solution:
After Amal, Bimal and Kamal are given their minimum required pens, the pens left are 8 – (1 + 2 + 3) = 2 pens
Now these two pens have to be divided between three persons so that each person can get zero pens = $^{2+3-1}C_{3-1}$ = $^4C_2$Â = 6
Question 14:Â How many four digit numbers, which are divisible by 6, can be formed using the digits 0, 2, 3, 4, 6, such that no digit is used more than once and 0 does not occur in the left-most position?
14)Â Answer:Â 50
Solution:
For the number to be divisible by 6, the sum of the digits should be divisible by 3 and the units digit should be even. Hence we have the digits as
Case I: 2, 3, 4, 6
Now the units place can be filled in three ways (2,4,6), and the remaining three places can be filled in 3! = 6 ways.
Hence total number of ways = 3*6 = 18
Case II: 0, 2, 3, 4
case II a: 0 is in the units place => 3! = 6 ways
case II b: 0 is not in the units place => units place can be filled in 2 ways( 2,4) , thousands place can be filled in 2 ways ( remaining 3 – 0) and remaining can be filled in 2! = 2 ways. Hence total number of ways = 2 * 2 * 2 = 8
Total number of ways in this case = 6 + 8 = 14 ways.
Case III: 0, 2, 4, 6
case III a: 0 is in the units place => 3! = 6 ways
case II b: 0 is not in the units place => units place can be filled in 3 ways( 2,4,6) , thousands place can be filled in 2 ways (remaining 3 – 0) and remaining can be filled in 2! = 2 ways. Hence total number of ways = 3 * 2 * 2 = 12
Total number of ways in this case = 6 + 12= 18 ways.
Hence the total number of ways = 18 + 14Â + 18 = 50 ways
Question 15:Â An infinite geometric progression $a_1,a_2,…$ has the property that $a_n= 3(a_{n+1}+ a_{n+2} + …)$ for every n $\geq$ 1. If the sum $a_1+a_2+a_3…+=32$, then $a_5$ is
a)Â 1/32
b)Â 2/32
c)Â 3/32
d)Â 4/32
15) Answer (C)
Solution:
Let the common ratio of the G.P. be r.
Hence we have $a_n= 3(a_{n+1}+ a_{n+2} + …)$
The sum up to infinity of GP is given by $\frac{a}{1-r}$ where a here is $a_{n+1}$
=> $a_n= 3(\frac{a_{n+1}}{1-r})$
=> $a_n= 3(\frac{a_{n}\times r}{1-r})$
=> $ r = \frac{1}{4}$
Now, $a_1+a_2+a_2…+=32$
=> $\frac{a_1}{1-r} = 32$
=> $\frac{a_1}{3/4} = 32$
=> $a_1 = 24$
$a_5 = a_1 \times r^4$
$a_5 = 24 \times (1/4)^4 = \frac{3}{32}$
Question 16:Â If $a_{1}=\frac{1}{2\times5},a_{2}=\frac{1}{5\times8},a_{3}=\frac{1}{8\times11},…,$ then $a_{1}+a_{2}+a_{3}+…+a_{100}$ is
a)Â $\frac{25}{151}$
b)Â $\frac{1}{2}$
c)Â $\frac{1}{4}$
d)Â $\frac{111}{55}$
16) Answer (A)
Solution:
$a_{100} = \frac{1}{ (3\times100 -1) \times (3\times100 + 2)}= \frac{1}{ 299 \times 302}$
$\frac{1}{2\times5} = \frac{1}{3} \times (\frac{1}{2} – \frac{1}{5})$
$\frac{1}{5\times8} = \frac{1}{3} \times (\frac{1}{5} – \frac{1}{8})$
$\frac{1}{8\times11} = \frac{1}{3} \times (\frac{1}{8} – \frac{1}{11})$
….
$\frac{1}{299\times302} = \frac{1}{3} \times (\frac{1}{299} – \frac{1}{302})$
Hence $a_{1}+a_{2}+a_{3}+…+a_{100}$ = $\frac{1}{3} \times (\frac{1}{2} – \frac{1}{5})$ + $\frac{1}{3} \times (\frac{1}{5} – \frac{1}{8})$ + $\frac{1}{3} \times (\frac{1}{8} – \frac{1}{11})$ + … + $\frac{1}{3} \times (\frac{1}{299} – \frac{1}{302})$
= $\frac{1}{3} \times (\frac{1}{2} – \frac{1}{302})$
= $\frac{25}{151}$
Question 17:Â If x is a positive quantity such that $2^{x}=3^{\log_{5}{2}}$. then x is equal to
a)Â $\log_{5}{8}$
b)Â $1+\log_{3}({\frac{5}{3}})$
c)Â $\log_{5}{9}$
d)Â $1+\log_{5}({\frac{3}{5}})$
17) Answer (D)
Solution:
Givne that:Â $2^{x}=3^{\log_{5}{2}}$
$\Rightarrow$ $2^{x}=2^{\log_{5}{3}}$
$\Rightarrow$Â $x=\log_{5}{3}$
$\Rightarrow$Â $x=\log_{5}{\dfrac{3*5}{5}}$
$\Rightarrow$Â $x=\log_{5}{5}+\log_{5}{\dfrac{3}{5}}$
$\Rightarrow$Â $x=1+\log_{5}{\dfrac{3}{5}}$. Hence, option D is the correct answer.
Question 18:Â If $\log_{12}{81}=p$, then $3(\dfrac{4-p}{4+p})$ is equal to
a)Â $\log_{4}{16}$
b)Â $\log_{6}{16}$
c)Â $\log_{2}{8}$
d)Â $\log_{6}{8}$
18) Answer (D)
Solution:
Given that:Â $\log_{12}{81}=p$
$\Rightarrow$Â $\log_{81}{12}=\dfrac{1}{p}$
$\Rightarrow$Â $\log_{3}{3*4}=\dfrac{4}{p}$
$\Rightarrow$Â $1+\log_{3}{4}=\dfrac{4}{p}$
Using Componendo and Dividendo,
$\Rightarrow$ $\dfrac{1+\log_{3}{4}-1}{1+\log_{3}{4}+1}=\dfrac{4-p}{4+p}$
$\Rightarrow$Â $\dfrac{\log_{3}{4}}{2+\log_{3}{4}}=\dfrac{4-p}{4+p}$
$\Rightarrow$Â $\dfrac{\log_{3}{4}}{\log_{3}{9}+\log_{3}{4}}=\dfrac{4-p}{4+p}$
$\Rightarrow$Â $\dfrac{\log_{3}{4}}{\log_{3}{36}}=\dfrac{4-p}{4+p}$
$\Rightarrow$Â $3*\dfrac{4-p}{4+p}=\dfrac{3\log_{3}{4}}{\log_{3}{36}}$
$\Rightarrow$Â $3*\dfrac{4-p}{4+p}=\dfrac{\log_{3}{64}}{\log_{3}{36}}$
$\Rightarrow$Â $3*\dfrac{4-p}{4+p}=\log_{36}{64}$
$\Rightarrow$Â $3*\dfrac{4-p}{4+p}=\log_{6^2}{8^2}=\log_{6}{8}$. Hence, option D is the correct answer.
Question 19:Â How many numbers with two or more digits can be formed with the digits 1,2,3,4,5,6,7,8,9, so that in every such number, each digit is used at most once and the digits appear in the ascending order?
19)Â Answer:Â 502
Solution:
It has been given that the digits in the number should appear in the ascending order. Therefore, there is only 1 possible arrangement of the digits once they are selected to form a number.
There are 9 numbers (1,2,3,4,5,6,7,8,9) in total.
2 digit numbers can be formed in $9C_2$ ways.
3 digit numbers can be formed in $9C_3$ ways.
…………………………………………..
..9 digit number can be formed in 9C9 ways.
We know that $nC_0+nC_1+nC_2+………+nC_n =2^n$
=> $9C_0 + 9C_1+9C_2+….9C_9 = 2^9$
$9C_0 + 9C_1 + …9C_9 = 512$
We have to subtract $9C_0$ and $9C_1$ from both the sides of the equations since we cannot form single digit numbers.
=> $9C_2 + 9C_3+…+9C_9=512-1-9$
$9C_2+9C_3+…+9C_9=502$
Therefore, $502$ is the right answer.
Question 20:Â Each of 74 students in a class studies at least one of the three subjects H, E and P. Ten students study all three subjects, while twenty study H and E, but not P. Every student who studies P also studies H or E or both. If the number of students studying H equals that studying E, then the number of students studying H is
20)Â Answer:Â 52
Solution:
Let us draw a Venn diagram using the information present in the question.
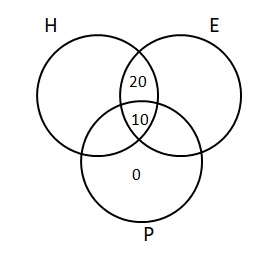
It is given that the number of students studying H equals that studying E.
Let ‘x’ be the total number of students who studied H, and H and P but mot E.We can also say that the same will be the number of students who studied E, and E and P but not H.Therefore,
x +Â 20 + 10 + x = 74
x = 22
Hence, the number of students studying H = 22 + 10+ 20 = 52
Question 21:Â If among 200 students, 105 like pizza and 134 like burger, then the number of students who like only burger can possibly be
a)Â 23
b)Â 26
c)Â 96
d)Â 93
21) Answer (D)
Solution:
It has been given that among 200 students, 105 like pizza and 134 like burger.
The question asks us to find out the number of students who can be liking only burgers among the given values.
The least number of students who like only burger will be obtained when everyone who likes pizza likes burger too.
In this case, 105 students will like pizza and burger and 134-105 = 29 students will like only burger. Therefore, the number of students who like only burger cannot be less than 29.
The maximum number of students who like only burger will be obtained when we try to separate the 2 sets as much as possible.
There are 200 students in total. 105 of them like pizza. Therefore, the remaining 95 students can like only burger and 134-95 = 39 students can like both pizza and burger. As we can see, the number of students who like burger cannot exceed 95.
The number of students who like only burger should lie between 29 and 95 (both the values are included).
93 is the only value among the given options that satisfies this condition and hence, option D is the right answer.
Question 22:Â Let x, y, z be three positive real numbers in a geometric progression such that x < y < z. If 5x, 16y, and 12z are in an arithmetic progression then the common ratio of the geometric progression is
a)Â $\frac{3}{6}$
b)Â $\frac{1}{6}$
c)Â $\frac{5}{2}$
d)Â $\frac{3}{2}$
22) Answer (C)
Solution:
Let x = $a$, y = $ar$ and z = $ar^2$
It is given that, 5x, 16y and 12z are in AP.
so, 5x + 12z = 32y
On replacing the values of x, y and z, we get
$5a + 12ar^2 = 32ar$
or, $12r^2 – 32r + 5$ = 0
On solving, $r$ = $\frac{5}{2}$ or $\frac{1}{6}$
For $r$ =Â $\frac{1}{6}$, x < y < z is not satisfied.
So, $r$ = $\frac{5}{2}$
Hence, option C is the correct answer.
Question 23:Â Given that $x^{2018}y^{2017}=\frac{1}{2}$, and $x^{2016}y^{2019}=8$, then value of $x^{2}+y^{3}$ is
a)Â $\dfrac{31}{4}$
b)Â $\dfrac{35}{4}$
c)Â $\dfrac{37}{4}$
d)Â $\dfrac{33}{4}$
23) Answer (D)
Solution:
Given that $x^{2018}y^{2017}=\frac{1}{2}$Â … (1)
$x^{2016}y^{2019}=8$ … (2)
Equation (2)/Â Equation (1)
$\dfrac{y^2}{x^2} = \dfrac{8}{1/2}$
$\dfrac{y}{x} = 4$ or $-4$
Case 1: When $\dfrac{y}{x} = 4$
$x^{2018}(4x)^{2017}=\dfrac{1}{2}$
$x^{2018+2017}(2)^{4034}=\dfrac{1}{2}$
$x^{4035}=\dfrac{1}{(2)^{4035}}$
$x=\dfrac{1}{2}$
Since, $\dfrac{y}{x} = 4$, => y = 2
Therefore, $x^{2}+y^{3}$ = $\dfrac{1}{4}+8$ = $\dfrac{33}{4}$
Case 2: When $\dfrac{y}{x} = -4$
$x^{2018}(-4x)^{2017}=\dfrac{1}{2}$
$x^{2018+2017}(2)^{4034}=\dfrac{-1}{2}$
$x^{4035}=\dfrac{1}{(-2)^{4035}}$
$x=\dfrac{-1}{2}$
Since, $\dfrac{y}{x} = -4$, => y = 2
Therefore, $x^{2}+y^{3}$ = $\dfrac{1}{4}+8$ = $\dfrac{33}{4}$. Hence, option D is the correct answer.
Question 24:Â If $\log_{2}({5+\log_{3}{a}})=3$ and $\log_{5}({4a+12+\log_{2}{b}})=3$, then a + b is equal to
a)Â 59
b)Â 40
c)Â 32
d)Â 67
24) Answer (A)
Solution:
$\log_{2}({5+\log_{3}{a}})=3$
=>$5 +Â \log_{3}{a}$ = 8
=>$Â \log_{3}{a}$ = 3
or $a$ = 27
$\log_{5}({4a+12+\log_{2}{b}})=3$
=>$4a+12+\log_{2}{b}$ = 125
Putting $a$ = 27, we get
$\log_{2}{b}$ = 5
or, $b$ = 32
So, $a + b$ = 27 + 32 = 59
Hence, option A is the correct answer.
Question 25:Â How many two-digit numbers, with a non-zero digit in the units place, are there which are more than thrice the number formed by interchanging the positions of its digits?
25)Â Answer:Â 6
Solution:
Let ‘ab’ be the two digit number. Where b $\neq$ 0.
We will get number ‘ba’ after interchanging its digit.
It is given that 10a+b > 3*(10b + a)
7a > 29b
If b = 1, then a = {5, 6, 7, 8, 9}
If b = 2, then a = {9}
If b = 3, then no value of ‘a’ is possible. Hence, we can say that there are a total of 6 such numbers.
Question 26: For two sets A and B, let AΔB denote the set of elements which belong to A or B but not both. If P = {1,2,3,4}, Q = {2,3,5,6,}, R = {1,3,7,8,9}, S = {2,4,9,10}, then the number of elements in (PΔQ)Δ(RΔS) is
26)Â Answer:Â 7
Solution:
P = {1,2,3,4} and Q = {2,3,5,6,}
PΔQ = {1, 4, 5, 6}
R = {1,3,7,8,9} and S = {2,4,9,10}
RΔS = {1, 2, 3, 4, 7, 8, 10}
(PΔQ)Δ(RΔS) = {2, 3, 5, 6, 7, 8, 10}
Thus, there are 7 elements in (PΔQ)Δ(RΔS) .
hence, 7 is the correct answer.
Question 27:Â In a tournament, there are 43 junior level and 51 senior level participants. Each pair of juniors play one match. Each pair of seniors play one match. There is no junior versus senior match. The number of girl versus girl matches in junior level is 153, while the number of boy versus boy matches in senior level is 276. The number of matches a boy plays against a girl is
27)Â Answer:Â 1098
Solution:
In a tournament, there are 43 junior level and 51 senior level participants.
Let ‘n’ be the number of girls on junior level. It is given that the number of girl versus girl matches in junior level is 153.
$\Rightarrow$ nC2 = 153
$\Rightarrow$ n(n-1)/2 = 153
$\Rightarrow$ n(n-1) = 306
=> n$^{2}$-n-306 = 0
=> (n+17)(n-18)=0
=> n=18Â (rejecting n=-17)
Therefore, number of boys on junior level = 43 – 18 = 25.
Let ‘m’ be the number of boys on senior level. It is given that the number of boy versus boy matches in senior level is 276.
$\Rightarrow$ mC2 = 276
$\Rightarrow$ m = 24
Therefore, number of girls on senior level = 51 – 24 = 27.
Hence, the number of matches a boy plays against a girl = 18*25+24*27 = 1098
Question 28:Â Let $\ a_{1},a_{2}…a_{52}\ $ be positive integers such that $\ a_{1}$ < $a_{2}$ < … <Â $a_{52}\ $. Suppose, their arithmetic mean is one less than arithmetic mean of $a_{2}$, $a_{3}$, ….$a_{52}$. If $a_{52}$= 100, then the largest possible value of $a_{1}$is
a)Â 48
b)Â 20
c)Â 23
d)Â 45
28) Answer (C)
Solution:
Let ‘x’ be the average of all 52 positive integers $\ a_{1},a_{2}…a_{52}\ $.
$a_{1}+a_{2}+a_{3}+…+a_{52}$ = 52x … (1)
Therefore, average of $a_{2}$, $a_{3}$, ….$a_{52}$ = x+1
$a_{2}+a_{3}+a_{4}+…+a_{52}$ = 51(x+1)Â … (2)
From equation (1) and (2), we can say that
$a_{1}+51(x+1)$ =Â 52x
$a_{1}$ = x – 51.
We have to find out the largest possible value of $a_{1}$. $a_{1}$ will be maximum when ‘x’ is maximum.
(x+1) is the average of terms $a_{2}$, $a_{3}$, ….$a_{52}$. We know that $a_{2}$ < $a_{3}$ < … < $a_{52}\ $ and $a_{52}$ = 100.
Therefore, (x+1) will be maximum when each term is maximum possible. If $a_{52}$ = 100, then $a_{52}$ = 99, $a_{50}$ = 98 ends so on.
$a_{2}$ = 100 + (51-1)*(-1) = 50.
Hence, Â $a_{2}+a_{3}+a_{4}+…+a_{52}$ = 50+51+…+99+100 =Â 51(x+1)
$\Rightarrow$ $\dfrac{51*(50+100)}{2} =Â 51(x+1)$
$\Rightarrow$ $x =Â 74$
Therefore, the largest possible value of $a_{1}$ = x – 51 = 74 – 51 = 23.
Question 29:Â The value of the sum 7 x 11 + 11 x 15 + 15 x 19 + …+ 95 x 99 is
29)Â Answer:Â 80707
Solution:
S = 7 x 11 + 11 x 15 + 15 x 19 + …+ 95 x 99
Nth term of the series can be written as $T_{n} = (4n+3)*(4n+7)$
Last term, (4n+3) = 95 i.e. n = 23
$\sum_{n=1}^{n=23} (4n+3)*(4n+7)$
$\Rightarrow$ $\sum_{n=1}^{n=23}16n^2+40n+21$
$\Rightarrow$Â $16*\dfrac{23*24*47}{6}+40*\dfrac{23*24}{2}+21*23$
$\Rightarrow$Â $80707$
Question 30:Â If N and x are positive integers such that $N^{N}$ = $2^{160}\ and \ N{^2} + 2^{N}\ $ is an integral multiple of $\ 2^{x}$, then the largest possible x is
30)Â Answer:Â 10
Solution:
It is given that $N^{N}$ = $2^{160}$
We can rewrite the equation as $N^{N}$ = $(2^5)^{160/5}$ =Â $32^{32}$
$\Rightarrow$ N = 32
$N{^2} + 2^{N}$ = $32^2+2^{32}=2^{10}+2^{32}=2^{10}*(1+2^{22})$
Hence, we can say that $N{^2} + 2^{N}$ can be divided by $2^{10}$
Therefore, x$_{max}$ = 10
Question 31: The arithmetic mean of x, y and z is 80, and that of x, y, z, u and v is 75, where u=(x+y)/2 and v=(y+z)/2. If x ≥ z, then the minimum possible value of x is
31)Â Answer:Â 105
Solution:
Given that the arithmetic mean of x, y and z is 80.
$\Rightarrow$ $\dfrac{x+y+z}{3} = 80$
$\Rightarrow$ $x+y+z = 240$Â … (1)
Also,  $\dfrac{x+y+z+v+u}{5} = 75$
$\Rightarrow$ $\dfrac{x+y+z+v+u}{5} = 75$
$\Rightarrow$Â $x+y+z+v+u = 375$
Substituting values from equation (1),
$\Rightarrow$Â $v+u = 135$
It is given that u=(x+y)/2 and v=(y+z)/2.
$\Rightarrow$Â $(x+y)/2+(y+z)/2 = 135$
$\Rightarrow$ $x+2y+z = 270$
$\Rightarrow$ $y = 30$  (Since $x+y+z = 240$)
Therefore, we can say that $x+z = 240 – y = 210$. We are also given that x ≥ z,
Hence, $x_{min}$ = 210/2 = 105.
Question 32:Â If A = {$6^{2n} -35n – 1$}, where $n$ = 1,2,3,… and B = {35($n$-1)}, where $n$ = 1,2,3,… then which of the following is true?
a)Â Every member of A is in B and at least one member of B is not in A
b)Â Neither every member of A is in B nor every member of B is in A
c)Â Every member of B is in A.
d)Â At least one member of A is not in B
32) Answer (A)
Solution:
If we carefully observe set A, then we find that $6^{2n} -35n – 1$ is divisible by 35. So, set A contains multiples of 35. However, not all the multiples of 35 are there in set A, for different values of $n$.
For $n = 1$, the value is 0, for $n = 2$, the value is 1225 which is the 35th multiple of 3.
If we observe set B, it consists of all the multiples of 35 including 0.
So, we can say that every member of set A will be in B while every member of set B will not necessarily be in set A.
Hence, option A is the correct answer.
Question 33:Â $\frac{1}{log_{2}100}-\frac{1}{log_{4}100}+\frac{1}{log_{5}100}-\frac{1}{log_{10}100}+\frac{1}{log_{20}100}-\frac{1}{log_{25}100}+\frac{1}{log_{50}100}$=?
a)Â $\frac{1}{2}$
b)Â 10
c)Â 0
d) −4
33) Answer (A)
Solution:
We know that $\dfrac{1}{log_{a}{b}}$ = $\dfrac{log_{x}{a}}{log_{x}{b}}$
Therefore, we can say that $\dfrac{1}{log_{2}{100}}$ = $\dfrac{log_{10}{2}}{log_{10}{100}}$
$\Rightarrow$ $\frac{1}{log_{2}100}-\frac{1}{log_{4}100}+\frac{1}{log_{5}100}-\frac{1}{log_{10}100}+\frac{1}{log_{20}100}-\frac{1}{log_{25}100}+\frac{1}{log_{50}100}$
$\Rightarrow$ $\dfrac{log_{10}{2}}{log_{10}{100}}$-$\dfrac{log_{10}{4}}{log_{10}{100}}$+$\dfrac{log_{10}{5}}{log_{10}{100}}$-$\dfrac{log_{10}{10}}{log_{10}{100}}$+$\dfrac{log_{10}{20}}{log_{10}{100}}$-$\dfrac{log_{10}{25}}{log_{10}{100}}$+$\dfrac{log_{10}{50}}{log_{10}{100}}$
We know that $log_{10}{100}=2$
$\Rightarrow$ $\dfrac{1}{2}*[log_{10}{2}-log_{10}{4}+log_{10}{5}-log_{10}{10}+log_{10}{20}-log_{10}{25}+log_{10}{50}]$
$\Rightarrow$ $\dfrac{1}{2}*[log_{10}{\dfrac{2*5*20*50}{4*10*25}}]$
$\Rightarrow$ $\dfrac{1}{2}*[log_{10}10]$
$\Rightarrow$ $\dfrac{1}{2}$
Question 34:Â If p$^{3}$ = q$^{4}$ = r$^{5}$ = s$^{6}$, then the value of $log_{s}{(pqr)}$ is equal to
a)Â $\frac{47}{10}$
b)Â $\frac{24}{5}$
c)Â $\frac{16}{5}$
d)Â $1$
34) Answer (A)
Solution:
Given that, p$^{3}$ = q$^{4}$ = r$^{5}$ = s$^{6}$
p$^{3}$=s$^{6}$
p =Â s$^{\frac{6}{3}}$ = s$^{2}$Â Â …(1)
Similarly, q = s$^{\frac{6}{4}}$ = s$^{\frac{3}{2}}$  …(2)
Similarly, r =Â s$^{\frac{6}{5}}$Â Â …(3)
$\Rightarrow$ $log_{s}{(pqr)}$
By substituting value of p, q, and r from equation (1), (2) and (3)
$\Rightarrow$ $log_{s}{(s^{2}*s^{\frac{3}{2}}*s^{\frac{6}{5}})}$
$\Rightarrow$ $log_{s}(s^{\frac{47}{10}})$
$\Rightarrow$ $\dfrac{47}{10}$
Hence, option A is the correct answer.
Question 35: Let $t_{1},t_{2}$,… be real numbers such that $t_{1}+t_{2}+…+t_{n} = 2n^{2}+9n+13$, for every positive integer $n \geq 2$. If $t_{k}=103$, then k equals
35)Â Answer:Â 24
Solution:
It is given that $t_{1}+t_{2}+…+t_{n} = 2n^{2}+9n+13$, for every positive integer $n \geq 2$.
We can say that $t_{1}+t_{2}+…+t_{k} = 2k^{2}+9k+13$  … (1)
Replacing k by (k-1) we can say that
$t_{1}+t_{2}+…+t_{k-1} = 2(k-1)^{2}+9(k-1)+13$  … (2)
On subtracting equation (2) from equation (1)
$\Rightarrow$ $t_{k} =Â 2k^{2}+9k+13 –Â 2(k-1)^{2}+9(k-1)+13$
$\Rightarrow$ $103 =Â 4k+7$
$\Rightarrow$ $k =Â 24$
Question 36:Â Let $a_1, a_2, …$ be integers such that
$a_1 – a_2 + a_3 – a_4 + …. + (-1)^{n – 1} a_n = n,$ for all $n \geq 1.$
Then $a_{51} + a_{52} + …. + a_{1023}$ equals
a)Â 0
b)Â 1
c)Â 10
d)Â -1
36) Answer (B)
Solution:
$a_1 – a_2 + a_3 – a_4 + …. + (-1)^{n – 1} a_n = n$
It is clear from the above equation that when n is odd, the co-efficient of a is positive otherwise negative.
$a_1 – a_2 = 2$
$a_1 = a_2 + 2$
$a_1 – a_2 + a_3 = 3$
On substituting the value of $a_1$ in the above equation, we get
$a_3$ = 1
$a_1 – a_2 + a_3 – a_4 = 4$
On substituting the values of $a_1, a_3$ in the above equation, we get
$a_4$ = -1
$a_1 – a_2 + a_3 – a_4 +a_5 = 5$
On substituting the values of $a_1, a_3, a_4$ in the above equation, we get
$a_5$ = 1
So we can conclude that $a_3, a_5, a_7….a_{n+1}$ = 1 and $a_2, a_4, a_6….a_{2n}$ = -1
Now we have to find the value of $a_{51} + a_{52} + …. + a_{1023}$
Number of terms = 1023=51+(n-1)1
n=973
There will be 486 even and 487 odd terms, so the value of $a_{51} + a_{52} + …. + a_{1023}$ = 486*-1+487*1=1
Question 37:Â The real root of the equation $2^{6x} + 2^{3x + 2} – 21 = 0$ is
a)Â $\log_{2}{9}$
b)Â $\frac{\log_{2}{3}}{3}$
c)Â $\log_{2}{27}$
d)Â $\frac{\log_{2}{7}}{3}$
37) Answer (B)
Solution:
Let $2^{3x}$ = v
$2^{6x} + 2^{3x + 2} – 21 = 0$
= $v^2+4v-21=0$
=(v+7)(v-3)=0
v=3, -7
$2^{3x}$ = 3 or $2^{3x}$ = -7(This can be negated)
3x=$\log_23$
x=$\log_23$/3
Question 38:Â If $(2n + 1) + (2n + 3) + (2n + 5) + … + (2n + 47) = 5280$, then whatis the value of $1 + 2 + 3 + .. + n?$
38)Â Answer:Â 4851
Solution:
Let us first find the number of terms
47=1+(n-1)2
n=24
24*2n+1+3+5+….47=5280
48n+576=5280
48n=4704
n=98
Sum of first 98 terms = 98*99/2
=4851
Question 39:Â The number of common terms in the two sequences: 15, 19, 23, 27, . . . . , 415 and 14, 19, 24, 29, . . . , 464 is
a)Â 21
b)Â 20
c)Â 18
d)Â 19
39) Answer (B)
Solution:
A:Â 15, 19, 23, 27, . . . . , 415
B:Â 14, 19, 24, 29, . . . , 464
Here the first common term = 19
Common difference = LCM of 5, 4=20
19+(n-1)20Â $\le\ $ 415
(n-1)20Â $\le\ $ 396
(n-1) $\le\ $ 19.8
n=20
Question 40:Â If $a_1, a_2, ……$ are in A.P., then, $\frac{1}{\sqrt{a_1} + \sqrt{a_2}} + \frac{1}{\sqrt{a_2} + \sqrt{a_3}} + ……. + \frac{1}{\sqrt{a_n} + \sqrt{a_{n + 1}}}$ is equal to
a)Â $\frac{n}{\sqrt{a_1} + \sqrt{a_{n + 1}}}$
b)Â $\frac{n – 1}{\sqrt{a_1} + \sqrt{a_{n – 1}}}$
c)Â $\frac{n – 1}{\sqrt{a_1} + \sqrt{a_n}}$
d)Â $\frac{n}{\sqrt{a_1} – \sqrt{a_{n + 1}}}$
40) Answer (A)
Solution:
We have, $\frac{1}{\sqrt{a_1} + \sqrt{a_2}} + \frac{1}{\sqrt{a_2} + \sqrt{a_3}} + ……. + \frac{1}{\sqrt{a_n} + \sqrt{a_{n + 1}}}$
Now, $\frac{1}{\sqrt{a_1} + \sqrt{a_2}}$ = $\frac{\sqrt{a_2} – \sqrt{a_1}}{(\sqrt{a_2} + \sqrt{a_1})(\sqrt{a_2} – \sqrt{a_1})}$  (Multiplying numerator and denominator by $\sqrt{a_2} – \sqrt{a_1}$)
= $\frac{\sqrt{a_2} – \sqrt{a_1}}{({a_2} – {a_1}}$
=$\frac{\sqrt{a_2} – \sqrt{a_1}}{d}$Â Â (where d is the common difference)
Similarly, $ \frac{1}{\sqrt{a_2} + \sqrt{a_3}}$ = $\frac{\sqrt{a_3} – \sqrt{a_2}}{d}$ and so on.
Then the expression $\frac{1}{\sqrt{a_1} + \sqrt{a_2}} + \frac{1}{\sqrt{a_2} + \sqrt{a_3}} + ……. + \frac{1}{\sqrt{a_n} + \sqrt{a_{n + 1}}}$
can be written as $\ \frac{\ 1}{d}(\sqrt{a_2}-\sqrt{a_1}+\sqrt{a_3}-\sqrt{a_3}+……………………..\sqrt{a_{n+1}} – \sqrt{a_{n}}$
=Â $\ \frac{\ n}{nd}(\sqrt{a_{n+1}}-\sqrt{a_1})$Â (Multiplying both numerator and denominator by n)
= $\ \frac{n(\sqrt{a_{n+1}}-\sqrt{a_1})}{{a_{n+1}} – {a_1}}$Â Â Â $(a_{n+1} – {a_1} =nd)$
=Â $\frac{n}{\sqrt{a_1} + \sqrt{a_{n + 1}}}$
Question 41:Â If $(5.55)^x = (0.555)^y = 1000$, then the value of $\frac{1}{x} – \frac{1}{y}$ is
a)Â $\frac{1}{3}$
b)Â 3
c)Â 1
d)Â $\frac{2}{3}$
41) Answer (A)
Solution:
We have, $(5.55)^x = (0.555)^y = 1000$
Taking log in base 10 on both sides,
x($\log_{10}555$-2) = y($\log_{10}555$-3) = 3
Then, x($\log_{10}555$-2) = 3…..(1)
y($\log_{10}555$-3) = 3Â …..(2)
From (1) and (2)
=> $\log_{10}555$=$\ \frac{\ 3}{x}$+2=$\ \frac{\ 3}{y}+3$
=>Â $\frac{1}{x} – \frac{1}{y}$ =Â $\frac{1}{3}$
Question 42:Â If the population of a town is p in the beginning of any year then it becomes 3 + 2p in the beginning of the next year. If the population in the beginning of 2019 is 1000, then the population in the beginning of 2034 will be
a)Â $(1003)^{15} + 6$
b)Â $(997)^{15} – 3$
c)Â $(997)2^{14} + 3$
d)Â $(1003)2^{15} – 3$
42) Answer (D)
Solution:
The population of town at the beginning of 1st year = p
The population of town at the beginning of 2nd year = 3+2p
The population of town at the beginning of 3rd year = 2(3+2p)+3 = 2*2p+2*3+3 =4p+3(1+2)
The population of town at the beginning of 4th year = 2(2*2p+2*3+3)+3 = 8p+3(1+2+4)
Similarly population at the beginning of the nth year =Â $2^{n-1}$p+3($2^{n-1}-1$) =Â $2^{n-1}\left(p+3\right)$-3
The population in the beginning of 2019 is 1000, then the population in the beginning of 2034 will be $(2^{2034-2019})\left(1000+3\right)$-3 = $2^{15}\left(1003\right)$-3
Question 43:Â A club has 256 members of whom 144 can play football, 123 can play tennis, and 132 can play cricket. Moreover, 58 members can play both football and tennis, 25 can play both cricket and tennis, while 63 can play both football and cricket. If every member can play at least one game, then the number of members who can play only tennis is
a)Â 38
b)Â 32
c)Â 45
d)Â 43
43) Answer (D)
Solution:
Assume the number of members who can play exactly 1 game = I
The number of members who can play exactly 1 game = II
The number of members who can play exactly 1 game = III
I+2II+3III=144+123+132=399….(1)
I+II+III=256……(2)
=> II+2III=143…..(3)
Also, II+3III=58+25+63=146 ……(4)
=> III = 3 (From 3 and 4)
=> II =137
=> I = 116
The members who play only tennis = 123-58-25+3 = 43
Question 44:Â If $a_1 + a_2 + a_3 + …. + a_n = 3(2^{n + 1} – 2)$, for every $n \geq 1$, then $a_{11}$ equals
44)Â Answer:Â 6144
Solution:
11th term of series =Â $a_{11}$ = Sum of 11 terms – Sum of 10 terms = $3(2^{11 + 1} – 2)$-3$(2^{10 + 1} – 2)$
= 3$(2^{12} – 2-2^{11} +2)$=3$(2^{11})(2-1)$= 3*$2^{11}$ = 6144
Question 45:Â If m and n are integers such that $(\surd2)^{19} 3^4 4^2 9^m 8^n = 3^n 16^m (\sqrt[4]{64})$ then m is
a)Â -20
b)Â -24
c)Â -12
d)Â -16
45) Answer (C)
Solution:
We have, $(\surd2)^{19} 3^4 4^2 9^m 8^n = 3^n 16^m (\sqrt[4]{64})$
Converting both sides in powers of 2 and 3, we get
$2^{\ \frac{19\ }{2}}3^42^43^{2m}2^{3n}$ =Â $3^n2^{4m}2^{\frac{\ 6}{4}}$
Comparing the power of 2 we get, $\ \frac{\ 19}{2}+4+3n\ =4m+\frac{\ 6}{4}\ $
=> 4m=3n+12 …..(1)
Comparing the power of 3 we get, $4+2m=n$
Substituting the value of n in (1), we get
4m=3(4+2m)+12
=> m=-12
Question 46:Â Let x and y be positive real numbers such that
$\log_{5}{(x + y)} + \log_{5}{(x – y)} = 3,$ and $\log_{2}{y} – \log_{2}{x} = 1 – \log_{2}{3}$. Then $xy$ equals
a)Â 150
b)Â 25
c)Â 100
d)Â 250
46) Answer (A)
Solution:
We have, $\log_{5}{(x + y)} + \log_{5}{(x – y)} = 3$
=>Â $x^2-y^2=125$……(1)
$\log_{2}{y} – \log_{2}{x} = 1 – \log_{2}{3}$
=>$\ \frac{\ y}{x}$ =Â $\ \frac{\ 2}{3}$
=> 2x=3y  => x=$\ \frac{\ 3y}{2}$
On substituting the value of x in 1, we get
$\ \frac{\ 5x^2}{4}$=125
=>y=10, x=15
Hence xy=150
Question 47: With rectangular axes of coordinates, the number of paths from (1, 1) to (8, 10) via (4, 6), where each step from any point (x, y) is either to (x, y+1) or to (x+1, y), is
47)Â Answer:Â 3920
Solution:
The number of paths from (1, 1) to (8, 10) via (4, 6) = The number of paths from (1,1) to (4,6) * The number of paths from (4,6) to (8,10)
To calculate the number of paths from (1,1) to (4,6), 4-1 =3 steps in x-directions and 6-1=5 steps in y direction
Hence the number of paths from (1,1) to (4,6) = $^{(3+5)}C_3$ = 56
To calculate the number of paths from (4,6) to (8,10), 8-4 =4 steps in x-directions and 10-6=4 steps in y direction
Hence the number of paths from (4,6) to (8,10) = $^{(4+4)}C_4$ = 70
The number of paths from (1, 1) to (8, 10) via (4, 6) = 56*70=3920
Question 48:Â If Y is a negative number such that $2^{Y^2({\log_{3}{5})}}=5^{\log_{2}{3}}$, then Y equals to:
a)Â $\log_{2}(\frac{1}{5})$
b)Â $\log_{2}(\frac{1}{3})$
c)Â $-\log_{2}(\frac{1}{5})$
d)Â $-\log_{2}(\frac{1}{3})$
48) Answer (B)
Solution:
$2^{Y^2({\log_{3}{5})}}=5^{Y^2(\log_3 2)}$
Given, $5^{Y^2\left(\log_32\right)}=5^{\left(\log_23\right)}$
=>Â $Y^2\left(\log_32\right)=\left(\log_23\right)=>Y^2=\left(\log_23\right)^2$
=>$Y=\left(-\log_23\right)^{\ }or\ \left(\log_23\right)$
since Y is a negative number, Y=$\left(-\log_23\right)=\left(\log_2\frac{1}{3}\right)$
Question 49:Â If $x=(4096)^{7+4\sqrt{3}}$, then which of the following equals to 64?
a)Â $\frac{x^{7}}{x^{2\sqrt{3}}}$
b)Â $\frac{x^{\frac{7}{2}}}{x^{\frac{4}{\sqrt{3}}}}$
c)Â $\frac{x^{\frac{7}{2}}}{x^{2{\sqrt{3}}}}$
d)Â $\frac{x^{7}}{x^{4\sqrt{3}}}$
49) Answer (C)
Solution:
$x=2^{12\left(7+4\sqrt{\ 3}\right)}$.
$x^{\frac{7}{2}}=2^{42\left(7+4\sqrt{\ 3}\right)}$
$x^{2\sqrt{\ 3}}=2^{24\sqrt{\ 3}\left(7+4\sqrt{\ 3}\right)}$
$\frac{x^{\frac{7}{2}}}{x^{2{\sqrt{3}}}}$ =Â $2^{\left(7+4\sqrt{\ 3}\right)\left(42-24\sqrt{\ 3}\right)}=2^{\left(7+4\sqrt{\ 3}\right)\left(7-4\sqrt{\ 3}\right)6}$ =$2^6$.
Hence C is correct answer.
Question 50:Â If $\log_{4}{5}=(\log_{4}{y})(\log_{6}{\sqrt{5}})$, then y equals
50)Â Answer:Â 36
Solution:
$\frac{\log\ 5}{2\log2}\ =\frac{\log\ y}{2\log2}\cdot\frac{\log\ 5}{2\log6}$
$\log\ 36\ =\ \log\ y;\ \therefore\ y\ =36$
Question 51:Â If $x_1=-1$ and $x_m=x_{m+1}+(m+1)$ for every positive integer m, then $X_{100}$ equals
a)Â -5050
b)Â -5151
c)Â -5051
d)Â -5150
51) Answer (A)
Solution:
$x_1=-1$
$x_1=x_2+2$ =>Â $x_2=x_1-2$ = -3
Similarly,
$x_3=x_1-5=-6$
$x_4=-10$
.
.
The series is -1, -3, -6, -10, -15……
When the differences are in AP, then the nth term is $-\frac{n\left(n+1\right)}{2}$
$x_{100}=-\frac{100\left(100+1\right)}{2}=-5050$
Question 52:Â If $\log_{a}{30}=A,\log_{a}({\frac{5}{3}})=-B$ and $\log_2{a}=\frac{1}{3}$, then $\log_3{a}$ equals
a)Â $\frac{2}{A+B-3}$
b)Â $\frac{2}{A+B}-3$
c)Â $\frac{A+B}{2}-3$
d)Â $\frac{A+B-3}{2}$
52) Answer (A)
Solution:
$\log_a30=A\ or\ \log_a5+\log_a2+\log_a3=A$………..(1)
$\log_a\left(\frac{5}{3}\right)=-B\ or\ \log_a3-\log_a5=B$………….(2)
and finally $\log_a2=3$
Substituting this in (1) we get $\log_a5+\log_a3=A-3$
Now we have two equations in two variables (1) and (2) . On solving we get
$\log_a3=\frac{\left(A+B-3\right)}{2\ }or\ \log_3a=\frac{2}{A+B-3}$
Question 53:Â If a,b,c are non-zero and $14^a=36^b=84^c$, then $6b(\frac{1}{c}-\frac{1}{a})$ is equal to
53)Â Answer:Â 3
Solution:
Let $14^a=36^b=84^c$ = k
=> a =Â $\log_{14}k$ , b =Â $\log_{36}k$, c=$\log_{84}k$
$6b(\frac{1}{c}-\frac{1}{a})$ =Â $6\cdot\frac{1}{2}\log_6k\left(\log_k84-\log_k14\right)$ = 3
Question 54:Â $\frac{2\times4\times8\times16}{(\log_{2}{4})^{2}(\log_{4}{8})^{3}(\log_{8}{16})^{4}}$ equals
54)Â Answer:Â 24
Solution:
$\frac{\left(2\cdot4\cdot8\cdot16\right)}{\left(\log_22^2\right)^2\cdot\left(\log_{2^2}2^3\right)^3\cdot\left(\log_{2^3}2^4\right)^4}\cdot$
=Â $\frac{2^{10}}{4\cdot\left(\frac{3}{2}\right)^3\cdot\left(\frac{4}{3}\right)^4}=24$
Question 55:Â The value of $\log_{a}({\frac{a}{b}})+\log_{b}({\frac{b}{a}})$, for $1<a\leq b$ cannot be equal to
a)Â 0
b)Â -1
c)Â 1
d)Â -0.5
55) Answer (C)
Solution:
On expanding the expression we get $1-\log_ab+1-\log_ba$
$or\ 2-\left(\log_ab+\frac{1}{\log_ba}\right)$
Now applying the property of AM>=GM, we get that  $\frac{\left(\log_ab+\frac{1}{\log_ba}\right)}{2}\ge1\ or\ \left(\log_ab+\frac{1}{\log_ba}\right)\ge2$ Hence from here we can conclude that the expression will always be equal to 0 or less than 0. Hence any positive value is not possible. So 1 is not possible.
Question 56: Students in a college have to choose at least two subjects from chemistry, mathematics and physics. The number of students choosing all three subjects is 18, choosing mathematics as one of their subjects is 23 and choosing physics as one of their subjects is 25. The smallest possible number of students who could choose chemistry as one of their subjects is
a)Â 22
b)Â 21
c)Â 20
d)Â 19
56) Answer (C)
Solution:
Now 23 students choose maths as one of their subject.
This means (MPC)+ (MC) + (PC)=23 where MPC denotes students who choose all the three subjects maths, physics and chemistry and so on.
So MC + PM =5 Similarly we have PC+ MP =7
We have to find the smallest number of students choosing chemistry
For that in the first equation let PM=5 and MC=0. In the second equation this PC=2
Hence minimum number of students choosing chemistry will be (18+2)=20 Since 18 students chose all the three subjects.
Question 57:Â Let the m-th and n-th terms of a geometric progression be $\frac{3}{4}$ and 12. respectively, where $m < n$. If the common ratio of the progression is an integer r, then the smallest possible value of $r + n – m$ is
a)Â 6
b)Â 2
c)Â -4
d)Â -2
57) Answer (D)
Solution:
Let the first term of the GP be “a” . Now from the question we can show that
$ar^{m-1}=\frac{3}{4}$Â Â Â $ar^{n-1}=12$
Dividing both the equations we get $r^{m-1-n+1}=\frac{1}{16}\ or\ r^{m-n}=16^{-1\ }or\ r^{n-m}=16$
So for the minimum possible value we take Now give minimum possible value to “r” i.e -4 and n-m=2
Hence minimum possible value of r+n-m=-4+2=-2
Question 58:Â The number of groups of three or more distinct numbers that can be chosen from 1, 2, 3, 4, 5, 6, 7 and 8 so that the groups always include 3 and 5, while 7 and 8 are never included together is
58)Â Answer:Â 47
Solution:
The possible arrangements are of the form
35 _ Can be chosen in 6 ways.
35 _ _ We can choose 2 out of the remaining 6 in $^6C_2=15$ways. We remove 1 case where 7 and 8 are together to get 14 ways.
35 _ _ _We can choose 3 out of the remaining 6 in $^6C_3=20$ways. We remove 4 cases where 7 and 8 are together to get 16 ways.
35 _ _ _ _We can choose 4 out of the remaining 6 in $^6C_4=15$ways. We remove 6 case where 7 and 8 are together to get 9 ways.
35 _ _ _ _ _ We choose 1 out of 7 and 8 and all the remaining others in 2 ways.
Thus, total number of cases = 6+14+16+9+2 = 47.
Alternatively,
The arrangement requires a selection of 3 or more numbers while including 3 and 5 and 7, 8 are never included together. We have cases including a selection of only 7, only 8 and neither 7 nor 8.
Considering the cases, only 7 is selected.
We can select a maximum of 7 digit numbers. We must select 3, 5, and 7.
Hence we must have ( 3, 5, 7) for the remaining 4 numbers we have
Each of the numbers can either be selected or not selected and we have 4 numbers :
Hence we have _ _ _ _ and 2 possibilities for each and hence a total of 2*2*2*2 = 16 possibilities.
SImilarly, including only 8, we have 16 more possibilities.
Cases including neither 7 nor 8.
We must have 3 and 5 in the group but there must be no 7 and 8 in the group.
Hence we have 3 5 _ _ _ _.
For the 4 blanks, we can have 2 possibilities for either placing a number or not among 1, 2, 4, 6.
= 16 possibilities
But we must remove the case where neither of the 4 numbers are placed because the number becomes a two-digit number.
Hence 16 – 1 = 15 cases.
Total = 16+15+16 = 47 possibilities
Question 59:Â How many three-digit numbers are greater than 100 and increase by 198 when the three digits are arranged in the reverse order?
59)Â Answer:Â 70
Solution:
Let the numbers be of the form 100a+10b+c, where a, b, and c represent single digits.
Then (100c+10b+a)-(100a+10b+c)=198
99c-99a=198
c-a = 2.
Now, a can take the values 1-7. a cannot be zero as the initial number has 3 digits and cannot be 8 or 9 as then c would not be a single-digit number.
Thus, there can be 7 cases.
B can take the value of any digit from 0-9, as it does not affect the answer. Hence, the total cases will be $7\times\ 10=70$.
Question 60:Â If $5 – \log_{10}\sqrt{1 + x} + 4 \log_{10} \sqrt{1 – x} = \log_{10} \frac{1}{\sqrt{1 – x^2}}$, then 100x equals
60)Â Answer:Â 99
Solution:
$5 – \log_{10}\sqrt{1 + x} + 4 \log_{10} \sqrt{1 – x} = \log_{10} \frac{1}{\sqrt{1 – x^2}}$
We can re-write the equation as:Â $5-\log_{10}\sqrt{1+x}+4\log_{10}\sqrt{1-x}=\log_{10}\left(\sqrt{1+x}\times\ \sqrt{1-x}\right)^{-1}$
$5-\log_{10}\sqrt{1+x}+4\log_{10}\sqrt{1-x}=\left(-1\right)\log_{10}\left(\sqrt{1+x}\right)+\left(-1\right)\log_{10}\left(\sqrt{1-x}\right)$
$5=-\log_{10}\sqrt{1+x}+\log_{10}\sqrt{1+x}-\log_{10}\sqrt{1-x}-4\log_{10}\sqrt{1-x}$
$5=-5\log_{10}\sqrt{1-x}$
$\sqrt{1-x}=\frac{1}{10}$
Squaring both sides:Â $\left(\sqrt{1-x}\right)^2=\frac{1}{100}$
$\therefore\ $Â $x=1-\frac{1}{100}=\frac{99}{100}$
Hence, $100\ x\ =100\times\ \frac{99}{100}=99$
Question 61:Â If $x_0 = 1, x_1 = 2$, and $x_{n + 2} = \frac{1 + x_{n + 1}}{x_n}, n = 0, 1, 2, 3, ……,$ then $x_{2021}$ is equal to
a)Â 4
b)Â 1
c)Â 3
d)Â 2
61) Answer (D)
Solution:
$x_0=1$
$x_1=2$
$x_2=\frac{\left(1+x_1\right)}{x_0}=\frac{\left(1+2\right)}{1}=3$
$x_3=\frac{\left(1+x_2\right)}{x_1}=\frac{\left(1+3\right)}{2}=2$
$x_4=\frac{\left(1+x_3\right)}{x_2}=\frac{\left(1+2\right)}{3}=1$
$x_5=\frac{\left(1+x_4\right)}{x_3}=\frac{\left(1+1\right)}{2}=1$
$x_6=\frac{\left(1+x_5\right)}{x_4}=\frac{\left(1+1\right)}{1}=2$
Hence, the series begins to repeat itself after every 5 terms. Terms whose number is of the form 5n are 1, 5n+1 are 2… and so on, where n=0,1,2,3,….
2021 is of the form 5n+1. Hence, its value will be 2.
Question 62: The natural numbers are divided into groups as (1), (2, 3, 4), (5, 6, 7, 8, 9), ….. and so on. Then, the sum of the numbers in the 15th group is equal to
a)Â 6119
b)Â 6090
c)Â 4941
d)Â 7471
62) Answer (A)
Solution:
The first number in each group: 1, 2, 5, 10, 17…..
Their common difference is in Arithmetic Progression. Hence, the general term of the series can be expressed as a quadratic equation.
Let the quadratic equation of the general term be $ax^2+bx+c$
1st term = a+b+c=1
2nd term = 4a+2b+c=2
3rd term = 9a+3b+c=5
Solving the equations, we get a=1, b=-2, c=2.
Hence, the first term in the 15th group will be $\left(15\right)^2-2\left(15\right)+2=197$
We can see that the number of terms in each group is 1, 3, 5, 7…. and so on. These are of the form 2n-1. Hence, the number of terms in 15th group will be 29. Hence, the last term in the 15th group will be 197+29-1 = 225.
Sum of terms in group 15=Â $\frac{29}{2}\left(197+225\right)\ =\ 6119$
Alternatively,
The final term in each group is the square of the group number.
In the first group 1, second group 4, …………
The final element of the 14th group is $\left(14\right)^2=\ 196$, similarly for the 15th group this is : $\left(15\right)^2=\ 225$
Each group contains all the consecutive elements in this range.
Hence the 15th group the elements are:
(197, 198, …………………………..225).
This is an Arithmetic Progression with a common difference of 1 and the number of element 29.
Hence the sum is given by :Â Â $\frac{n}{2}\cdot\left(first\ term\ +last\ term\right)$
$\frac{29}{2}\cdot\left(197+225\right)$
6119.
Question 63:Â Three positive integers x, y and z are in arithmetic progression. If $y-x>2$ and $xyz=5(x+y+z)$, then z-x equals
a)Â 8
b)Â 12
c)Â 14
d)Â 10
63) Answer (C)
Solution:
Given x, y, z are three terms in an arithmetic progression.
Considering x = a, y = a+d, z = a+2*d.
Using the given equation x*y*z = 5*(x+y+z)
a*(a+d)*(a+2*d) = 5*(a+a+d+a+2*d)
=a*(a+d)*(a+2*d)Â = 5*(3*a+3*d) = 15*(a+d).
= a*(a+2*d) = 15.
Since all x, y, z are positive integers and y-x > 2. a, a+d, a+2*d are integers.
The common difference is positive and greater than 2.
Among the different possibilities are : (a=1, a+2d = 5), (a, =3, a+2d = 5), (a = 5, a+2d = 3), (a=15, a+2d = 1)
Hence the only possible case satisfying the condition is :
a = 1, a+2*d = 15.
x = 1, z = 15.
z-x = 14.
Question 64:Â The number of ways of distributing 15 identical balloons, 6 identical pencils and 3 identical erasers among 3 children, such that each child gets at least four balloons and one pencil, is
64)Â Answer:Â 1000
Solution:
This question is an application of the product rule in probability and combinatorics.
In the product rule, if two events A and B can occur in x and y ways, and for an event E, both events A and B need to take place, the number of ways that E can occur is xy. This can be expanded to 3 or more events as well.
Event 1: Distribution of balloons
Since each child gets at least 4 balloons, we will initially allocate these 4 balloons to each of them.
So we are left with 15 – 4 x 3 = 15 – 12 = 3 balloons and 3 children.
Now we need to distribute 3 identical balloons to 3 children.
This can be done in $^{n+r-1}C_{r-1}$ ways, where n = 3 and r = 3.
So, number of ways =Â $^{3+3-1}C_{3-1}=^5C_2=\frac{5\times\ 4}{2\times\ 1}=10$
Event 2: Distribution of pencils
Since each child gets at least one pencil, we will allocate 1 pencil to each child. We are now left with 6 – 3 = 3 pencils.
We now need to distribute 3 identical pencils to 3 children.
This can be done in $^{n+r-1}C_{r-1}$ ways, where n = 3 and r = 3.
So, number of ways = $^{3+3-1}C_{3-1}=^5C_2=\frac{5\times\ 4}{2\times\ 1}=10$
Event 3: Distribution of erasers
We need to distribute 3 identical erasers to 3 children.
This can be done in $^{n+r-1}C_{r-1}$ ways, where n = 3 and r = 3.
So, number of ways = $^{3+3-1}C_{3-1}=^5C_2=\frac{5\times\ 4}{2\times\ 1}=10$
Applying the product rule, we get the total number of ways = 10 x 10 x 10 = 1000.
Question 65:Â If $\log_{2}[3+\log_{3} \left\{4+\log_{4}(x-1) \right\}]-2=0$ then 4x equals
65)Â Answer:Â 5
Solution:
We have :
$\log_2\left\{3+\log_3\left\{4+\log_4\left(x-1\right)\right\}\right\}=2$
we get $3+\log_3\left\{4+\log_4\left(x-1\right)\right\}=4$
we get $\log_3\left(4+\log_4\left(x-1\right)\ =\ 1\right)$
we get $4+\log_4\left(x-1\right)\ =\ 3$
$\log_4\left(x-1\right)\ =\ -1$
x-1 = 4^-1
x =Â $\frac{1}{4}+1=\frac{5}{4}$
4x = 5
Question 66:Â For a sequence of real numbers $x_{1},x_{2},…x_{n}$, If $x_{1}-x_{2}+x_{3}-….+(-1)^{n+1}x_{n}=n^{2}+2n$ for all natural numbers n, then the sum $x_{49}+x_{50}$ equals
a)Â 200
b)Â 2
c)Â -200
d)Â -2
66) Answer (D)
Solution:
Now as per the given series :
we get $x_1=1+2\ =3$
Now $x_1-x_2=\ 8$
so$x_2=-5$
Now $x_1-x_2+x_3\ =\ 15$
so $x_3\ =7$
so we get $x_n\ =\left(-1\right)^{n+1}\left(2n+1\right)$
so $x_{49}\ =\ 99$ and $x_{50}\ =-101$
Therefore $x_{49\ }+x_{50}\ =-2$
Question 67:Â For a real number a, if $\frac{\log_{15}{a}+\log_{32}{a}}{(\log_{15}{a})(\log_{32}{a})}=4$ then a must lie in the range
a)Â $2<a<3$
b)Â $3<a<4$
c)Â $4<a<5$
d)Â $a>5$
67) Answer (C)
Solution:
We have :$\frac{\log_{15}{a}+\log_{32}{a}}{(\log_{15}{a})(\log_{32}{a})}=4$
We get $\frac{\left(\frac{\log a}{\log\ 15}+\frac{\log a}{\log32}\right)}{\frac{\log a}{\log\ 15}\times\ \frac{\log a}{\log32}\ \ }=4$
we get $\log a\left(\log32\ +\log\ 15\right)=4\left(\log\ a\right)^2$
we get $\left(\log32\ +\log\ 15\right)=4\log a$
=$\log480=\log a^4$
=$a^4\ =480$
so we can say a is between 4 and 5 .
Question 68:Â Consider a sequence of real numbers, $x_{1},x_{2},x_{3},…$ such that $x_{n+1}=x_{n}+n-1$ for all $n\geq1$. If $x_{1}=-1$ then $x_{100}$ is equal to
a)Â 4849
b)Â 4949
c)Â 4950
d)Â 4850
68) Answer (D)
Solution:
Given $x_{n+1}\ =\ x_n\ +\ n\ -1$ and x1 = -1.
Considering
x1  = -1.   (1)
x2Â Â = x1+1-1 = x1 + 0Â Â (2)
x3Â Â = x2 + 2 – 1Â =x2 + 1Â Â Â (3)
x4Â Â = x3 + 3 – 1 = x3 + 2Â Â Â Â (4)
x100 = x99 + 98Â Â Â (100)
Adding the LHS and RHS for the hundred equations we have:
(x1+x2+………………….x100) = (-1+0+………98) + (x1+x2+……………x99)
Subtracting this we have :
(x1+………..x100) – (x1+…………. x 99) = $\frac{\left(98\cdot99\right)}{2}$ – 1.
x100 = 4851 – 1 = 4850
Alternatively
$x_1=-1$
$x_2=x_1+1-1=x_1=-1$
$x_3=x_2+2-1=x_2+1=-1+1=0$
$x_4=x_3+3-1=x_3+2=0+2=2$
$x_5=x_4+4-1=x_4+3=2+3=5$
……
If we observe the series, it is a series that has a difference between the consecutive terms in an AP.
Such series are represented as $t\left(n\right)=a+bn+cn^2$
We need to find t(100).
t(1) = -1
a + b + c = -1
t(2) = -1
a + 2b + 4c = -1
t(3) = 0
a + 3b + 9c = 0
Solving we get,
b + 3c = 0
b + 5c = 1
c = 0.5
b = -1.5
a = 0
Now,
$t\left(100\right)=\left(-1.5\right)100+\left(0.5\right)100^2=-150+5000=4850$
Question 69:Â A four-digit number is formed by using only the digits 1, 2 and 3 such that both 2 and 3 appear at least once. The number of all such four-digit numbers is
69)Â Answer:Â 50
Solution:
The question asks for the number of 4 digit numbers using only the digits 1, 2, and 3 such that the digits 2 and 3 appear at least once.
The different possibilities include :
Case 1:The four digits are ( 2, 2, 2, 3). Since the number 2 is repeated 3 times. The total number of arrangements are :
$\frac{4!}{3!}$ = 4.
Case 2: The four digits are 2, 2, 3, 3. The total number of four-digit numbers formed using this are :
$\frac{4!}{2!\cdot2!}=\ 6$
Case 3: The four digits are 2, 3, 3, 3. The number of possible 4 digit numbers are :
$\frac{4!}{3!}$ = 4
Case4: The four digits are 2, 3, 3, 1. The number of possible 4 digit numbers are :
$\frac{4!}{2!}=\ 12$
Case5: Using the digits 2, 2, 3, 1. The number of possible 4 digit numbers are :
$\frac{4!}{2!}=\ 12$
Case 6: Using the digits 2, 3, 1, 1. The number of possible 4 digit numbers are :
$\frac{4!}{2!}=\ 12$
A total of 12 + 12 + 12 + 4 + 6 + 4 = 50 possibilities.
Alternatively
We have to form 4 digit numbers using 1,2,3 such that 2,3 appears at least once
So the possible cases :
Now we get $\frac{4!}{2!}\times\ 3$= 36 ( When one digit is used twice and the remaining two once )
$\frac{4!}{3!}\times\ 2$ = 8 ( When 1 is used 0 times and 2 and 3 is used 3 times or 1 time )
$\frac{4!}{2!\times\ 2!}=\ 6$( When 2 and 3 is used 2 times each )
So total numbers = 36+8+6 =50
Question 70:Â For any natural number n, suppose the sum of the first n terms of an arithmetic progression is $(n + 2n^2)$. If the $n^{th}$ term of the progression is divisible by 9, then the smallest possible value of n is
a)Â 9
b)Â 4
c)Â 7
d)Â 8
70) Answer (C)
Solution:
It is given,
$S_n=2n^2+n$
$S_{n-1}=2\left(n-1\right)^2+\left(n-1\right)$
$S_{n-1}=2n^2-3n+1$
$T_n=S_n-S_{n-1}=2n^2+n-2n^2+3n-1=4n-1$
$T_n=4n-1$
The terms are 3, 7, 11, 15, 19, 23, 27,……
27 is the first term in the series divisible by 9.
27 is the 7th term.
Therefore, the least possible value of n is 7.
The answer is option C.
Question 71:Â The number of ways of distributing 20 identical balloons among 4 children such that each child gets some balloons but no child gets an odd number of balloons, is
71)Â Answer:Â 84
Solution:
Let the number of balloons each child received be 2a, 2b, 2c and 2d
2a + 2b + 2c + 2d = 20
a + b + c + d = 10
Each of them should get more than zero balloons.
Therefore, total number of ways =Â $(n-1)_{C_{r-1}}=(10-1)_{C_{4-1}}=9_{C_3}=84$
Question 72:Â In a class of 100 students, 73 like coffee, 80 like tea and 52 like lemonade. It may be possible that some students do not like any of these three drinks. Then the difference between the maximum and minimum possible number of students who like all the three drinks is
a)Â 47
b)Â 53
c)Â 52
d)Â 48
72) Answer (A)
Solution:
Let n, s, d and t be the number of students who likes none of the drinks, exactly one drink, exactly 2 drinks and all three drinks, respectively.
It is given,
n +Â s + d + t = 100 …… (1)
s + 2d + 3t = 73 + 80 + 52
s + 2d + 3t = 205 …… (2)
(2)-(1), we get
d + 2t – n = 105
Maximum value t can take is 52, i.e. t = 52, d = 1 and n = 0
Minimum value t can take is 5, i.e. t = 5, d = 95 and n = 0 (This also satisfies equation (1))
Difference = 52 – 5 = 47
The answer is option A.
Question 73:Â The average of a non-decreasing sequence of N numbers $a_{1},a_{2}, … , a_{N}$ is 300. If $a_1$, is replaced by $6a_{1}$ , the new average becomes 400. Then, the number of possible values of $a_{1 }$, is
73)Â Answer:Â 14
Solution:
$a_1+a_2+…..+a_N=300N$
$6a_1+a_2+…..+a_N=400N$
$5a_1=100N$
$a_1=20N$
As the given sequence of numbers is non-decreasing sequence, N can take values from 2 to 15.
N is not equal to 1, if N = 1, then average of N numbers is 300 wouldn’t satisfy.
Therefore, N can take values from 2 to 15, i.e. 14 values.
Question 74: On day one, there are 100 particles in a laboratory experiment. On day n, where $n\ge2$, one out of every n articles produces another particle. If the total number of particles in the laboratory experiment increases to 1000 on day m, then m equals
a)Â 19
b)Â 16
c)Â 18
d)Â 17
74) Answer (A)
Solution:
Given, the number of particles on day 1 = 100
On day 2, one out of every 2 articles produces another particle.
The number of particles on day 2 will be $\frac{100}{2}$, i.e. 50 particles
On day 3, one out of every 3 articles produces another particle.
The number of particles on day 3 will be $\frac{100+50}{3}$, i.e. 50 particles
On day 4, one out of every 4 articles produces another particle.
The number of particles on day 4 will be $\frac{100+50+50}{4}$, i.e. 50 particles
Series will be 100, 50, 50, 50,….
It is given,
100 + (m-1)50 = 1000
m = 19
The answer is option A.
Question 75:Â The number of integers greater than 2000 that can be formed with the digits 0, 1, 2, 3, 4, 5, using each digit at most once, is
a)Â 1440
b)Â 1200
c)Â 1480
d)Â 1420
75) Answer (A)
Solution:
Case 1: 4-digit numbers
Given digits – 0, 1, 2, 3, 4, 5
_, _, _, _
As the numbers should be greater than 2000, first digit can be 2, 3, 4 and 5.
For remaining digits, we need to arrange 3 digits from the remaining 5 digits, i.e. 5*4*3 = 60 ways
Total number of possible 4-digit numbers = 4*60 = 240
Case 2: 5-digit numbers
_, _, _, _, _
First digit cannot be zero.
Therefore, total number of cases = 5*5*4*3*2 = 600
Case 3: 6-digit numbers
_, _, _, _, _, _
First digit cannot be zero.
Therefore, total number of cases = 5*5*4*3*2*1 = 600
Total number of integers possible = 600 + 600 + 240 = 1440
The answer is option A.
Question 76: Consider the arithmetic progression 3, 7, 11, … and let $A_n$ denote the sum of the first n terms of this progression. Then the value of $\frac{1}{25} \sum_{n=1}^{25} A_{n}$ is
a)Â 455
b)Â 442
c)Â 415
d)Â 404
76) Answer (A)
Solution:
Sum of n terms in an A.P =Â $\frac{n}{2}\left(2a+\left(n-1\right)d\right)$
$A_n=\frac{n}{2}\left(6+\left(n-1\right)4\right)=n\left(2n+1\right)$
$\Sigma\ A_n=\Sigma\ n\left(2n+1\right)=2\Sigma\ n^2+\Sigma\ n=\ \frac{\ 2n\left(n+1\right)\left(2n+1\right)}{6}+\ \frac{\ n\left(n+1\right)}{2}$
Substituting n = 25, we get
$\frac{1}{25} \sum_{n=1}^{25} A_{n}$ =Â $\frac{1}{25}\left(\ \frac{\ 2\left(25\right)\left(25+1\right)\left(50+1\right)}{6}+\ \frac{\ 25\left(25+1\right)}{2}\right)$
$\frac{1}{25} \sum_{n=1}^{25} A_{n}$ =Â $\ 26\left(17\right)+13$ = 455
The answer is option A.
Question 77:Â If $(\sqrt{\frac{7}{5}})^{3x-y}=\frac{875}{2401}$ and $(\frac{4a}{b})^{6x-y}=(\frac{2a}{b})^{y-6x}$, for all non-zero real values of a and b, then the value of $x+y$ is
77)Â Answer:Â 14
Solution:
$(\sqrt{\frac{7}{5}})^{3x-y}=\frac{875}{2401}$
$\left(\frac{7}{5}\right)^{\frac{\left(3x-y\right)}{2}}=\frac{125}{343}$
$\left(\frac{7}{5}\right)^{\frac{\left(3x-y\right)}{2}}=\left(\frac{7}{5}\right)^{-3}$
3x-y = -6
$(\frac{4a}{b})^{6x-y}=(\frac{2a}{b})^{y-6x}$
Therefor, y=6x as the bases are different so the power should be zero for the results to be equal.
3x-y=-6
or, 3x – 6x = -6
or x= 2
y= 6x = 12
x+y = 14
Question 78: The arithmetic mean of all the distinct numbers that can be obtained by rearranging the digits in 1421, including itself, is
a)Â 2222
b)Â 2442
c)Â 2592
d)Â 3333
78) Answer (A)
Solution:
The number of 4-digit numbers possible using 1,1,2, and 4 is $\frac{4!}{2!}=12$
Number of 1’s, 2’s and 4’s in units digits will be in the ratio 2:1:1, i.e. 6 1’s, 3 2’s and 3 4’s.
Sum = 6(1) + 3(2) + 3(4) = 24
Similarly, in tens digit, hundreds digit and thousands digit as well.
Therefore, sum = 24 + 24(10) + 24(100) + 24(1000) = 24(1111)
Mean =Â $\frac{24\left(1111\right)}{12}=2222$
The answer is option A.
Question 79:Â The average of all 3-digit terms in the arithmetic progression 38, 55, 72, …, is
79)Â Answer:Â 548
Solution:
General term = 38 + (n-1)17 = 17n + 21 = 17(n+1) + 4 = 17k + 4
Each term is in the form of 17k + 4
Least 3-digit number in the form of 17k + 4 is at k = 6, i.e. 106
Highest 3-digit number in the form of 17k + 4 is at k = 58, i.e. 990
106, 123, 140,……….., 990
990 = 106 + 17(n-1)
n = 53
Sum =Â $\frac{53}{2}\left(106+990\right)=53\times548$
Average =Â $53\times\frac{548}{53}=548$
