Percentage Questions for CAT

Percentage Questions for CAT have appeared in the CAT Quant section. You can check out the CAT percentage questions based on CAT Previous year papers. In this article, we will look into some important percentage questions for CAT with solutions. These are a good source for practice; If you want to practice these questions, you can download these Percentage Questions for CAT PDF, which is completely Free.

Download Percentage Questions for CAT

Join CAT 2023 Oline Coaching

Question 1: If equal numbers of people are born on each day, find the approximate percentage of the people whose birthday will fall on 29th February. If we are to consider people born in 20th century (1901-2000) and assuming no deaths.

a) 0.374

b) 0.5732

c) 0.0664

d) None of these

1) Answer (D)

View Video Solution

Solution:

Assume one person is born every day. In 100 years, there will be 25 leap years. So 25*1 additional people will be born on these days.

So, total people born will be = $365 \times 100 \times 1 + 25 \times 1$
And people born on 29th february = $25 \times 1$
Hence percentage will be = $\frac{25 \times 1}{365 \times 100 \times 1 + 25 \times 1} \times 100$ = 0.0684

Question 2: Arun’s present age in years is 40% of Barun’s. In another few years, Arun’s age will be half of Barun’s. By what percentage will Barun’s age increase during this period?

2) Answer: 20

View Video Solution

Solution:

Let Arun’s current age be A. Hence, Barun’s current age is 2.5A
Let Arun’s age be half of Barun’s age after X years.
Therefore, 2*(X+A) = 2.5A + X
Or, X = 0.5A
Hence, Barun’s age increased by 0.5A/2.5A = 20%

Question 3: The number of girls appearing for an admission test is twice the number of boys. If 30% of the girls and 45% of the boys get admission, the percentage of candidates who do not get admission is

a) 35

b) 50

c) 60

d) 65

3) Answer (D)

View Video Solution

Solution:

Let the number of girls be 2x and number of boys be x.

Girls getting admission = 0.6x

Boys getting admission = 0.45x

Number of students not getting admission = 3x – 0.6x -0.45x = 1.95x

Percentage = (1.95x/3x) * 100 = 65%

Question 4: In a village, the production of food grains increased by 40% and the per capita production of food grains increased by 27% during a certain period. The percentage by which the population of the village increased during the same period is nearest to

a) 16

b) 13

c) 10

d) 7

4) Answer (C)

View Video Solution

Solution:

Let initial population and production be x,y and final population be z
Final production = 1.4y, final percapita = 1.27 times initial percapita
=> $\frac{1.4y}{z} $ = $ 1.27 \times \frac{y}{x}$
=> $\frac{z}{x} = \frac{1.4}{1.27} \approx 1.10$
Hence the percentage increase in population = 10%

Question 5: A jar contains a mixture of 175 ml water and 700 ml alcohol. Gopal takes out 10% of the mixture and substitutes it by water of the same amount. The process is repeated once again. The percentage of water in the mixture is now

a) 30.3

b) 35.2

c) 25.4

d) 20.5

5) Answer (B)

View Video Solution

Solution:

Final quantity of alcohol in the mixture = $\dfrac{700}{700+175}*(\dfrac{90}{100})^2*[700+175]$ = 567 ml

Therefore, final quantity of water in the mixture = 875 – 567 = 308 ml

Hence, we can say that the percentage of water in the mixture = $\dfrac{308}{875}\times 100$ = 35.2 %

Question 6: The strength of a salt solution is p% if 100 ml of the solution contains p grams of salt. Each of three vessels A, B, C contains 500 ml of salt solution of strengths 10%, 22%, and 32%, respectively. Now, 100 ml of the solution in vessel A is transferred to vessel B. Then, 100 ml of the solution in vessel B is transferred to vessel C. Finally, 100 ml of the solution in vessel C is transferred to vessel A. The strength, in percentage, of the resulting solution in vessel A is

a) 15

b) 13

c) 12

d) 14

6) Answer (D)

View Video Solution

Solution:

Each of three vessels A, B, C contains 500 ml of salt solution of strengths 10%, 22%, and 32%, respectively.

The amount of salt in vessels A, B, C = 50 ml, 110 ml, 160 ml respectively.

The amount of water in vessels A, B, C = 450 ml, 390 ml, 340 ml respectively.

In 100 ml solution in vessel A, there will be 10ml of salt and 90 ml of water

Now, 100 ml of the solution in vessel A is transferred to vessel B. Then, 100 ml of the solution in vessel B is transferred to vessel C. Finally, 100 ml of the solution in vessel C is transferred to vessel A

i.e after the first transfer, the amount of salt in vessels A, B, C = 40, 120, 160 ml respectively.

after the second transfer, the amount of salt in vessels A, B, C =40, 100, 180 ml respectively.

After the third transfer, the amount of salt in vessels A, B, C = 70, 100, 150 respectively.

Each transfer can be captured through the following table.

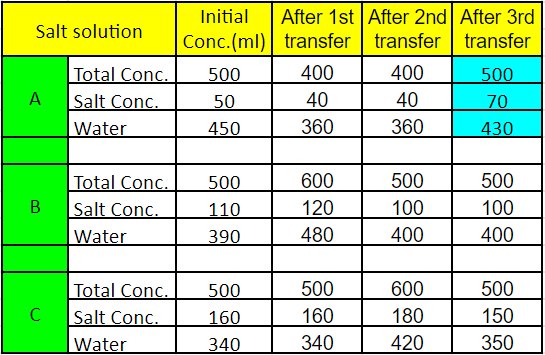
Percentage of salt in vessel A =$\ \frac{\ 70}{500}\times\ 100$

=14%

Question 7: The salaries of Ramesh, Ganesh and Rajesh were in the ratio 6:5:7 in 2010, and in the ratio 3:4:3 in 2015. If Ramesh’s salary increased by 25% during 2010-2015, then the percentage increase in Rajesh’s salary during this period is closest to

a) 10

b) 7

c) 9

d) 8

7) Answer (B)

View Video Solution

Solution:

Let the salaries of Ramesh, Ganesh and Rajesh in 2010 be 6x, 5x, 7x respectively

Let the salaries of Ramesh, Ganesh and Rajesh in 2015 be 3y, 4y, 3y respectively

It is given that Ramesh’s salary increased by 25% during 2010-2015,3y = 1.25*6x

y=2.5x

Percentage increase in Rajesh’s salary = 7.5-7/7=0.07

=7%

Question 8: In a class, 60% of the students are girls and the rest are boys. There are 30 more girls than boys. If 68% of the students, including 30 boys, pass an examination, the percentage of the girls who do not pass is

8) Answer: 20

View Video Solution

Solution:

Assuming the number of students =100x

Hence, the number of girls = 60x and the number of boys = 40x

We have, 60x-40x=30  => x=1.5

The number of girls = 60*1.5=90

Number of girls that pass = 68x-30=68*1.5-30 = 102-30=72

The number of girls who do not pass = 90-72=18

Hence the percentage of girls who do not pass = 1800/90=20

Question 9: A chemist mixes two liquids 1 and 2. One litre of liquid 1 weighs 1 kg and one litre of liquid 2 weighs 800 gm. If half litre of the mixture weighs 480 gm, then the percentage of liquid 1 in the mixture, in terms of volume, is

a) 80

b) 70

c) 85

d) 75

9) Answer (A)

View Video Solution

Solution:

The weight/volume(g/L) for liquid 1 = 1000

The weight/volume(g/L) for liquid 2 = 800

The weight/volume(g/L) of the mixture = 480/(1/2) = 960

Using alligation the ratio of liquid 1 and liquid 2 in the mixture = (960-800)/(1000-960) = 160/40 = 4:1

Hence the percentage of liquid 1 in the mixture = 4*100/(4+1)=80

Question 10: Two alcohol solutions, A and B, are mixed in the proportion 1:3 by volume. The volume of the mixture is then doubled by adding solution A such that the resulting mixture has 72% alcohol. If solution A has 60% alcohol, then the percentage of alcohol in solution B is

a) 90%

b) 94%

c) 92%

d) 89%

10) Answer (C)

View Video Solution

Solution:

Initially let’s consider A and B as one component

The volume of the mixture is doubled by adding A(60% alcohol) i.e they are mixed in 1:1 ratio and the resultant mixture has 72% alcohol.

Let the percentage of alcohol in component 1 be ‘x’.

Using allegations , $\frac{\left(72-60\right)}{x-72}=\frac{1}{1}$ => x= 84

Percentage of alcohol in A = 60% => Let’s percentage of alcohol in B = x%

The resultant mixture has 84% alcohol. ratio = 1:3

Using allegations , $\frac{\left(x-84\right)}{84-60}=\frac{1}{3}$

=> x= 92%

Download CAT 2023 Study Plan PDF